1 4 Cup Plus 1 4 Cup Equals
News Co
Apr 07, 2025 · 5 min read
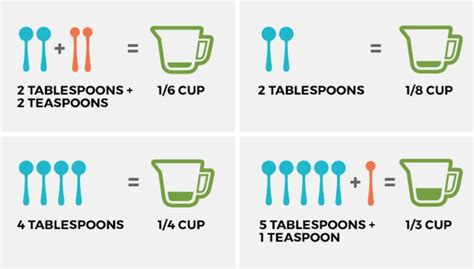
Table of Contents
1/4 Cup Plus 1/4 Cup Equals: A Deep Dive into Fractions and Measurement
Understanding fractions is fundamental to various aspects of life, from cooking and baking to construction and engineering. This seemingly simple question, "1/4 cup plus 1/4 cup equals?", opens a door to exploring fractional arithmetic, its practical applications, and the importance of precise measurement. Let's delve into this seemingly simple problem and uncover its broader implications.
Understanding Fractions: The Building Blocks of Measurement
Before tackling the problem directly, let's review the basics of fractions. A fraction represents a part of a whole. It's composed of two parts:
- Numerator: The top number, indicating how many parts we have.
- Denominator: The bottom number, indicating the total number of equal parts the whole is divided into.
In the case of 1/4 cup, the numerator (1) represents one part, and the denominator (4) indicates that the whole cup is divided into four equal parts.
Adding Fractions: A Step-by-Step Guide
Adding fractions with the same denominator is straightforward. We simply add the numerators while keeping the denominator the same. Therefore, to solve 1/4 cup plus 1/4 cup:
1/4 + 1/4 = (1 + 1) / 4 = 2/4
Simplifying Fractions: Reducing to the Lowest Terms
The fraction 2/4 represents two out of four equal parts. However, this fraction can be simplified. Simplification involves finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it. The GCD of 2 and 4 is 2. Therefore:
2/4 = (2 ÷ 2) / (4 ÷ 2) = 1/2
The Answer: 1/4 Cup Plus 1/4 Cup Equals 1/2 Cup
Therefore, 1/4 cup plus 1/4 cup equals 1/2 cup. This seemingly simple calculation has profound implications in various fields.
Real-World Applications: Where Precision Matters
The accurate addition of fractions is crucial in numerous everyday situations and professional contexts:
1. Cooking and Baking: The Art of Precise Measurement
In cooking and baking, precise measurements are paramount to achieving desired results. Recipes often require fractional amounts of ingredients. Incorrectly adding fractions can lead to inconsistencies in taste, texture, and overall quality of the final product. Understanding how to add fractions ensures that you consistently follow the recipe, leading to better results. Imagine baking a cake; a slight deviation in the amount of baking powder can drastically alter the cake's texture.
2. Construction and Engineering: Building a Solid Foundation
In construction and engineering, precise measurements are critical for structural integrity and safety. Calculations involving fractions are used extensively in designing and building structures, ensuring accuracy in dimensions and material quantities. Errors in fractional calculations can have severe consequences, potentially leading to structural failures or safety hazards. For example, a slight miscalculation in the dimensions of a beam could compromise the entire structure's stability.
3. Medicine and Pharmacy: Ensuring Accurate Dosage
In medicine and pharmacy, precise measurements are vital for patient safety. Accurate dosage calculations involving fractions are essential to ensure the effectiveness and safety of medications. Errors in calculating fractional dosages can lead to adverse effects or treatment failure. This highlights the critical importance of mastering fractional arithmetic in healthcare.
4. Finance and Economics: Managing Resources Effectively
In finance and economics, precise calculations involving fractions are used in various contexts, including budgeting, investment analysis, and risk management. Accurately calculating fractions is crucial for making informed financial decisions and managing resources efficiently. For instance, understanding fractional shares of stock is essential for investors.
5. Science and Research: Analyzing Data Accurately
In scientific research, data analysis often involves fractional calculations. Accurate handling of fractions is crucial for obtaining reliable results and drawing valid conclusions from experiments and observations. Incorrect calculations can lead to flawed interpretations and inaccurate scientific findings.
Beyond the Basics: Exploring More Complex Fractional Calculations
While adding simple fractions like 1/4 + 1/4 is relatively straightforward, more complex scenarios arise frequently. Let's explore some examples:
Adding Fractions with Different Denominators
Adding fractions with different denominators requires finding a common denominator—a number that is a multiple of both denominators. For example, adding 1/3 and 1/4:
- Find the least common multiple (LCM) of the denominators: The LCM of 3 and 4 is 12.
- Convert the fractions to equivalent fractions with the common denominator: 1/3 = (1 × 4) / (3 × 4) = 4/12 1/4 = (1 × 3) / (4 × 3) = 3/12
- Add the numerators: 4/12 + 3/12 = 7/12
Therefore, 1/3 + 1/4 = 7/12.
Subtracting Fractions
Subtracting fractions follows a similar principle to addition. If the denominators are the same, subtract the numerators. If the denominators are different, find a common denominator before subtracting.
Multiplying and Dividing Fractions
Multiplying fractions involves multiplying the numerators and denominators separately. Dividing fractions involves inverting the second fraction and then multiplying.
Mastering Fractions: Tips and Techniques for Success
Mastering fractions requires consistent practice and a solid understanding of the underlying concepts. Here are some helpful tips:
- Visual aids: Use visual aids like diagrams or fraction circles to represent fractions and aid in understanding.
- Practice regularly: Consistent practice is key to mastering any mathematical concept, including fractions.
- Break down complex problems: Break down complex problems into smaller, more manageable steps.
- Use online resources: Numerous online resources, including interactive tutorials and practice exercises, are available to assist in learning fractions.
- Seek help when needed: Don't hesitate to seek help from teachers, tutors, or online communities when struggling with fractions.
Conclusion: The Importance of Precision in a Fractionated World
The simple question, "1/4 cup plus 1/4 cup equals?", has led us on a journey through the world of fractions, highlighting their importance in various aspects of life. From cooking to construction, medicine to finance, accurate fractional calculations are essential for achieving precise results, ensuring safety, and making informed decisions. Mastering fractions is not merely an academic exercise; it's a crucial life skill with far-reaching implications. By understanding the principles of fractional arithmetic and applying them consistently, we can navigate a world where precision is paramount. Remember, a simple 1/2 cup can make all the difference!
Latest Posts
Related Post
Thank you for visiting our website which covers about 1 4 Cup Plus 1 4 Cup Equals . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.