1/6 Divided By 4 As A Fraction
News Co
May 08, 2025 · 4 min read
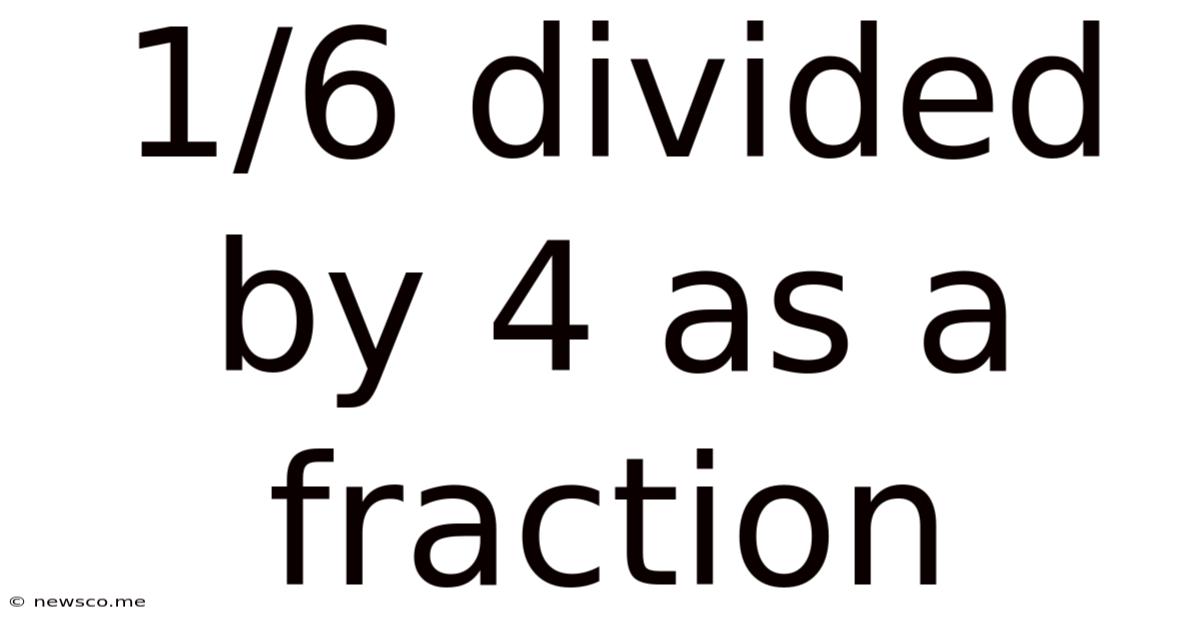
Table of Contents
1/6 Divided by 4: A Comprehensive Guide to Fraction Division
Dividing fractions can seem daunting, but with a clear understanding of the process, it becomes straightforward. This article will guide you through the steps of dividing the fraction 1/6 by 4, providing a detailed explanation and exploring various related concepts. We'll cover not only the calculation itself but also the underlying principles and practical applications of fraction division.
Understanding Fraction Division
Before diving into the specific problem, let's refresh our understanding of fraction division. The core concept revolves around reciprocals. The reciprocal of a fraction is simply the fraction flipped upside down. For instance, the reciprocal of 2/3 is 3/2.
To divide one fraction by another, we follow these steps:
- Find the reciprocal of the second fraction (the divisor).
- Multiply the first fraction by the reciprocal of the second fraction.
- Simplify the resulting fraction (if possible).
Solving 1/6 Divided by 4
Now, let's tackle the problem at hand: 1/6 divided by 4. Remember that we can express the whole number 4 as a fraction: 4/1. Therefore, our problem becomes:
(1/6) ÷ (4/1)
Following the steps outlined above:
-
Find the reciprocal of the second fraction (4/1): The reciprocal of 4/1 is 1/4.
-
Multiply the first fraction (1/6) by the reciprocal of the second fraction (1/4):
(1/6) x (1/4) = (1 x 1) / (6 x 4) = 1/24
-
Simplify the resulting fraction: The fraction 1/24 is already in its simplest form, as 1 and 24 share no common factors other than 1.
Therefore, 1/6 divided by 4 is equal to 1/24.
Visualizing Fraction Division
Visualizing the process can help solidify understanding. Imagine you have a pizza cut into 6 equal slices. You have 1 slice (1/6 of the pizza). Now, you want to divide that single slice among 4 people. Each person would receive 1/24 of the original pizza.
Connecting to Real-World Applications
Fraction division isn't just a mathematical exercise; it's a practical skill with various real-world applications. Here are a few examples:
-
Cooking: If a recipe calls for 1/6 of a cup of flour and you want to make only 1/4 of the recipe, you'll need to calculate (1/6) ÷ 4 = 1/24 of a cup of flour.
-
Construction: Dividing lengths of materials accurately is crucial in construction. If you need to divide a piece of wood that is 1/6 of a meter long into 4 equal parts, each part will be 1/24 of a meter long.
-
Sharing Resources: Imagine you have 1/6 of a bag of candy and you want to share it equally among 4 friends. Each friend receives 1/24 of the bag of candy.
These examples demonstrate that understanding fraction division is essential for tackling everyday problems involving proportions and sharing.
Further Exploring Fraction Division
Let's explore some related concepts to strengthen your understanding of fraction division:
Dividing Fractions by Whole Numbers
As seen in our example, dividing a fraction by a whole number is a specific case of fraction division. The whole number is simply expressed as a fraction with a denominator of 1.
Dividing Fractions by Fractions
The same principles apply when dividing a fraction by another fraction. For instance, to solve (2/3) ÷ (1/2), you would find the reciprocal of (1/2), which is (2/1), and then multiply: (2/3) x (2/1) = 4/3.
Complex Fractions
Complex fractions involve fractions within fractions. These can be simplified by applying the principles of fraction division. For example:
(1/2) / (1/4) = (1/2) x (4/1) = 4/2 = 2
Improper Fractions and Mixed Numbers
The same principles apply when dealing with improper fractions (where the numerator is larger than the denominator) or mixed numbers (a combination of a whole number and a fraction). Always convert mixed numbers to improper fractions before performing division.
Practicing Fraction Division
Practice is key to mastering any mathematical concept. Try working through these problems:
- 1/8 divided by 2
- 2/5 divided by 3
- 3/4 divided by 1/2
- 1 1/2 divided by 1/3
Remember to follow the steps outlined above: find the reciprocal of the second fraction and then multiply. Always simplify the final answer.
Mastering Fraction Division: A Summary
Dividing fractions, while appearing complex at first glance, becomes manageable with consistent practice and a clear understanding of the principles involved. By understanding reciprocals and applying the steps of fraction division, you can confidently tackle a wide range of problems, both simple and complex. The real-world applications of this skill are numerous, highlighting its practical importance beyond the realm of theoretical mathematics. Remember to practice regularly to solidify your understanding and build confidence in your ability to solve fraction division problems effectively. The more you practice, the easier it will become! You'll soon be able to solve even the most challenging fraction division problems with ease and accuracy.
Latest Posts
Latest Posts
-
3 Divided By 8 In Fraction
May 09, 2025
-
Range Of Stem And Leaf Plot
May 09, 2025
-
Can A Given Point Be In Two Lines
May 09, 2025
-
How To Get Rid Of Fraction Exponents
May 09, 2025
-
5 Futov 4 Dyuyma V Sm
May 09, 2025
Related Post
Thank you for visiting our website which covers about 1/6 Divided By 4 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.