10 Out Of 60 As A Percentage
News Co
May 08, 2025 · 5 min read
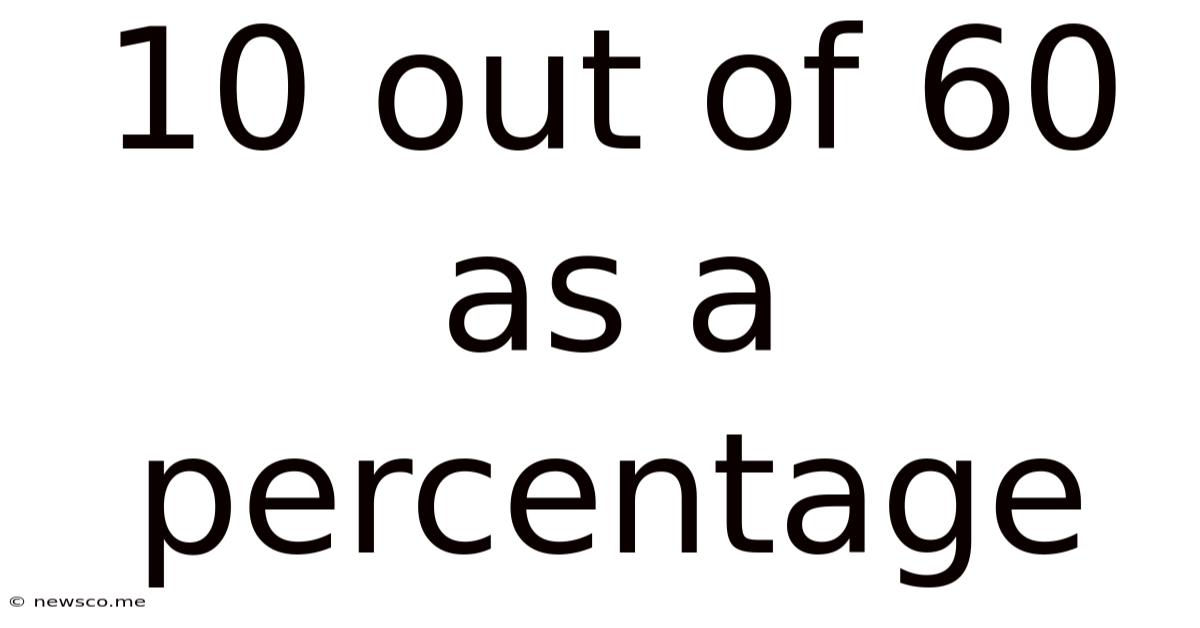
Table of Contents
10 Out of 60 as a Percentage: A Comprehensive Guide
Converting fractions to percentages is a fundamental skill in mathematics with widespread applications in various fields, from calculating grades and discounts to understanding statistical data and financial reports. This comprehensive guide will delve into the calculation of 10 out of 60 as a percentage, explaining the process step-by-step and exploring various related concepts to build a strong understanding of percentage calculations. We will also look at practical applications and related percentage problems to solidify your understanding.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" is derived from the Latin "per centum," meaning "out of a hundred." Therefore, a percentage represents a portion or proportion of a whole, where the whole is always considered to be 100%.
For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Calculating 10 Out of 60 as a Percentage
To calculate 10 out of 60 as a percentage, we follow these simple steps:
Step 1: Express the values as a fraction:
The phrase "10 out of 60" can be written as the fraction 10/60.
Step 2: Simplify the fraction (if possible):
We can simplify the fraction by dividing both the numerator (10) and the denominator (60) by their greatest common divisor (GCD), which is 10.
10 ÷ 10 = 1 60 ÷ 10 = 6
This simplifies the fraction to 1/6.
Step 3: Convert the fraction to a decimal:
To convert the fraction 1/6 to a decimal, we divide the numerator (1) by the denominator (6):
1 ÷ 6 ≈ 0.1667
Step 4: Convert the decimal to a percentage:
To convert the decimal 0.1667 to a percentage, we multiply it by 100 and add the "%" symbol:
0.1667 × 100 = 16.67%
Therefore, 10 out of 60 is equal to 16.67%.
Alternative Calculation Method: Direct Percentage Calculation
Instead of simplifying the fraction first, we can directly calculate the percentage using the following formula:
(Part / Whole) × 100%
In our case:
(10 / 60) × 100% = 0.1667 × 100% = 16.67%
Both methods yield the same result. Choosing the method that feels most comfortable and efficient for you is key.
Practical Applications of Percentage Calculations
Understanding percentage calculations is crucial in various real-world scenarios. Here are a few examples:
1. Academic Performance:
Imagine a student scoring 10 out of 60 on a test. Their percentage score, 16.67%, provides a clear representation of their performance relative to the total possible marks.
2. Sales and Discounts:
Stores often advertise discounts as percentages. For instance, a "20% off" sale means you pay 80% of the original price. Understanding percentages allows you to calculate the actual price reduction.
3. Financial Calculations:
Interest rates on loans, savings accounts, and investments are expressed as percentages. These percentages are crucial in determining the total amount to be repaid or the interest earned.
4. Data Analysis and Statistics:
Percentages are widely used in representing statistical data. For instance, survey results, market share analysis, and population demographics are often expressed using percentages.
5. Budgeting and Finance Management:
Tracking expenses and income using percentages helps in visualizing the proportion of funds allocated to different categories, aiding in better financial planning and decision-making.
Related Percentage Problems and Solutions
Let's explore a few more percentage problems that relate to the concept of calculating 10 out of 60:
Problem 1: If a student scores 40 out of 60 on a test, what is their percentage score?
Solution:
(40 / 60) × 100% = 66.67%
Problem 2: A store offers a 15% discount on an item originally priced at $60. What is the discounted price?
Solution:
Discount amount = 15% of $60 = (15/100) × $60 = $9
Discounted price = Original price – Discount amount = $60 – $9 = $51
Problem 3: If a company's sales increased from 60 units to 70 units, what is the percentage increase?
Solution:
Increase in sales = 70 – 60 = 10 units
Percentage increase = (Increase in sales / Original sales) × 100% = (10 / 60) × 100% = 16.67%
Problem 4: A survey reveals that 10 out of 60 respondents prefer a particular brand. What percentage of respondents prefer that brand?
Solution:
(10 / 60) × 100% = 16.67%
Advanced Concepts and Further Exploration
While the calculation of 10 out of 60 as a percentage is relatively straightforward, a deeper understanding of percentages involves exploring concepts like:
- Percentage Change: Calculating the percentage increase or decrease between two values.
- Percentage Points: The difference between two percentages.
- Compound Interest: Calculating interest earned on both the principal amount and accumulated interest.
- Percentage Yield: A measure of the return on an investment.
- Statistical Significance: Determining the likelihood that observed results are not due to chance.
Mastering these advanced concepts will enhance your ability to analyze and interpret data effectively, whether in academic studies, financial management, or any other field that utilizes percentage calculations.
Conclusion: Mastering Percentages for Success
Understanding percentages is a crucial skill applicable across numerous domains. This guide has thoroughly explained the calculation of 10 out of 60 as a percentage (16.67%), detailing the step-by-step process and providing several real-world examples to illustrate its significance. By grasping the fundamental concepts and exploring the related percentage problems, you can confidently tackle various percentage-related challenges and enhance your analytical and problem-solving abilities. Remember, consistent practice and application are key to mastering this essential mathematical concept.
Latest Posts
Related Post
Thank you for visiting our website which covers about 10 Out Of 60 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.