10 Over 15 As A Percentage
News Co
Mar 26, 2025 · 4 min read
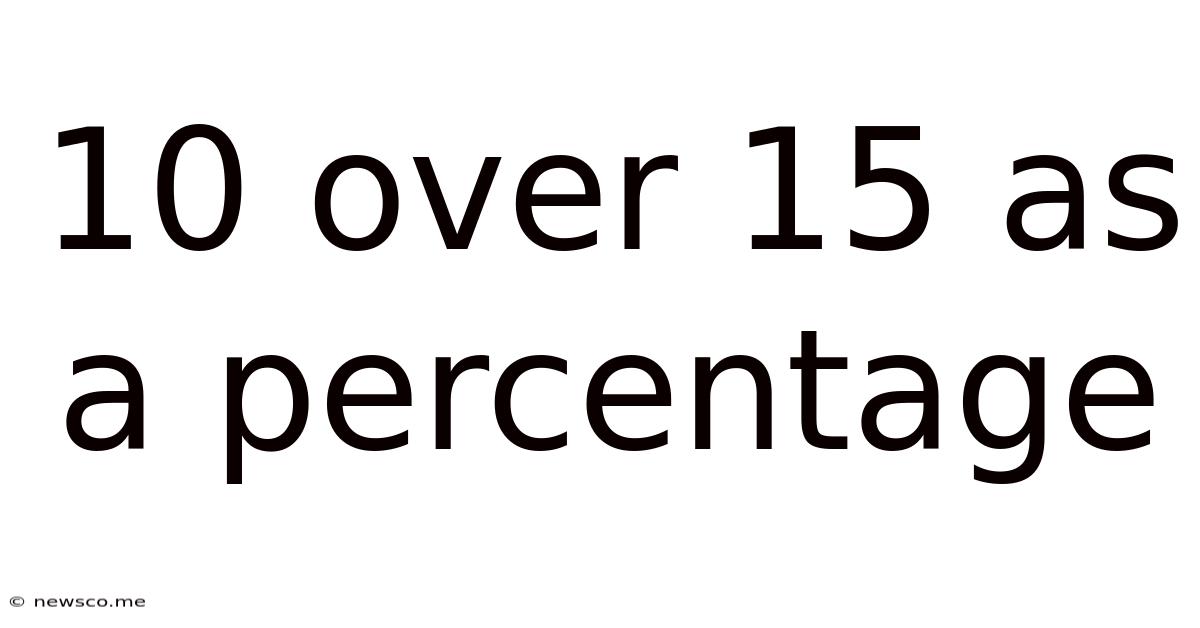
Table of Contents
10 Out of 15 as a Percentage: A Comprehensive Guide
Converting fractions to percentages is a fundamental skill in mathematics with widespread applications in various fields, from calculating grades and discounts to analyzing statistical data and understanding financial reports. This comprehensive guide will delve into the process of converting the fraction 10/15 into a percentage, exploring different methods and providing a detailed explanation to ensure a thorough understanding. We'll also look at practical examples and explore related concepts to solidify your knowledge.
Understanding Fractions and Percentages
Before we dive into the conversion, let's briefly review the concepts of fractions and percentages.
Fractions represent a part of a whole. They consist of a numerator (the top number) and a denominator (the bottom number). In our case, the fraction is 10/15, where 10 represents the part and 15 represents the whole.
Percentages, denoted by the symbol %, represent a fraction of 100. They provide a standardized way to express proportions, making comparisons easier. For example, 50% means 50 out of 100, or 50/100.
Method 1: Simplifying the Fraction
The first and often easiest method involves simplifying the fraction before converting to a percentage. Simplifying a fraction means reducing it to its lowest terms by dividing both the numerator and the denominator by their greatest common divisor (GCD).
Finding the GCD of 10 and 15
The GCD of 10 and 15 is 5. This means we can divide both numbers by 5 without changing the fraction's value.
Simplifying the Fraction
10 ÷ 5 = 2 15 ÷ 5 = 3
Therefore, 10/15 simplifies to 2/3.
Converting the Simplified Fraction to a Percentage
To convert a fraction to a percentage, we multiply the fraction by 100%:
(2/3) * 100% = 66.666...%
This can be rounded to 66.67% for practical purposes.
Method 2: Direct Conversion
This method involves directly converting the fraction 10/15 to a percentage without simplifying it first.
Converting the Fraction to a Decimal
Divide the numerator (10) by the denominator (15):
10 ÷ 15 = 0.6666...
Converting the Decimal to a Percentage
Multiply the decimal by 100%:
0.6666... * 100% = 66.666...%
Again, this can be rounded to 66.67%.
Method 3: Using Proportions
This method uses the concept of proportions to solve the problem. We can set up a proportion to find the percentage:
10/15 = x/100
Where 'x' represents the percentage we want to find.
Solving the Proportion
To solve for x, we cross-multiply:
15x = 1000
x = 1000/15
x = 66.666...
Therefore, 10/15 is equal to 66.67%.
Practical Applications of Percentage Calculations
Understanding how to calculate percentages is crucial in many real-world scenarios. Here are a few examples:
Grade Calculation
Imagine a student scored 10 out of 15 points on a quiz. Using the methods described above, we've determined that this equates to 66.67%. This allows for easy comparison of performance across different assessments.
Discount Calculation
A store offers a 10/15 discount on an item originally priced at $30. This represents a 66.67% discount. The discounted price would be calculated as follows:
Discount amount = $30 * 0.6667 = $20
Discounted price = $30 - $20 = $10
Survey Results
If 10 out of 15 respondents to a survey answered "yes" to a particular question, this means 66.67% of respondents answered "yes." This allows for quick analysis of survey results and identification of trends.
Financial Analysis
Percentage calculations are vital in financial analysis. They are used to calculate interest rates, growth rates, profit margins, and various other financial ratios. Understanding these calculations is crucial for making informed financial decisions.
Understanding the Concept of Recurring Decimals
Notice that in the calculations above, we encountered a recurring decimal: 0.6666... This is represented as 0.6̅ or 2/3. Recurring decimals are decimals that repeat infinitely. When converting fractions to percentages, rounding is often necessary for practical applications, but understanding the recurring nature of certain fractions is important for precise calculations.
Advanced Concepts Related to Percentages
While the conversion of 10/15 to a percentage is straightforward, exploring related concepts can broaden your understanding:
- Percentage Increase/Decrease: This involves calculating the percentage change between two values. For example, if a price increases from $10 to $15, the percentage increase is 50%.
- Percentage Points: These are used to express the difference between two percentages. For example, if the interest rate increases from 5% to 10%, the increase is 5 percentage points.
- Compounding Percentages: This concept is essential in finance and involves calculating interest or growth over multiple periods, taking into account the accumulated interest or growth from previous periods.
Conclusion: Mastering Percentage Conversions
Converting fractions like 10/15 to percentages is a fundamental skill with far-reaching applications. Understanding the different methods, from simplifying fractions to using proportions, empowers you to tackle various percentage-related problems effectively. Remember to practice these methods to build your confidence and proficiency in handling percentage calculations in diverse contexts. The ability to convert fractions to percentages and work with percentages effectively is a valuable asset in numerous academic, professional, and everyday situations. By mastering this skill, you'll enhance your analytical abilities and confidently navigate situations requiring percentage calculations.
Latest Posts
Latest Posts
-
Find The Point On The Y Axis Which Is Equidistant From
May 09, 2025
-
Is 3 4 Bigger Than 7 8
May 09, 2025
-
Which Of These Is Not A Prime Number
May 09, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 09, 2025
-
Are Alternate Exterior Angles Always Congruent
May 09, 2025
Related Post
Thank you for visiting our website which covers about 10 Over 15 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.