11 Divided By 3 As A Fraction
News Co
May 08, 2025 · 5 min read
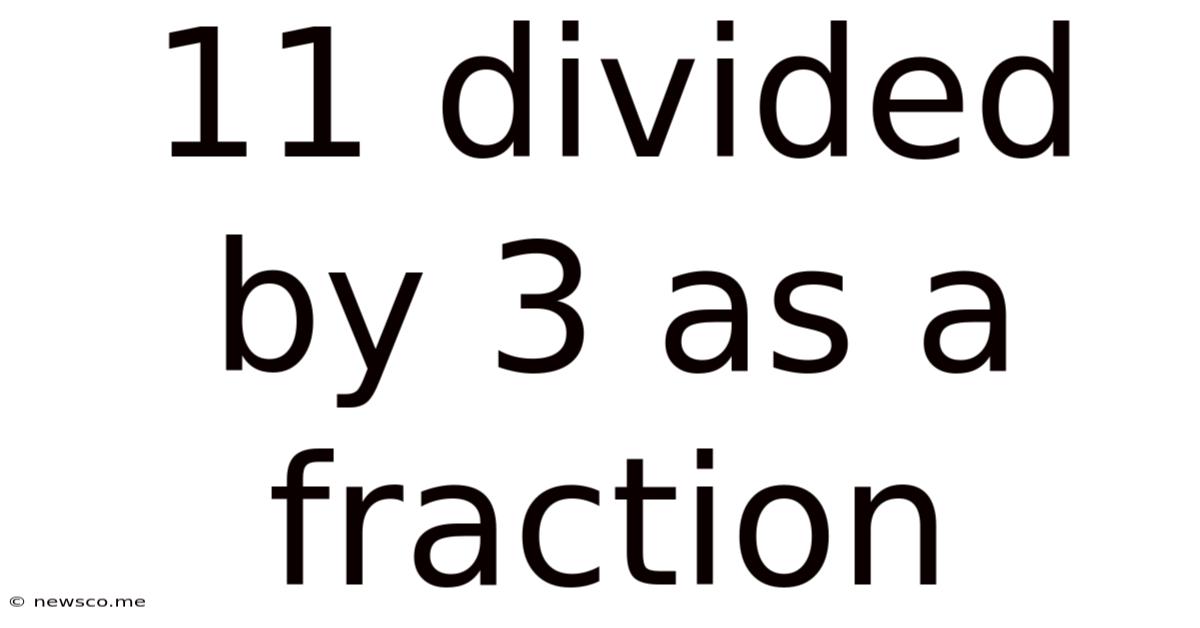
Table of Contents
11 Divided by 3 as a Fraction: A Comprehensive Guide
Dividing 11 by 3 might seem like a simple arithmetic problem, but it opens a door to understanding fractions, mixed numbers, and decimal representations in a deeper way. This comprehensive guide will explore this seemingly straightforward division problem in detail, covering various methods of solution and highlighting the important concepts related to fractions and their applications.
Understanding the Basics: Fractions and Division
Before diving into the specifics of 11 divided by 3, let's solidify our understanding of the fundamental concepts. A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). The denominator indicates how many equal parts the whole is divided into, while the numerator shows how many of those parts we're considering.
Division is essentially the process of finding out how many times one number (the divisor) goes into another number (the dividend). When we divide 11 by 3, we're asking: "How many times does 3 fit into 11?"
Calculating 11 Divided by 3: Step-by-Step
Let's perform the division:
-
Long Division: The traditional method involves long division. Three goes into 11 three times (3 x 3 = 9). This leaves a remainder of 2 (11 - 9 = 2).
-
Expressing as a Mixed Number: The result of the long division can be expressed as a mixed number. A mixed number combines a whole number and a fraction. In this case, 3 (the quotient) becomes the whole number, and the remainder (2) becomes the numerator of the fraction. The denominator remains the same as the original divisor (3). Therefore, 11 divided by 3 is equal to 3 2/3.
-
Improper Fraction: We can also express the result as an improper fraction. An improper fraction has a numerator that is greater than or equal to its denominator. To convert the mixed number to an improper fraction, we multiply the whole number (3) by the denominator (3), add the numerator (2), and place the result over the original denominator: (3 x 3) + 2 = 11. So, the improper fraction is 11/3.
Visualizing 11/3: A Practical Approach
Imagine you have 11 identical objects, such as cookies. If you want to divide these cookies equally among 3 friends, how many cookies does each friend get?
You can give each friend 3 cookies (3 x 3 = 9 cookies total). You'll have 2 cookies left over. To divide these remaining cookies fairly, you'd cut each of the remaining 2 cookies into 3 equal pieces. Each friend would then receive an additional 2/3 of a cookie. Thus, each friend receives a total of 3 2/3 cookies. This visual representation reinforces the mixed number representation of 11/3.
Decimal Representation of 11/3
While fractions are often preferred in mathematical contexts, we can also express 11/3 as a decimal. Performing the long division, we get 3.666... The "6" repeats infinitely, so we represent it using a bar notation: 3.6̅. This is a repeating decimal.
Applications of Fractions: Real-World Examples
Understanding fractions is crucial in various real-world situations:
-
Cooking and Baking: Recipes often require fractional measurements of ingredients (e.g., 2/3 cup of flour).
-
Construction and Engineering: Accurate measurements and calculations are essential in construction, and fractions are frequently used for precise dimensions.
-
Finance: Fractions are used in calculating interest rates, stock prices, and other financial aspects.
-
Data Analysis: Fractions play a vital role in representing proportions and percentages in data analysis and statistics.
Beyond 11/3: Exploring Other Fraction Concepts
The example of 11/3 provides a platform to delve deeper into fraction concepts:
Equivalent Fractions:
Equivalent fractions represent the same value but have different numerators and denominators. For example, 11/3 is equivalent to 22/6, 33/9, and so on. These fractions are all obtained by multiplying both the numerator and denominator by the same number.
Simplifying Fractions:
Simplifying a fraction means reducing it to its lowest terms. This is done by finding the greatest common divisor (GCD) of the numerator and the denominator and dividing both by the GCD. For instance, the fraction 12/18 can be simplified to 2/3 by dividing both the numerator and denominator by their GCD, which is 6.
Adding and Subtracting Fractions:
Adding or subtracting fractions requires a common denominator. If the denominators are the same, simply add or subtract the numerators. If they are different, find the least common multiple (LCM) of the denominators, convert the fractions to equivalent fractions with the LCM as the denominator, and then add or subtract the numerators.
Multiplying and Dividing Fractions:
Multiplying fractions involves multiplying the numerators together and the denominators together. Dividing fractions is equivalent to multiplying the first fraction by the reciprocal of the second fraction (switching the numerator and denominator of the second fraction).
Conclusion: Mastering Fractions for a Stronger Mathematical Foundation
The seemingly simple problem of 11 divided by 3 provides a valuable opportunity to explore the nuances of fractions, mixed numbers, decimals, and their practical applications. Mastering these concepts is fundamental for success in various academic and real-world situations. By understanding the different ways to represent and manipulate fractions, you build a stronger mathematical foundation that extends beyond simple arithmetic problems. The ability to confidently convert between fractions, mixed numbers, and decimals enhances problem-solving skills and provides a deeper appreciation for the intricacies of mathematics. Remember to practice regularly and utilize different approaches to solidify your understanding of these essential mathematical tools.
Latest Posts
Related Post
Thank you for visiting our website which covers about 11 Divided By 3 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.