2 Is What Percent Of 8
News Co
Mar 16, 2025 · 4 min read
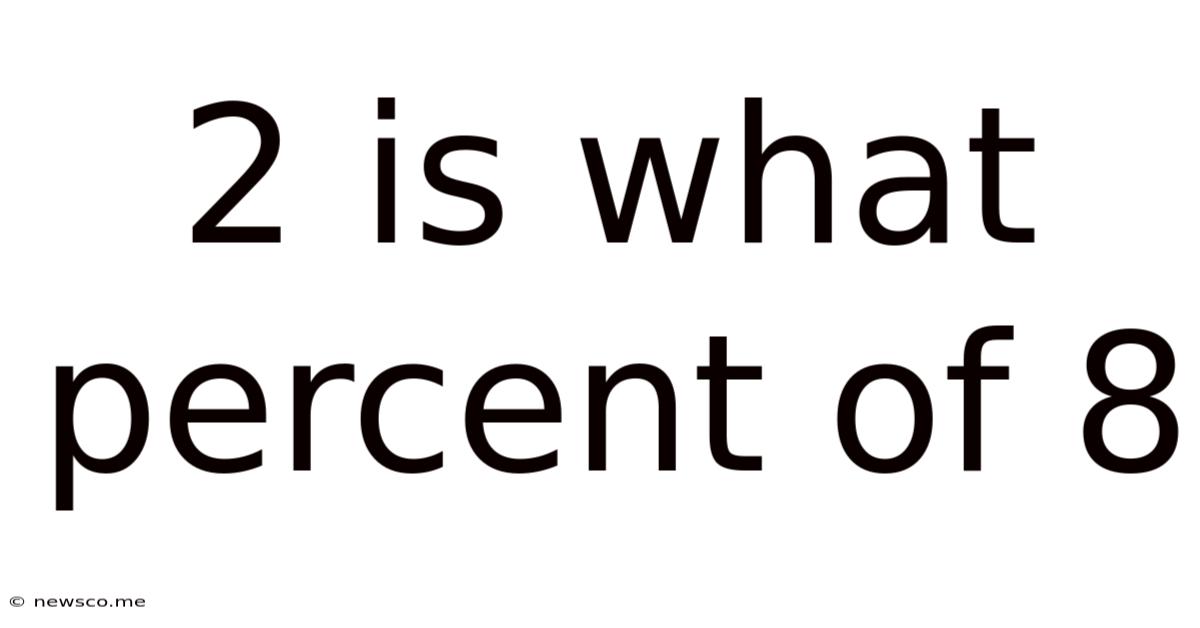
Table of Contents
2 is What Percent of 8? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous fields, from everyday budgeting to complex financial analysis. This article delves deep into the seemingly simple question, "2 is what percent of 8?", providing a comprehensive explanation of the underlying principles and showcasing various methods to solve such problems. We'll explore different approaches, discuss their applicability in various scenarios, and even touch upon advanced percentage calculations.
Understanding Percentages: The Basics
Before diving into the solution, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a number out of 100. The symbol "%" represents "per hundred" or "out of 100". For instance, 50% means 50 out of 100, which simplifies to ½ or 0.5 as a decimal.
The key to solving percentage problems lies in recognizing the relationship between three core components:
- The Part: This is the portion of the whole we are interested in. In our problem, "2" is the part.
- The Whole: This represents the total quantity or amount. In our problem, "8" is the whole.
- The Percentage: This is the fraction of the whole represented as a number out of 100. This is what we need to find.
Method 1: Using the Formula
The most straightforward method to determine what percentage 2 is of 8 involves using a simple formula:
(Part / Whole) x 100% = Percentage
Let's plug in the values from our problem:
(2 / 8) x 100% = Percentage
Simplifying the fraction:
(1/4) x 100% = 25%
Therefore, 2 is 25% of 8.
Method 2: Proportion Method
This method leverages the concept of proportions to solve percentage problems. We set up a proportion where one ratio represents the part to the whole, and the other ratio represents the percentage to 100:
Part / Whole = Percentage / 100
Substituting our values:
2 / 8 = x / 100
To solve for 'x' (the percentage), we cross-multiply:
2 * 100 = 8 * x
200 = 8x
Divide both sides by 8:
x = 200 / 8
x = 25
Therefore, 2 is 25% of 8.
Method 3: Decimal Conversion
This method involves converting the fraction into a decimal and then multiplying by 100 to express it as a percentage.
First, we express the part as a fraction of the whole:
2 / 8 = 0.25
Next, we convert the decimal to a percentage by multiplying by 100:
0.25 x 100% = 25%
Again, we find that 2 is 25% of 8.
Choosing the Right Method
While all three methods produce the same correct answer, the best approach depends on individual preference and the complexity of the problem. The formula method is generally preferred for its directness and ease of understanding. The proportion method is helpful for visualizing the relationship between the parts and the whole. The decimal conversion method is efficient for simple calculations.
Real-World Applications of Percentage Calculations
Understanding percentage calculations isn't limited to academic exercises. It's a crucial skill in many real-world situations:
- Finance: Calculating interest rates, discounts, taxes, profit margins, and investment returns all heavily rely on percentage calculations.
- Retail: Determining sale prices, calculating markups, and understanding profit percentages are essential for retailers.
- Science: Expressing experimental data, analyzing statistical results, and interpreting scientific findings often involve percentages.
- Everyday Life: Calculating tips, determining the percentage of ingredients in recipes, and understanding survey results all necessitate percentage calculations.
Beyond the Basics: More Complex Percentage Problems
While "2 is what percent of 8?" is a relatively simple problem, the principles discussed here extend to more complex scenarios. Let's explore a few examples:
Example 1: Finding the Whole
If 30% of a number is 15, what is the number?
We can set up a proportion:
30/100 = 15/x
Cross-multiplying and solving for x:
30x = 1500
x = 50
Therefore, the number is 50.
Example 2: Finding the Part
What is 15% of 60?
We can use the formula:
(15/100) x 60 = 9
Therefore, 15% of 60 is 9.
Example 3: Percentage Increase/Decrease
A product's price increased from $20 to $25. What is the percentage increase?
First, calculate the difference: $25 - $20 = $5
Then, divide the difference by the original price and multiply by 100:
($5 / $20) x 100% = 25%
Therefore, the price increased by 25%.
Mastering Percentages: Tips and Tricks
Here are some tips to improve your percentage calculation skills:
- Practice regularly: The more you practice, the more confident and efficient you'll become.
- Understand the concepts: Don't just memorize formulas; understand the underlying logic.
- Use different methods: Experiment with different approaches to find the method that best suits your learning style.
- Check your work: Always double-check your calculations to ensure accuracy.
- Utilize online resources: Many online tools and calculators can assist with percentage calculations.
Conclusion: The Power of Percentages
Understanding percentages is a versatile skill with far-reaching applications. From everyday tasks to complex financial calculations, mastering percentage calculations empowers you to analyze data effectively, make informed decisions, and navigate various aspects of life with greater confidence. The seemingly simple question, "2 is what percent of 8?", serves as a gateway to a world of quantitative understanding, offering a solid foundation for tackling more advanced percentage problems and real-world applications. By consistently practicing and applying the methods discussed in this article, you'll become proficient in percentage calculations and unlock their considerable power.
Latest Posts
Latest Posts
-
Find The Point On The Y Axis Which Is Equidistant From
May 09, 2025
-
Is 3 4 Bigger Than 7 8
May 09, 2025
-
Which Of These Is Not A Prime Number
May 09, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 09, 2025
-
Are Alternate Exterior Angles Always Congruent
May 09, 2025
Related Post
Thank you for visiting our website which covers about 2 Is What Percent Of 8 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.