20 Percent Of What Number Is 40
News Co
May 09, 2025 · 4 min read
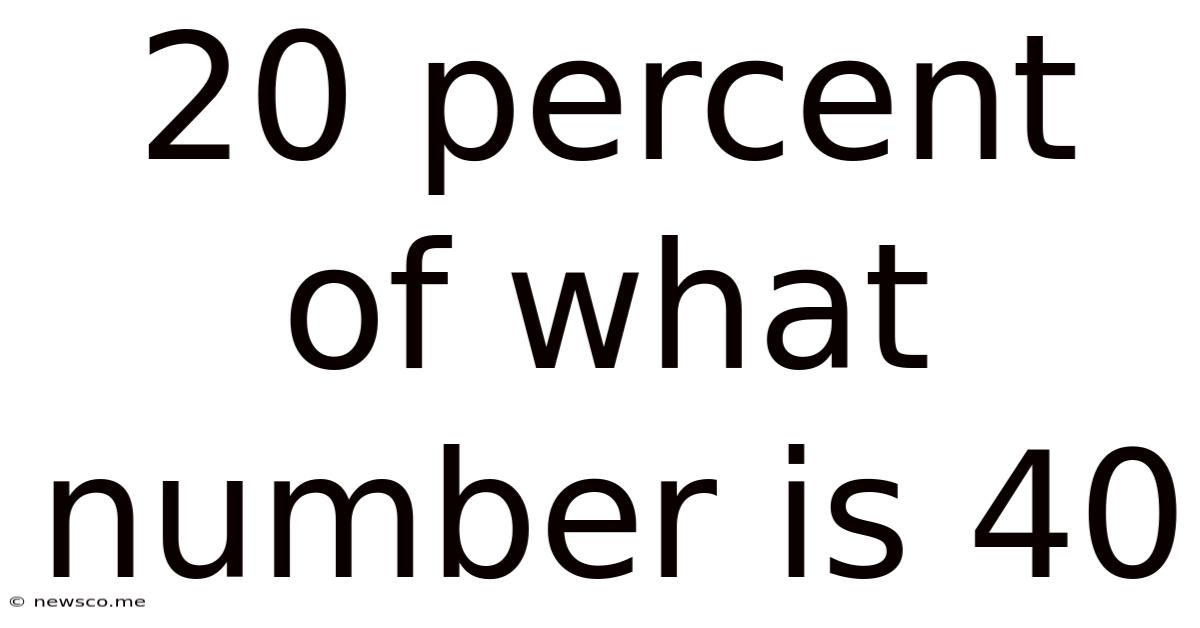
Table of Contents
20% of What Number is 40? A Deep Dive into Percentages and Problem Solving
This seemingly simple question, "20% of what number is 40?", opens the door to a fascinating exploration of percentages, their applications in everyday life, and various methods for solving percentage-based problems. Understanding how to tackle this type of problem is crucial for success in mathematics, finance, and numerous other fields. This article will not only provide the solution but also delve into the underlying concepts and multiple approaches to finding the answer, equipping you with a robust understanding of percentage calculations.
Understanding Percentages: The Foundation
Before diving into the solution, let's solidify our understanding of percentages. A percentage is a way of expressing a number as a fraction of 100. The symbol "%" represents "per cent" or "out of 100." For example, 20% means 20 out of 100, which can be written as the fraction 20/100 or the decimal 0.20. This fundamental concept is the key to solving percentage problems.
Method 1: Using the Equation Approach
The most straightforward method involves setting up an algebraic equation. We can represent the problem as follows:
20% * x = 40
Where 'x' is the unknown number we're trying to find. To solve for 'x', we first convert the percentage to a decimal:
0.20 * x = 40
Now, we isolate 'x' by dividing both sides of the equation by 0.20:
x = 40 / 0.20
x = 200
Therefore, 20% of 200 is 40.
Method 2: The Proportion Method
This method utilizes the concept of proportions. We can set up a proportion relating the percentage to the whole:
20/100 = 40/x
This reads as "20 is to 100 as 40 is to x." To solve for 'x', we cross-multiply:
20 * x = 100 * 40
20x = 4000
Now, divide both sides by 20:
x = 4000 / 20
x = 200
Again, we find that 20% of 200 is 40. This method reinforces the relationship between percentages and fractions.
Method 3: Using the Percentage Formula
A more generalized approach involves using the standard percentage formula:
(Percentage/100) * Whole = Part
In our problem:
- Percentage = 20
- Part = 40
- Whole = x (the unknown)
Substituting these values into the formula:
(20/100) * x = 40
Simplifying and solving for 'x' as we did in Method 1:
0.20 * x = 40
x = 40 / 0.20
x = 200
This method highlights the versatility of the percentage formula and its applicability to various percentage problems.
Real-World Applications: Where Percentages Matter
Understanding percentage calculations is vital in many aspects of daily life and professional settings. Here are some examples:
Finance and Budgeting:
- Calculating discounts: Retail stores frequently offer discounts as percentages. Knowing how to calculate the discounted price is essential for smart shopping. For example, a 20% discount on a $100 item equates to a savings of $20.
- Interest rates: Interest rates on loans, savings accounts, and investments are expressed as percentages. Understanding these percentages allows you to compare different financial options and make informed decisions.
- Tax calculations: Sales tax, income tax, and other taxes are often calculated as percentages of the total amount.
- Investment returns: Investors track their returns on investment as percentages to gauge the success of their portfolios.
Data Analysis and Statistics:
- Representing proportions: Percentages are used extensively in data analysis to represent proportions within a dataset. For instance, a survey might show that 20% of respondents prefer a particular brand.
- Calculating growth rates: Businesses often use percentages to calculate growth rates in sales, profits, or market share.
- Interpreting statistical data: Many statistical analyses present data using percentages to make it easier to understand and interpret.
Everyday Life:
- Tips and gratuities: Calculating a tip at a restaurant often involves determining a percentage of the total bill.
- Sale prices: Understanding percentage discounts is crucial for making informed purchasing decisions.
- Recipe scaling: Adjusting recipe ingredients often involves calculating percentages to scale a recipe up or down.
Beyond the Basics: Advanced Percentage Problems
While the problem "20% of what number is 40?" serves as a fundamental example, percentage problems can become more complex. These might involve:
- Multiple percentages: Calculating a series of percentage increases or decreases.
- Compounding interest: Calculating the accumulated value of an investment over time, considering the effect of compounding interest.
- Percentage change: Determining the percentage increase or decrease between two values.
Mastering Percentages: Tips and Practice
To truly master percentage calculations, consistent practice is key. Here are some tips:
- Practice regularly: Solve various percentage problems to reinforce your understanding.
- Use different methods: Try different approaches to solving problems, such as the equation method, proportion method, and the general percentage formula. This will enhance your problem-solving skills.
- Visualize the problem: Imagine the scenario described in the problem. This can help you grasp the context and apply the correct methods.
- Check your work: Always verify your answers to ensure accuracy.
- Seek help when needed: Don't hesitate to seek assistance from teachers, tutors, or online resources if you encounter difficulties.
In conclusion, the seemingly simple question, "20% of what number is 40?", offers a gateway to understanding the fundamental concepts of percentages and their wide-ranging applications. By mastering various methods for solving percentage problems, you equip yourself with a valuable skillset applicable to numerous aspects of life, from personal finance to professional success. Remember, consistent practice and a thorough understanding of the underlying concepts are the keys to mastering percentages.
Latest Posts
Latest Posts
-
Find The Point On The Y Axis Which Is Equidistant From
May 09, 2025
-
Is 3 4 Bigger Than 7 8
May 09, 2025
-
Which Of These Is Not A Prime Number
May 09, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 09, 2025
-
Are Alternate Exterior Angles Always Congruent
May 09, 2025
Related Post
Thank you for visiting our website which covers about 20 Percent Of What Number Is 40 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.