25 Percent As A Fraction In Simplest Form
News Co
Mar 26, 2025 · 5 min read
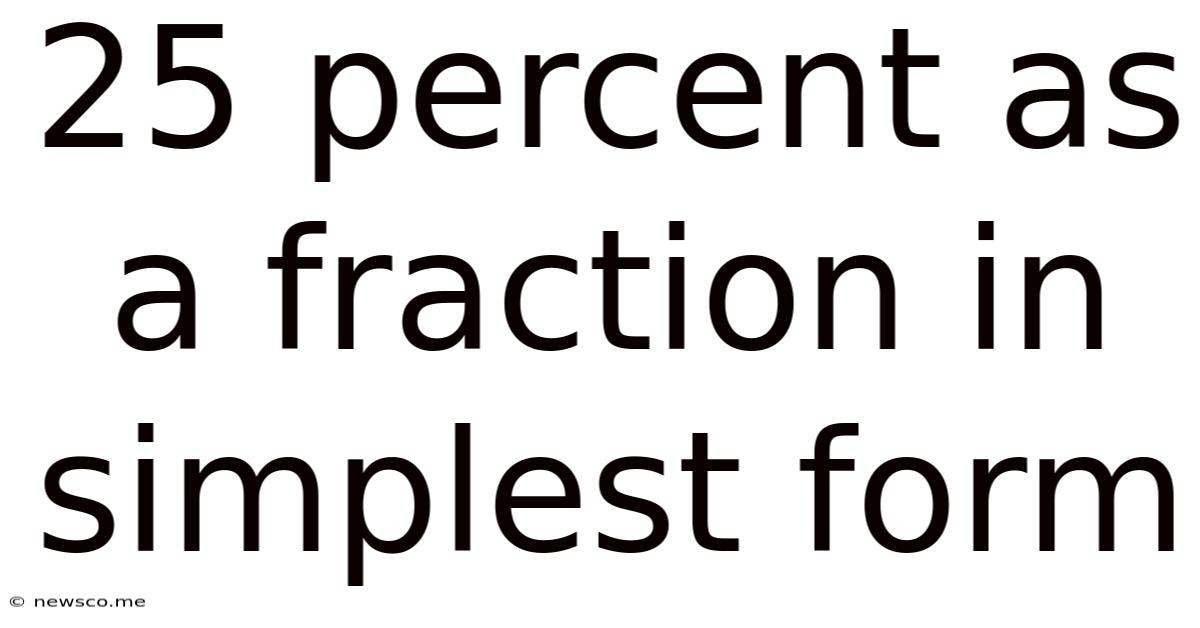
Table of Contents
25 Percent as a Fraction in Simplest Form: A Comprehensive Guide
Understanding percentages, fractions, and decimals is fundamental to various aspects of life, from everyday calculations to complex financial analyses. This comprehensive guide delves into the conversion of percentages to fractions, specifically focusing on expressing 25 percent as a fraction in its simplest form. We'll explore the underlying concepts, step-by-step procedures, and practical applications to solidify your understanding.
Understanding Percentages and Fractions
Before we dive into converting 25 percent, let's review the basics.
What is a Percentage?
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "per hundred" or "out of one hundred." For example, 25% means 25 out of 100.
What is a Fraction?
A fraction represents a part of a whole. It's expressed as a ratio of two numbers, the numerator (top number) and the denominator (bottom number). The numerator indicates the number of parts considered, while the denominator indicates the total number of parts in the whole. For example, 1/4 represents one part out of four equal parts.
Converting 25% to a Fraction
The process of converting a percentage to a fraction involves two simple steps:
-
Write the percentage as a fraction with a denominator of 100. This directly reflects the meaning of "per hundred." For 25%, this translates to 25/100.
-
Simplify the fraction to its lowest terms. This means finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it. The GCD is the largest number that divides both the numerator and the denominator without leaving a remainder.
Let's apply this to 25%:
-
25% as a fraction: 25/100
-
Finding the GCD: The GCD of 25 and 100 is 25.
-
Simplifying the fraction: Dividing both the numerator and denominator by 25 gives us 1/4.
Therefore, 25% expressed as a fraction in its simplest form is 1/4.
Visualizing 25% as a Fraction
Understanding fractions can be easier with visualization. Imagine a pizza cut into four equal slices. If you eat one slice, you've consumed 1/4 of the pizza. This visually represents 25% (one quarter of the whole). This simple example demonstrates the equivalence of 25% and 1/4.
Practical Applications of 25% (1/4)
The fraction 1/4, equivalent to 25%, appears frequently in various real-life scenarios:
-
Sales and Discounts: A 25% discount on a $100 item means a reduction of $25 (1/4 of $100).
-
Measurement Conversions: Understanding fractions is vital for converting units. For example, converting inches to feet involves the fraction 1/12 (1 inch is 1/12 of a foot).
-
Recipe Adjustments: If a recipe calls for a certain quantity, adjusting it by 25% involves simply dividing the quantity by four.
-
Data Analysis: Representing proportions of data sets as fractions simplifies interpretations and allows for easier comparisons.
-
Probability: In probability calculations, 25% represents a one-in-four chance of an event occurring.
-
Finance: Calculating interest rates, determining portions of investments, and understanding financial statements often involve percentages and their fractional equivalents.
Working with Other Percentages and Their Fractional Equivalents
The method used to convert 25% to 1/4 can be applied to other percentages:
-
50%: 50/100 simplifies to 1/2.
-
75%: 75/100 simplifies to 3/4.
-
10%: 10/100 simplifies to 1/10.
-
20%: 20/100 simplifies to 1/5.
-
30%: 30/100 simplifies to 3/10.
To convert any percentage to a fraction, follow these steps:
-
Write the percentage as a fraction over 100.
-
Simplify the fraction to its lowest terms by finding the greatest common divisor (GCD) of the numerator and the denominator and dividing both by the GCD.
This approach ensures that the fraction is expressed in its most concise and manageable form.
Advanced Concepts: Percentages Greater Than 100%
While less common in everyday contexts, percentages can also be greater than 100%. These represent values exceeding the whole. For instance, 150% can be expressed as 150/100, simplifying to 3/2 or 1 1/2. This signifies 1 and a half times the original value.
Understanding Decimal Equivalents
Percentages, fractions, and decimals are interconnected. 25% is equivalent to 1/4, which is also equal to 0.25 (in decimal form). Understanding these conversions facilitates seamless transitions between different mathematical representations.
Solving Problems Involving 25% (1/4)
Let's explore some example problems:
Problem 1: A store offers a 25% discount on a $80 shirt. What is the final price?
Solution:
-
Calculate the discount amount: 25% of $80 = (1/4) * $80 = $20
-
Subtract the discount from the original price: $80 - $20 = $60
The final price is $60.
Problem 2: If 25% of a number is 15, what is the number?
Solution:
-
Represent the problem as an equation: (1/4) * x = 15
-
Solve for x: x = 15 * 4 = 60
The number is 60.
Conclusion
Converting percentages to fractions, particularly understanding 25% as 1/4, is a crucial skill with numerous applications. By mastering the conversion process and understanding the underlying concepts, you enhance your mathematical proficiency and ability to solve problems in various real-world contexts. From everyday shopping to advanced financial calculations, the ability to effortlessly convert between percentages and fractions is an invaluable asset. Remember to practice these conversions regularly to reinforce your understanding and improve your problem-solving skills. The more familiar you become with these relationships, the easier it will become to navigate various numerical challenges.
Latest Posts
Latest Posts
-
Find The Point On The Y Axis Which Is Equidistant From
May 09, 2025
-
Is 3 4 Bigger Than 7 8
May 09, 2025
-
Which Of These Is Not A Prime Number
May 09, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 09, 2025
-
Are Alternate Exterior Angles Always Congruent
May 09, 2025
Related Post
Thank you for visiting our website which covers about 25 Percent As A Fraction In Simplest Form . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.