3 1/2 As A Whole Number
News Co
May 08, 2025 · 6 min read
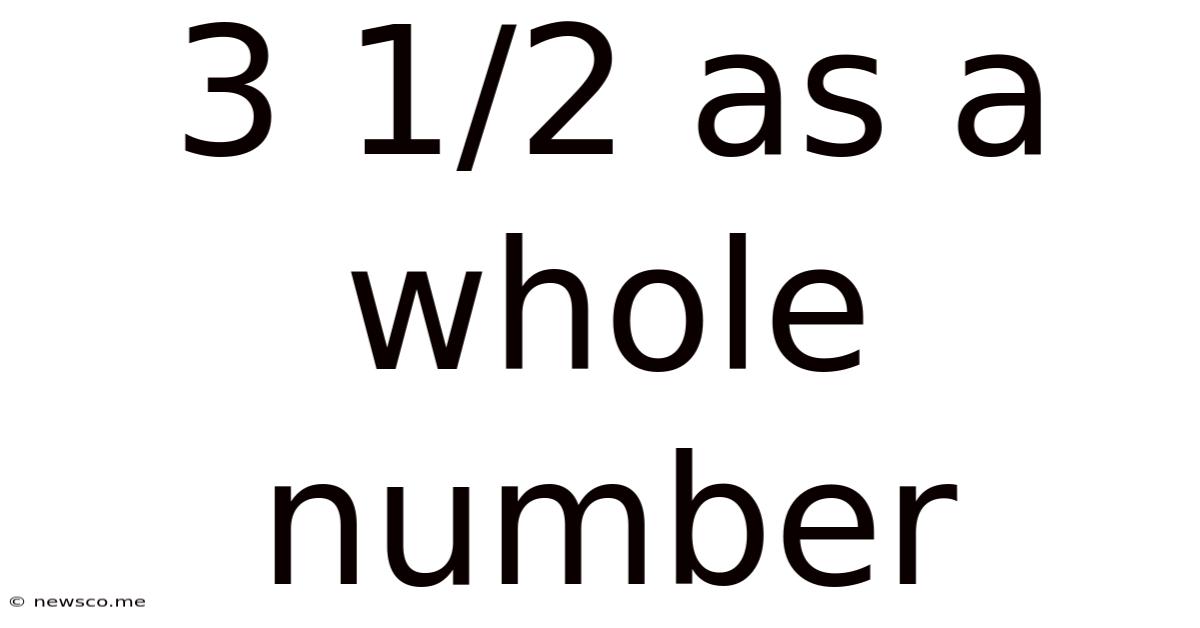
Table of Contents
3 1/2 as a Whole Number: Understanding Mixed Numbers and Their Applications
The question of how to represent 3 1/2 as a whole number is deceptively simple, yet it unveils fundamental concepts in mathematics, particularly in understanding fractions and their relationship to whole numbers. While there's no single whole number that perfectly equates to 3 1/2, we can explore several ways to address this, depending on the context and desired level of precision. This article delves deep into the topic, exploring various approaches, practical applications, and the broader mathematical concepts involved.
Understanding Mixed Numbers and Improper Fractions
Before we tackle the main question, let's solidify our understanding of mixed numbers and improper fractions. A mixed number, like 3 1/2, combines a whole number and a proper fraction. A proper fraction has a numerator (top number) smaller than its denominator (bottom number). An improper fraction, on the other hand, has a numerator equal to or greater than its denominator.
Converting between mixed numbers and improper fractions is a crucial skill. To convert a mixed number to an improper fraction, follow these steps:
- Multiply the whole number by the denominator: 3 * 2 = 6
- Add the numerator to the result: 6 + 1 = 7
- Keep the same denominator: The improper fraction equivalent of 3 1/2 is 7/2.
Conversely, to convert an improper fraction to a mixed number, you:
- Divide the numerator by the denominator: 7 ÷ 2 = 3 with a remainder of 1
- The quotient becomes the whole number: 3
- The remainder becomes the numerator of the fraction: 1
- The denominator remains the same: 2
- The mixed number is 3 1/2.
This conversion process is fundamental to understanding how to work with numbers that aren't purely whole.
Approximating 3 1/2 as a Whole Number
Since 3 1/2 isn't a whole number, we need to consider approximation techniques. The most straightforward approach is rounding. Rounding involves choosing the closest whole number. In this case:
- Rounding down: This results in 3. This is useful when precision isn't crucial and a lower bound is desired.
- Rounding up: This gives us 4. This is appropriate when a higher bound or a safety margin is needed.
The choice between rounding up or down often depends on the context. Imagine you need to buy chairs for a party. If you expect 3 1/2 people, rounding up to 4 ensures everyone has a seat. If you're measuring ingredients for a recipe, rounding down might be acceptable, depending on the recipe's tolerance for slight variations.
Representing 3 1/2 in Different Contexts
The best way to represent 3 1/2 as a whole number heavily depends on the situation. Let's look at a few examples:
Context 1: Counting Discrete Objects
If you're counting discrete objects, like apples, you can't have half an apple. In this context, you would round to the nearest whole number:
- Rounding down (3 apples): You have 3 complete apples, disregarding the half.
- Rounding up (4 apples): You acknowledge the half-apple and include it by rounding up. You might consider this if you want to make sure you have enough.
Context 2: Measurement
Imagine measuring a length. If you measure 3 1/2 meters of fabric, you wouldn't represent this as a whole number unless you are approximating for a rough estimate. The exact measurement of 3 1/2 meters is retained as it is. However, if this is for a simple calculation where precision isn't important, you might round.
Context 3: Data Analysis and Statistics
In statistical analysis, you might encounter 3 1/2 as an average value. Depending on the context and the level of precision needed, you might round up or down, or you might even keep it as a decimal (3.5). The impact of rounding should be carefully considered in the interpretation of the data.
Context 4: Financial Transactions
In financial transactions, precision is paramount. You cannot represent $3.50 as a whole number without losing the 50 cents. This illustrates how different contexts demand different levels of precision.
Decimal Representation: A Bridge Between Fractions and Whole Numbers
Another way to approach the problem is by converting 3 1/2 into its decimal equivalent: 3.5. While this isn't a whole number, it offers a more precise representation than simply rounding up or down. Decimal representation helps bridge the gap between fractions and whole numbers, providing greater accuracy in many situations.
Utilizing the Concept of Averaging
In certain scenarios, understanding how 3 1/2 is calculated can be crucial. Imagine you're calculating the average score of two tests: One test resulted in a score of 3 and the other in a score of 4. The average is calculated as (3 + 4) / 2 = 3.5, or 3 1/2.
In this context, 3 1/2 represents a mean, an average value indicating that the scores are centered around this figure. While 3 1/2 isn't a whole number score achievable on a single test, it provides a meaningful summary of the data.
Advanced Applications: Using 3 1/2 in Equations and Formulas
The handling of 3 1/2 depends heavily on its context within a larger mathematical problem. Let's imagine a few scenarios:
- Linear Equations: If 3 1/2 appears as a coefficient in a linear equation, it remains as 3.5 or 7/2 throughout the calculations. Simplifying into a whole number would lead to inaccuracy.
- Geometric Problems: In geometrical calculations involving areas or volumes, 3 1/2 would be treated as a precise numerical value, requiring careful manipulation without rounding.
- Algebraic Expressions: If 3 1/2 is part of an algebraic expression, you treat it as a constant, performing the operations according to the rules of algebra, again without prematurely rounding.
Conclusion: The Context Dictates the Representation
The question of how to represent 3 1/2 as a whole number doesn't have a single, definitive answer. The appropriate approach is heavily dependent on the context. Rounding, while a convenient method, might sacrifice precision. Using the decimal representation (3.5) often provides better accuracy, but still isn't a whole number. Understanding the underlying mathematical concepts, the relationships between fractions and whole numbers, and the specific requirements of a particular problem, is key to making an informed decision on the best way to handle this mixed number. Remember that maintaining accuracy and making appropriate choices regarding precision are pivotal aspects of mathematical problem-solving. The appropriate method is always chosen to ensure the final answer is accurate and meaningful in the context of its application.
Latest Posts
Latest Posts
-
8 1 4 As A Fraction
May 09, 2025
-
How To Find A1 In Arithmetic Sequence
May 09, 2025
-
Subtracting A Positive Integer From A Negative Integer
May 09, 2025
-
The Diagonals Of A Parallelogram Are Equal
May 09, 2025
-
Angle Pairs Worksheet Geometry Answer Key
May 09, 2025
Related Post
Thank you for visiting our website which covers about 3 1/2 As A Whole Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.