3/4 Has How Many 1/8 In It
News Co
May 08, 2025 · 4 min read
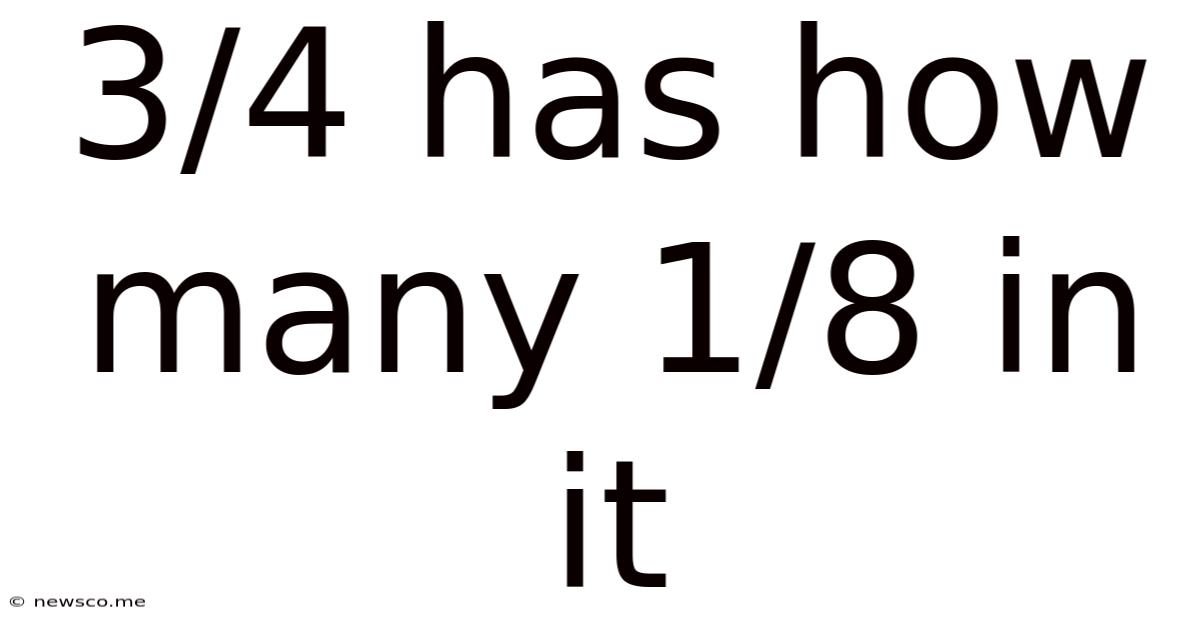
Table of Contents
3/4 Has How Many 1/8 in It? A Comprehensive Guide to Fraction Division
Understanding fractions is a fundamental skill in mathematics, essential for various applications in daily life and advanced studies. This comprehensive guide will delve into the question, "3/4 has how many 1/8 in it?", providing a step-by-step explanation, exploring different methods of solving fraction division problems, and offering practical examples to solidify your understanding.
Understanding Fractions: A Quick Recap
Before we tackle the central question, let's refresh our understanding of fractions. A fraction represents a part of a whole. It consists of two main parts:
- Numerator: The top number, indicating the number of parts we have.
- Denominator: The bottom number, indicating the total number of equal parts the whole is divided into.
For example, in the fraction 3/4, the numerator is 3, and the denominator is 4. This means we have 3 out of 4 equal parts of a whole.
Method 1: Converting to a Common Denominator
One straightforward approach to determining how many 1/8 are in 3/4 is to convert both fractions to have a common denominator. This allows for a direct comparison.
Finding the Least Common Denominator (LCD)
The least common denominator (LCD) is the smallest multiple that both denominators (4 and 8) share. In this case, the LCD is 8.
Converting the Fractions
To convert 3/4 to a fraction with a denominator of 8, we multiply both the numerator and the denominator by 2:
(3/4) * (2/2) = 6/8
Now we can compare 6/8 and 1/8 directly.
Determining the Number of 1/8s
Since we have 6/8, and each 1/8 is a unit, we simply have six 1/8s in 3/4.
Method 2: Using Fraction Division
Another method involves directly dividing the two fractions. Dividing fractions is accomplished by multiplying the first fraction by the reciprocal of the second fraction. The reciprocal of a fraction is obtained by swapping the numerator and denominator.
Finding the Reciprocal
The reciprocal of 1/8 is 8/1, or simply 8.
Performing the Division
To find out how many 1/8s are in 3/4, we perform the following calculation:
(3/4) ÷ (1/8) = (3/4) * (8/1) = (3 * 8) / (4 * 1) = 24/4 = 6
Therefore, there are six 1/8s in 3/4.
Method 3: Visual Representation
A visual approach can help solidify understanding, especially for beginners. Imagine a pie chart or a rectangular shape representing a whole.
Dividing the Whole
Divide the whole into four equal parts. Shade three of these parts to represent 3/4.
Subdividing the Parts
Now, divide each of the four parts into two smaller equal parts. This results in a total of eight equal parts, and each part represents 1/8.
Counting the 1/8s
Observe that the three previously shaded parts now encompass six of the smaller 1/8 parts. This visually confirms that there are six 1/8s in 3/4.
Expanding the Concept: Working with More Complex Fractions
The principles discussed above can be extended to more complex fraction division problems. Let's explore some examples:
Example 1: How many 2/5 are in 4/10?
First, convert 4/10 to its simplest form: 2/5. Therefore, there is one 2/5 in 4/10.
Example 2: How many 3/8 are in 9/16?
We can use the division method: (9/16) ÷ (3/8) = (9/16) * (8/3) = (9 * 8) / (16 * 3) = 72/48 = 3/2 = 1 ½. There are one and a half 3/8 in 9/16.
Practical Applications of Fraction Division
Understanding fraction division is crucial in various real-world scenarios:
- Cooking and Baking: Adjusting recipes, scaling ingredients up or down.
- Construction and Engineering: Measuring and cutting materials accurately.
- Finance: Calculating proportions, percentages, and interest.
- Data Analysis: Working with data sets and interpreting results.
Troubleshooting Common Mistakes
Several common pitfalls can arise when working with fractions:
- Incorrectly finding the reciprocal: Remember to swap the numerator and denominator when finding the reciprocal.
- Improper cancellation: Ensure you cancel common factors only from the numerator and denominator of the same fraction.
- Incorrect simplification: Always simplify fractions to their lowest terms.
Conclusion: Mastering Fraction Division
Understanding how to determine how many times one fraction goes into another is a critical skill. This guide has presented three methods – conversion to a common denominator, direct fraction division, and visual representation – to solve problems like "3/4 has how many 1/8 in it?". Mastering these techniques will build a solid foundation for more advanced mathematical concepts and problem-solving in various real-world situations. By practicing regularly and understanding the underlying principles, you can confidently tackle any fraction division problem you encounter. Remember to check your work and simplify your answer to its lowest terms for the most accurate and efficient solution.
Latest Posts
Related Post
Thank you for visiting our website which covers about 3/4 Has How Many 1/8 In It . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.