38 Is 19 Of What Number
News Co
May 07, 2025 · 4 min read
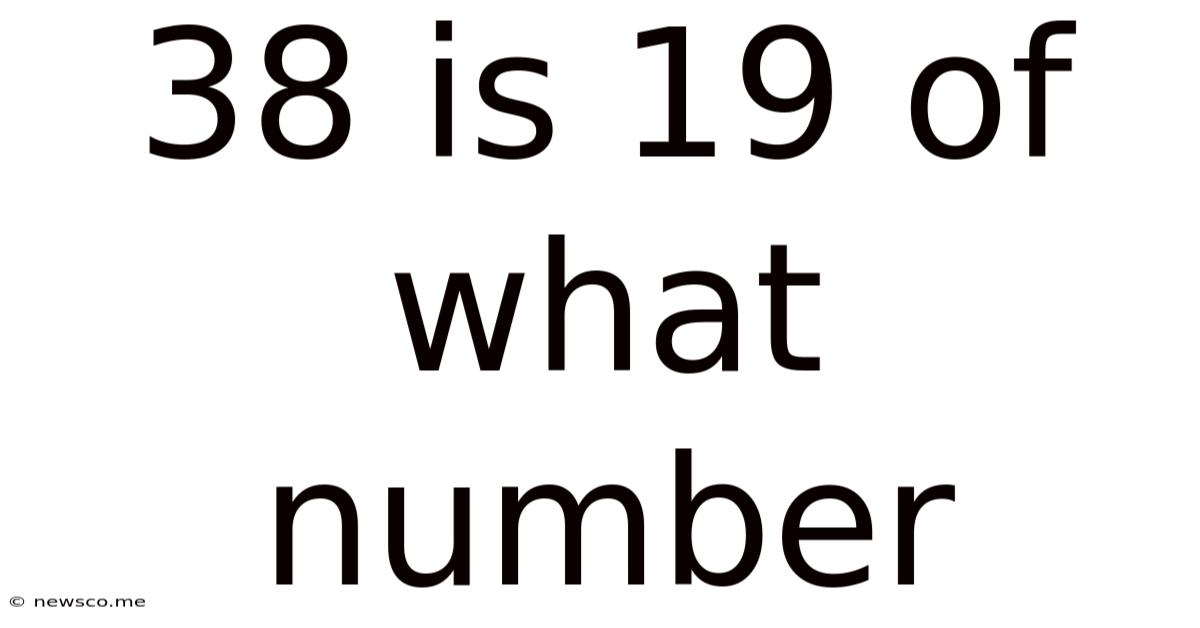
Table of Contents
38 is 19% of What Number? A Deep Dive into Percentage Calculations
This seemingly simple question, "38 is 19% of what number?", opens the door to a fascinating exploration of percentage calculations, their applications in various fields, and the underlying mathematical principles. While the answer itself is straightforward, understanding the how and why behind the solution is crucial for anyone looking to improve their mathematical literacy and problem-solving skills. This article will not only provide the solution but also delve into the various methods of solving percentage problems, exploring their practical implications and offering further examples to solidify your understanding.
Understanding Percentages: The Foundation
Before tackling the specific problem, let's establish a solid understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per cent," meaning "out of 100." For example, 50% means 50 out of 100, which can be written as the fraction 50/100 or the decimal 0.5.
Percentages are ubiquitous in everyday life. We encounter them in sales discounts (e.g., "20% off"), interest rates (e.g., "5% annual interest"), statistics (e.g., "75% of respondents agreed"), and countless other situations. Mastering percentage calculations is therefore essential for navigating these scenarios effectively.
Method 1: Using the Percentage Formula
The most common approach to solving percentage problems is using the fundamental percentage formula:
Part = Percent × Whole
In our case, we know the "Part" (38) and the "Percent" (19%). We need to find the "Whole," which we'll represent with the variable 'x'. Substituting these values into the formula gives us:
38 = 0.19 × x
To solve for 'x', we need to isolate it by dividing both sides of the equation by 0.19:
x = 38 / 0.19
Calculating this gives us:
x = 200
Therefore, 38 is 19% of 200.
Method 2: Using Proportions
Another effective method involves setting up a proportion. A proportion is a statement that two ratios are equal. We can represent our problem as the following proportion:
19/100 = 38/x
This proportion states that the ratio of 19 to 100 (19%) is equal to the ratio of 38 to the unknown number (x). To solve for x, we can cross-multiply:
19x = 3800
Then, divide both sides by 19:
x = 3800 / 19
x = 200
Again, we arrive at the same answer: 38 is 19% of 200.
Method 3: Working with Decimals
This method directly converts the percentage to a decimal and sets up an equation. 19% is equivalent to 0.19 (by dividing 19 by 100). The problem can be expressed as:
0.19x = 38
Dividing both sides by 0.19:
x = 38 / 0.19
x = 200
This method offers a concise and direct way to solve the problem.
Practical Applications of Percentage Calculations
Understanding percentage calculations is vital in numerous real-world scenarios. Consider these examples:
-
Finance: Calculating interest earned on savings accounts, understanding loan repayments, analyzing investment returns, and determining tax rates all involve percentage calculations.
-
Business: Analyzing sales data, calculating profit margins, determining discounts, and evaluating market share require proficiency in percentage calculations.
-
Science: Expressing experimental results, analyzing statistical data, and representing proportions in scientific studies often utilize percentages.
-
Everyday Life: Calculating tips at restaurants, understanding sales taxes, comparing prices, and interpreting survey results all involve percentages.
Solving More Complex Percentage Problems
Let's extend our understanding by tackling some more complex scenarios:
Example 1: Finding the Percentage Increase/Decrease:
A product's price increased from $150 to $180. What is the percentage increase?
First, find the difference: $180 - $150 = $30
Then, divide the difference by the original price: $30 / $150 = 0.2
Finally, multiply by 100 to express it as a percentage: 0.2 × 100 = 20%
Therefore, the price increased by 20%.
Example 2: Calculating a Percentage of a Percentage:
A store offers a 20% discount on an item already reduced by 10%. What is the final price if the original price was $500?
First, calculate the 10% discount: $500 × 0.10 = $50. The price becomes $500 - $50 = $450.
Next, calculate the 20% discount on the reduced price: $450 × 0.20 = $90. The final price is $450 - $90 = $360.
Avoiding Common Mistakes in Percentage Calculations
Several common mistakes can lead to incorrect results. Be mindful of these pitfalls:
-
Incorrect order of operations: Always follow the order of operations (PEMDAS/BODMAS) to ensure accurate calculations.
-
Confusing percentages and decimals: Remember to convert percentages to decimals (by dividing by 100) before performing calculations.
-
Incorrectly interpreting the base: Identify the base or original value correctly when calculating percentage increases or decreases.
-
Rounding errors: Avoid premature rounding during calculations to maintain accuracy. Round only at the final step.
Conclusion: Mastering Percentage Calculations for Success
The seemingly simple question of "38 is 19% of what number?" has provided a springboard for a thorough exploration of percentage calculations. We've examined various methods for solving percentage problems, highlighted their practical applications, and explored more complex scenarios. By understanding the underlying principles and avoiding common pitfalls, you can confidently tackle percentage calculations in any context, enhancing your mathematical skills and empowering you to navigate the numerical world with greater ease and accuracy. Remember that consistent practice is key to mastering any mathematical concept, so continue to challenge yourself with diverse problems and gradually expand your understanding. The more you practice, the more intuitive and effortless percentage calculations will become.
Latest Posts
Latest Posts
-
Cubic Parent Function Domain And Range
May 08, 2025
-
Domain And Range Of A Vertical Line
May 08, 2025
-
2 3 E 4 5 I 6 8 Next Value
May 08, 2025
-
What Is The Sum Of Exterior Angles Of A Pentagon
May 08, 2025
-
Calculate The Area Of The Following Figure
May 08, 2025
Related Post
Thank you for visiting our website which covers about 38 Is 19 Of What Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.