40 Percent Of What Number Is 20
News Co
May 08, 2025 · 4 min read
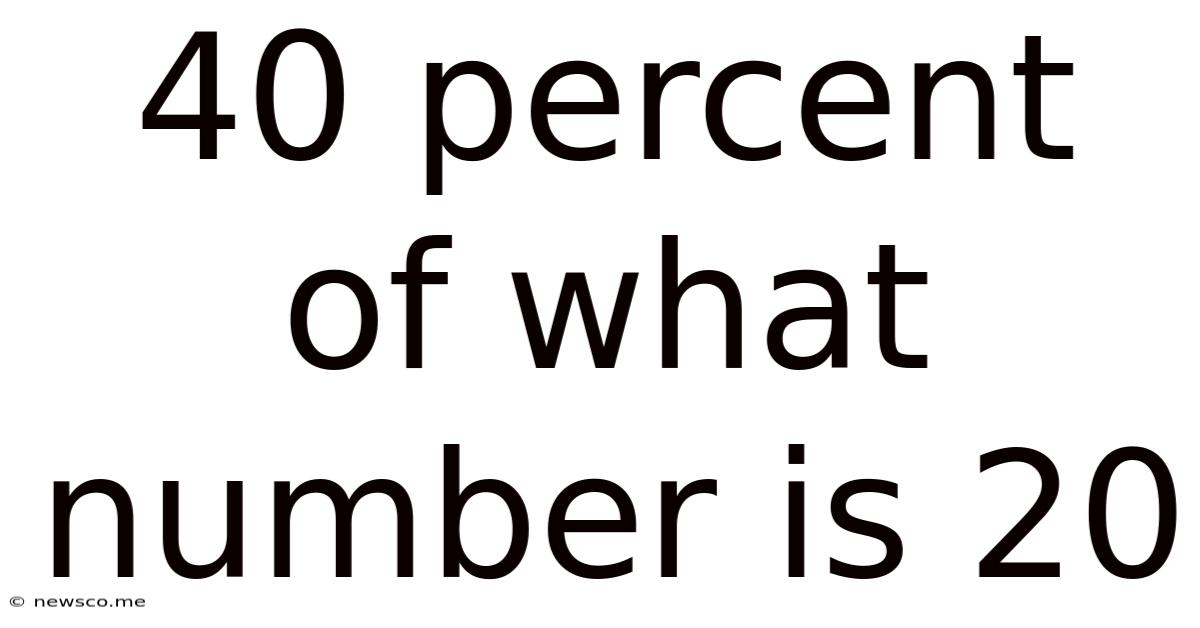
Table of Contents
- 40 Percent Of What Number Is 20
- Table of Contents
- 40% of What Number is 20? Unlocking the World of Percentages
- Understanding Percentages: The Foundation
- Method 1: Using the Equation
- Method 2: Using Proportions
- Method 3: Working with Fractions
- Real-World Applications: Where Percentages Matter
- Expanding Your Percentage Skills: Beyond the Basics
- Mastering Percentages: A Continuous Learning Process
- Latest Posts
- Related Post
40% of What Number is 20? Unlocking the World of Percentages
This seemingly simple question – "40% of what number is 20?" – opens a door to a vast world of mathematical concepts and real-world applications. Understanding how to solve this problem not only helps you with basic percentage calculations but also equips you to tackle more complex scenarios in various fields, from finance and business to science and everyday life. This comprehensive guide will delve into multiple methods of solving this problem, explain the underlying principles, and showcase practical examples to solidify your understanding.
Understanding Percentages: The Foundation
Before diving into the solution, let's establish a solid understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per hundred" or "out of 100." For example, 40% can be written as 40/100 or its simplified fraction, 2/5. This fundamental understanding is crucial for solving percentage problems.
Method 1: Using the Equation
The most straightforward approach involves setting up an algebraic equation. Let's represent the unknown number as 'x'. The problem can be translated into the following equation:
0.40x = 20
Here, 0.40 is the decimal equivalent of 40%. To solve for 'x', we need to isolate it. We can do this by dividing both sides of the equation by 0.40:
x = 20 / 0.40
x = 50
Therefore, 40% of 50 is 20.
Method 2: Using Proportions
Proportions offer another effective way to solve percentage problems. A proportion expresses the equality of two ratios. In this case, we can set up the following proportion:
40/100 = 20/x
This proportion states that the ratio of 40 to 100 is equal to the ratio of 20 to the unknown number 'x'. To solve for 'x', we can cross-multiply:
40x = 20 * 100
40x = 2000
Now, divide both sides by 40:
x = 2000 / 40
x = 50
Again, we find that 40% of 50 is 20.
Method 3: Working with Fractions
As mentioned earlier, 40% can be expressed as the fraction 2/5. We can rewrite the problem as:
(2/5) * x = 20
To solve for 'x', multiply both sides by the reciprocal of 2/5, which is 5/2:
x = 20 * (5/2)
x = 100 / 2
x = 50
This method confirms that 40% of 50 is 20. This approach highlights the interchangeability between percentages, decimals, and fractions.
Real-World Applications: Where Percentages Matter
Understanding percentage calculations is crucial in numerous real-life situations. Here are some examples:
-
Finance: Calculating interest earned on savings accounts, understanding discounts on purchases, determining loan payments, and analyzing investment returns all rely heavily on percentage calculations. For instance, if a bank offers a 3% interest rate on a savings account, you'd use percentage calculations to determine the amount of interest earned on your deposit.
-
Business: Businesses use percentages for profit margins, sales tax calculations, markup pricing, and analyzing market share. A business might use percentage calculations to determine the profit margin on a product after factoring in costs and sales price.
-
Science: Percentages are commonly used in scientific research, data analysis, and presenting results. For instance, researchers might report that 60% of participants in a study showed a particular response.
-
Everyday Life: Calculating tips at restaurants, understanding sales tax, determining discounts during shopping, and comparing prices all involve using percentages. For example, if you want to calculate a 15% tip on a meal, you'll use percentage calculations.
Expanding Your Percentage Skills: Beyond the Basics
While this article focused on solving "40% of what number is 20?", the underlying principles can be applied to solve a wide range of percentage problems. Here are some variations and more advanced scenarios:
-
Finding the percentage: If you know two numbers and need to find the percentage one represents of the other, you can use the formula: (Part / Whole) * 100%
-
Finding the part: If you know the percentage and the whole number, you can calculate the part by multiplying the whole number by the percentage (expressed as a decimal).
-
Finding the whole: This is the type of problem we tackled in this article. You know the part and the percentage, and need to find the whole number.
-
Percentage increase/decrease: These calculations involve finding the difference between two values and expressing it as a percentage of the original value. Percentage increase/decrease is often used to track changes in stock prices, population sizes, or other measurable quantities.
-
Compound interest: This involves calculating interest on both the principal amount and the accumulated interest from previous periods. This is a more advanced application of percentage calculations crucial for understanding long-term investments and loans.
Mastering Percentages: A Continuous Learning Process
Becoming proficient in percentage calculations takes practice. Start with simple problems like the one discussed here and gradually work your way towards more complex scenarios. Using different methods – equations, proportions, fractions – will help reinforce your understanding and allow you to choose the most efficient method based on the problem at hand. Remember, the key is to understand the underlying principles, and with consistent practice, you’ll master the world of percentages with confidence. This knowledge will not only help you solve mathematical problems but will also prove invaluable in navigating various aspects of your personal and professional life.
Latest Posts
Related Post
Thank you for visiting our website which covers about 40 Percent Of What Number Is 20 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.