47 6 As A Mixed Number
News Co
May 08, 2025 · 5 min read
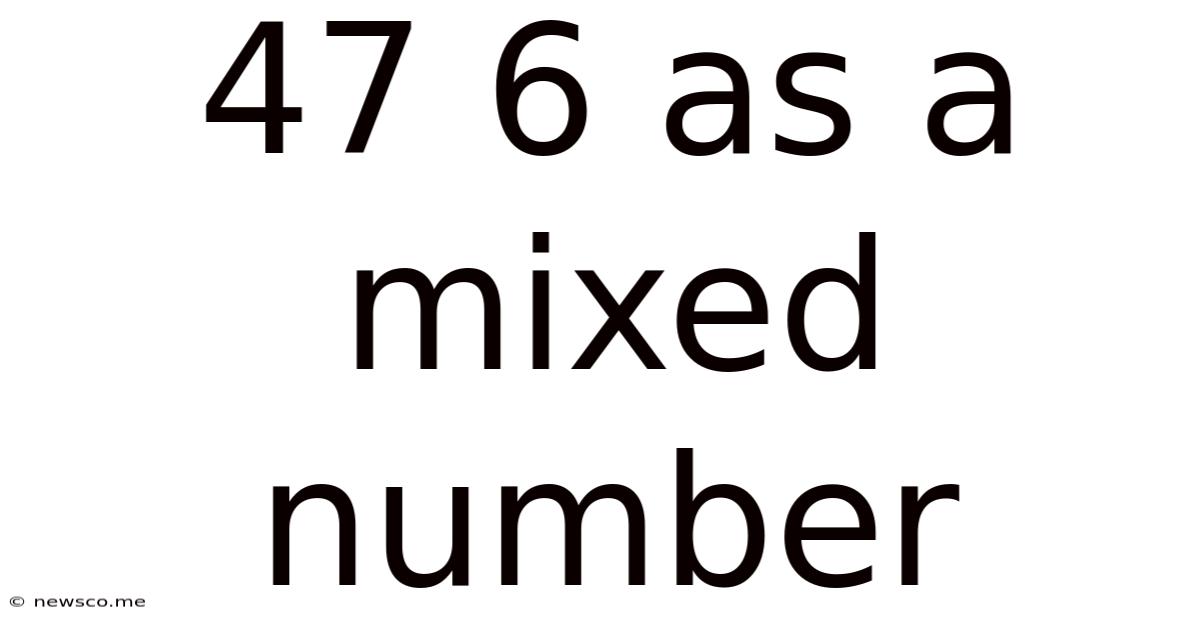
Table of Contents
47/6 as a Mixed Number: A Comprehensive Guide
Understanding fractions and how to convert them into mixed numbers is a fundamental skill in mathematics. This comprehensive guide will delve into the process of converting the improper fraction 47/6 into a mixed number, explaining the concept in detail and providing practical examples. We'll also explore related concepts and applications to solidify your understanding.
Understanding Improper Fractions and Mixed Numbers
Before we dive into converting 47/6, let's clarify the definitions:
-
Improper Fraction: An improper fraction is a fraction where the numerator (the top number) is greater than or equal to the denominator (the bottom number). For example, 47/6 is an improper fraction because 47 is greater than 6.
-
Mixed Number: A mixed number combines a whole number and a proper fraction. A proper fraction is a fraction where the numerator is less than the denominator. For instance, 7 5/6 is a mixed number.
The key to understanding mixed numbers lies in recognizing that the whole number represents how many times the denominator goes into the numerator completely, and the fraction represents the remainder.
Converting 47/6 to a Mixed Number: Step-by-Step
Converting an improper fraction like 47/6 to a mixed number involves dividing the numerator by the denominator. Here's the step-by-step process:
-
Divide the numerator by the denominator: Divide 47 by 6. This gives us a quotient (the result of the division) and a remainder.
47 ÷ 6 = 7 with a remainder of 5
-
The quotient becomes the whole number part of the mixed number: The quotient, 7, becomes the whole number part of our mixed number.
-
The remainder becomes the numerator of the fractional part: The remainder, 5, becomes the numerator of the fraction in our mixed number.
-
The denominator remains the same: The denominator of the original improper fraction, 6, remains the same in the fractional part of the mixed number.
Therefore, 47/6 as a mixed number is 7 5/6.
Visual Representation: Understanding the Concept
Imagine you have 47 cookies, and you want to divide them equally among 6 friends. You can give each friend 7 cookies (7 x 6 = 42 cookies). You'll have 5 cookies left over (47 - 42 = 5). This leftover represents the remaining fraction. So each friend gets 7 whole cookies and 5/6 of a cookie. This perfectly illustrates the mixed number 7 5/6.
Practical Applications of Converting Improper Fractions to Mixed Numbers
Converting improper fractions to mixed numbers is crucial in various mathematical contexts and real-world applications:
-
Measurement: When measuring lengths, weights, or volumes, you often encounter mixed numbers. For example, 7 5/6 inches represents a measurement that's more easily understood than 47/6 inches.
-
Baking and Cooking: Recipes frequently utilize mixed numbers to indicate ingredient quantities, making the instructions clearer and more user-friendly. For instance, a recipe might call for 2 1/2 cups of flour.
-
Time: Expressing time often involves mixed numbers. For example, 1 hour and 45 minutes can be represented as 1 3/4 hours.
-
Geometry and Problem Solving: Many geometric problems and word problems require the conversion of improper fractions to mixed numbers for easier calculation and interpretation of results.
Converting Mixed Numbers back to Improper Fractions
It's equally important to understand the reverse process—converting a mixed number back to an improper fraction. This skill is essential for performing calculations involving mixed numbers. Let's convert 7 5/6 back to an improper fraction:
-
Multiply the whole number by the denominator: Multiply 7 (the whole number) by 6 (the denominator) which equals 42.
-
Add the numerator: Add the result (42) to the numerator (5), which gives you 47.
-
Keep the denominator the same: The denominator remains 6.
Therefore, 7 5/6 as an improper fraction is 47/6. This confirms our initial conversion.
Working with Mixed Numbers: Addition and Subtraction
Adding and subtracting mixed numbers requires careful attention to the fractional parts. Here's a brief overview:
Addition:
To add mixed numbers, add the whole numbers together and then add the fractions. If the sum of the fractions results in an improper fraction, convert it to a mixed number and add it to the sum of the whole numbers.
Subtraction:
Subtracting mixed numbers is similar. Subtract the fractions first. If the numerator of the top fraction is smaller than the bottom fraction, borrow 1 from the whole number, convert it into a fraction with the same denominator, and then subtract.
Working with Mixed Numbers: Multiplication and Division
Multiplication and division of mixed numbers typically involve converting the mixed numbers into improper fractions first. This simplifies the calculations significantly.
Multiplication:
Convert both mixed numbers to improper fractions, multiply the numerators, and then multiply the denominators. Simplify the resulting fraction if possible and convert back to a mixed number if desired.
Division:
Convert both mixed numbers to improper fractions. To divide fractions, invert the second fraction (the divisor) and multiply. Simplify the resulting fraction and convert to a mixed number, if necessary.
Advanced Concepts: Equivalent Fractions and Simplification
Understanding equivalent fractions is essential when working with mixed numbers. Equivalent fractions represent the same value but have different numerators and denominators. For example, 5/10, 10/20, and 1/2 are all equivalent fractions.
Simplification, or reducing fractions to their lowest terms, involves dividing the numerator and denominator by their greatest common divisor (GCD). This makes the fraction easier to work with.
Conclusion: Mastering Mixed Numbers
Converting 47/6 to the mixed number 7 5/6 is a foundational skill in mathematics. Understanding this process, along with the ability to convert back and forth between improper fractions and mixed numbers, is vital for success in various mathematical applications and real-world scenarios. By mastering the concepts outlined in this guide, you'll gain a stronger foundation in fractions and enhance your overall mathematical abilities. Remember to practice regularly to solidify your understanding and build confidence in tackling more complex fraction problems. The ability to comfortably work with mixed numbers will undoubtedly prove valuable in your academic and professional pursuits.
Latest Posts
Latest Posts
-
Find The Prime Factors Of 50
May 08, 2025
-
How Much Is 65 Pounds In Dollars
May 08, 2025
-
What Is The Value Of X In Each Figure
May 08, 2025
-
An Irrational Number Greater Than 10
May 08, 2025
-
What Is 20 Out Of 25 In Percentage
May 08, 2025
Related Post
Thank you for visiting our website which covers about 47 6 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.