8 6 On A Number Line
News Co
May 08, 2025 · 5 min read
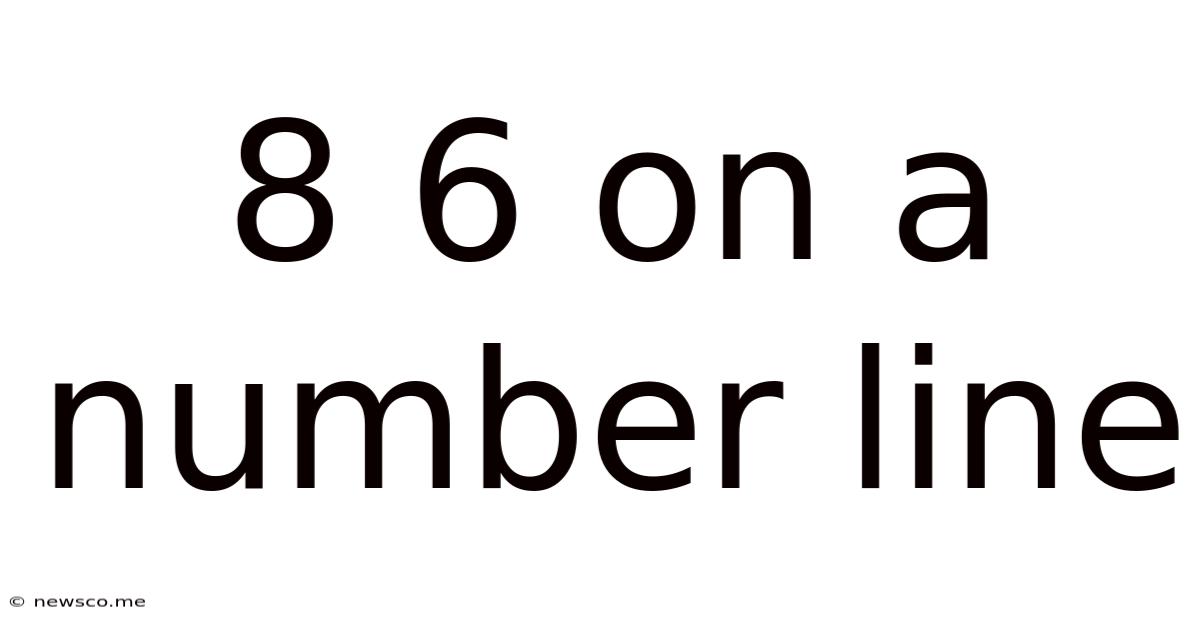
Table of Contents
8 and 6 on a Number Line: A Comprehensive Guide
Understanding number lines is fundamental to grasping mathematical concepts. This comprehensive guide delves into the representation of numbers, specifically 8 and 6, on a number line, exploring various aspects, including their position, distance, and applications. We'll also touch upon more advanced concepts and their relevance to real-world scenarios.
Understanding the Number Line
A number line is a visual representation of numbers as points on a line. It provides a clear and intuitive way to understand the ordering and relationships between numbers. The line extends infinitely in both directions, represented by arrows at each end. A zero point (0) is typically marked as the origin, separating positive numbers (to the right) from negative numbers (to the left).
Key Features of a Number Line:
- Origin (0): The central point, separating positive and negative numbers.
- Positive Numbers: Located to the right of the origin, increasing in value as you move right.
- Negative Numbers: Located to the left of the origin, decreasing in value as you move left.
- Equal Intervals: The distance between consecutive numbers is consistent, ensuring accurate representation of numerical relationships.
Locating 8 and 6 on the Number Line
Locating 8 and 6 on a number line is straightforward. Since both are positive integers, they will be found to the right of the origin.
- Locating 6: Starting at the origin (0), move six units to the right. The point you reach represents the number 6.
- Locating 8: Starting at the origin (0), move eight units to the right. The point you reach represents the number 8.
Notice that 8 is located to the right of 6 on the number line. This visually confirms that 8 is greater than 6 (8 > 6).
Comparing 8 and 6 on the Number Line
The number line provides a visual tool for comparing numbers. The position of a number on the line directly indicates its relative size. Since 8 is to the right of 6, we can immediately see that:
- 8 > 6 (8 is greater than 6)
- 6 < 8 (6 is less than 8)
This simple visual comparison is crucial for understanding inequalities and ordering numbers.
Calculating the Distance Between 8 and 6
The number line can also help us calculate the distance between two numbers. The distance between 8 and 6 is simply the number of units separating them. Counting the units between 6 and 8 on the number line gives us a distance of 2 units.
Alternatively, we can use subtraction: 8 - 6 = 2. This confirms the distance between 8 and 6 is 2 units.
Applications of Number Lines: Real-World Examples
Number lines aren't just abstract mathematical tools; they have practical applications in various real-world scenarios:
1. Temperature Measurement:
Thermometers often utilize a number line to display temperature readings, with positive numbers representing above-zero temperatures and negative numbers representing below-zero temperatures. You can easily compare temperatures using the relative positions on the number line. For instance, 8°C is warmer than 6°C.
2. Measurement of Physical Quantities:
Rulers and measuring tapes are essentially number lines used to measure lengths. The markings represent units (inches, centimeters, etc.), and you can locate specific lengths on the number line.
3. Timelines:
Historians and project managers often use number lines (timelines) to represent events chronologically. The number line's horizontal axis represents time, with events plotted at their corresponding points.
4. Financial Tracking:
In finance, number lines can represent profit/loss, stock prices over time, or budget allocation. Visualizing these using a number line enhances understanding and analysis.
5. Data Representation:
Number lines are helpful in representing simple datasets, especially for comparing values. For example, you can show the number of sales for each month on a number line, visually comparing sales performance across different months.
Extending the Concept: Integers, Decimals, and Fractions
While our initial focus was on integers (8 and 6), the number line's utility extends to other number types:
Integers:
The number line easily accommodates all integers, both positive and negative, extending infinitely in both directions. This allows for comparing and ordering a wide range of integers.
Decimals:
Decimals can also be represented on a number line. The intervals between whole numbers can be further subdivided to accommodate decimal values. For instance, you can locate 6.5 midway between 6 and 7.
Fractions:
Fractions can also be accurately located on a number line. Consider the fraction 6 ½. This would lie exactly midway between 6 and 7 on the number line.
Advanced Applications and Concepts
Beyond basic representation and comparison, number lines contribute to more advanced mathematical concepts:
1. Inequalities:
Number lines are instrumental in visualizing inequalities. For example, the inequality x > 6 represents all points on the number line to the right of 6. Similarly, x ≤ 8 represents all points to the left of 8, including 8 itself.
2. Absolute Value:
The absolute value of a number represents its distance from zero on the number line. For instance, the absolute value of -6 (|-6|) is 6, as it's 6 units away from zero.
3. Coordinate Plane:
By combining two perpendicular number lines, we create a coordinate plane (Cartesian coordinate system), which allows for the representation of points in two dimensions. This is fundamental to graphing and visualizing functions.
4. Functions and Graphs:
Number lines are the foundation for creating graphs of functions. The x-axis (horizontal) and y-axis (vertical) are both number lines, enabling visualization of relationships between variables.
5. Set Theory:
Number lines can be used to visually represent sets of numbers, such as intervals, unions, and intersections. This helps in understanding set operations graphically.
Conclusion: The Ubiquitous Number Line
The seemingly simple number line is a powerful tool with far-reaching applications in mathematics and beyond. Its ability to visually represent numbers, compare values, and illustrate relationships makes it an invaluable asset for learning and problem-solving. From basic comparisons of numbers like 8 and 6 to advanced concepts in algebra, calculus, and beyond, the number line remains a cornerstone of mathematical understanding. Its versatile nature ensures its continued relevance in diverse fields, providing a clear and accessible way to visualize numerical data and relationships. Mastering the number line is a crucial step towards developing a strong foundation in mathematics and its applications in various aspects of life.
Latest Posts
Latest Posts
-
How To Find The Range Of A Square Root Function
May 08, 2025
-
What Does The X Axis Of This Graph Represent
May 08, 2025
-
Cuanto Es 16 Celsius En Fahrenheit
May 08, 2025
-
Determine Which Of The Following Relations Is A Function
May 08, 2025
-
How To Find Prime Factorization Of A Large Number
May 08, 2025
Related Post
Thank you for visiting our website which covers about 8 6 On A Number Line . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.