9 3 1 1/3 What's Next
News Co
May 08, 2025 · 4 min read
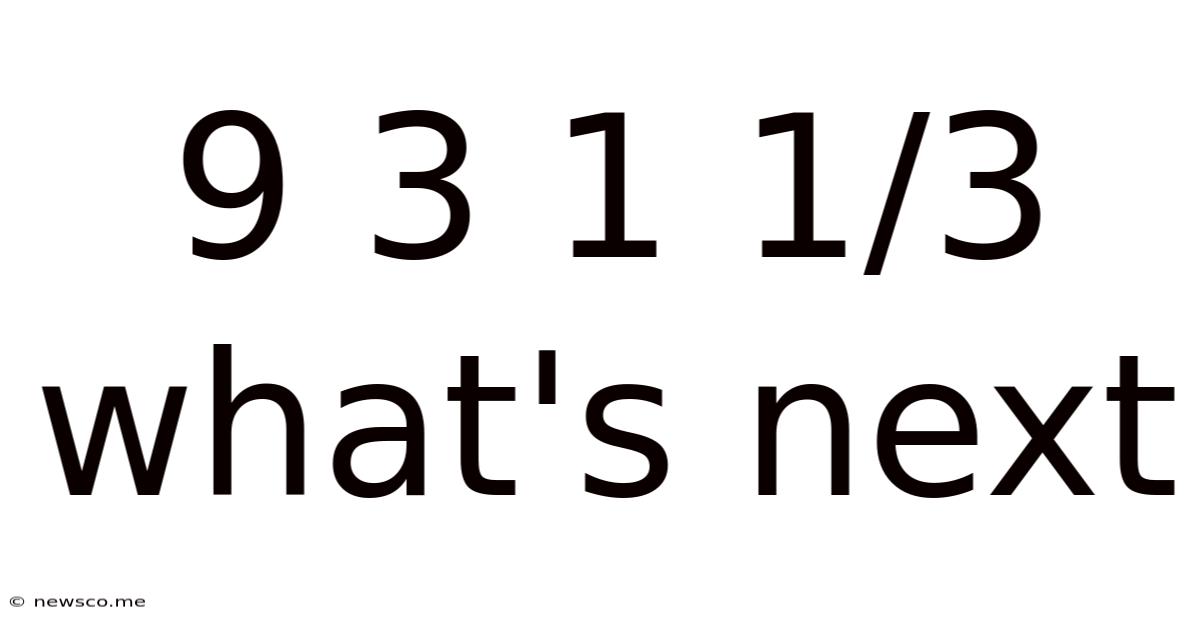
Table of Contents
9, 3, 1, ⅓: What's Next? Unraveling the Sequence and Exploring Mathematical Patterns
The deceptively simple sequence 9, 3, 1, ⅓… presents a fascinating puzzle for those intrigued by mathematical patterns. At first glance, it might seem random, but a closer examination reveals a beautiful and consistent underlying logic. This article will delve into the intricacies of this sequence, exploring various approaches to identify the next number and discussing the broader mathematical concepts it embodies. We'll uncover not just the what, but also the why behind this intriguing progression.
Identifying the Pattern: Division by 3
The most straightforward approach to understanding this sequence lies in recognizing the consistent operation applied to each preceding term: division by 3.
- 9 ÷ 3 = 3
- 3 ÷ 3 = 1
- 1 ÷ 3 = ⅓
Following this pattern, the next number in the sequence is obtained by dividing ⅓ by 3:
- ⅓ ÷ 3 = 1/9
Therefore, the sequence continues: 9, 3, 1, ⅓, 1/9, ...
Geometric Progression: A Formal Mathematical Definition
This sequence is a prime example of a geometric progression (GP). A geometric progression is a sequence where each term is obtained by multiplying the previous term by a constant value, known as the common ratio. In our case, the common ratio is ⅓. The general formula for a geometric progression is:
a, ar, ar², ar³, ar⁴,...
where:
- 'a' is the first term of the sequence.
- 'r' is the common ratio.
In our sequence, a = 9 and r = ⅓. We can express the sequence using this formula:
9, 9*(⅓), 9*(⅓)², 9*(⅓)³, 9*(⅓)⁴...
This formal definition solidifies the understanding that the sequence is not random but follows a precise mathematical rule.
Extending the Sequence: Exploring Further Terms
Using the geometric progression formula, we can easily calculate further terms in the sequence. For example:
- The fifth term: 9*(⅓)⁴ = 1/27
- The sixth term: 9*(⅓)⁵ = 1/81
- The seventh term: 9*(⅓)⁶ = 1/243
And so on. The sequence continues infinitely, approaching zero but never actually reaching it.
Visualizing the Sequence: A Graphical Representation
While the numerical representation is clear, visualizing the sequence graphically can enhance understanding. Plotting the terms on a graph with the term number on the x-axis and the value on the y-axis reveals an exponential decay curve. This visually demonstrates the rapid decrease in the value of each subsequent term as the sequence progresses.
Applications of Geometric Progressions: Real-World Examples
Geometric progressions are not merely abstract mathematical concepts; they find applications in various real-world scenarios. Some examples include:
- Compound Interest: The growth of money invested with compound interest follows a geometric progression.
- Radioactive Decay: The decay of radioactive substances over time exhibits a geometric progression.
- Population Growth (under certain conditions): In ideal environments, population growth can initially follow a geometric progression, although this is often limited by environmental constraints.
- Drug Dosage: The concentration of a drug in the bloodstream after repeated doses can sometimes follow a geometric progression, depending on the drug's metabolism and dosage frequency.
Beyond the Numbers: Exploring the Concept of Limits
The sequence's convergence towards zero highlights an important concept in calculus: limits. As the sequence progresses, the terms get increasingly smaller, approaching zero. We can express this mathematically as:
lim (n→∞) 9(⅓)^n = 0*
This means that as 'n' (the term number) approaches infinity, the value of the nth term approaches zero. This demonstrates the powerful concept of limits and how infinite sequences can approach finite values (or in this case, zero).
Alternative Interpretations: Challenging the Assumptions
While the division-by-three pattern is the most obvious and likely interpretation, it's important to acknowledge that other, albeit less likely, patterns could potentially exist. The beauty of mathematics lies in its capacity for multiple interpretations. However, without further information or a different context, the geometric progression explanation remains the most parsimonious and logical solution.
The Importance of Context: Uncovering Hidden Meanings
The significance of the sequence 9, 3, 1, ⅓... might depend on the context in which it is presented. Without knowing the source or the surrounding information, it's difficult to assign any specific meaning beyond its inherent mathematical properties. For instance, it could be a simplified representation of a more complex phenomenon, a puzzle within a larger problem, or simply an exercise in pattern recognition.
Conclusion: The Power of Pattern Recognition
The seemingly simple sequence 9, 3, 1, ⅓… provides a valuable lesson in pattern recognition and the power of mathematical reasoning. By applying basic mathematical principles, we can identify the underlying pattern, predict subsequent terms, and connect the sequence to broader mathematical concepts like geometric progressions and limits. The exploration of this sequence offers a glimpse into the elegance and utility of mathematics in understanding and modeling the world around us. The exercise underscores the importance of looking beyond the surface to uncover the underlying structures and relationships that govern seemingly simple phenomena. Further investigation into sequences like this can fuel a deeper appreciation for the beauty and interconnectedness of mathematical principles. By embracing the challenge of deciphering these numerical puzzles, we unlock not only the answer to "what's next," but also a greater understanding of the mathematical universe.
Latest Posts
Related Post
Thank you for visiting our website which covers about 9 3 1 1/3 What's Next . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.