All Formulas You Need For Algebra 2
News Co
Apr 05, 2025 · 6 min read
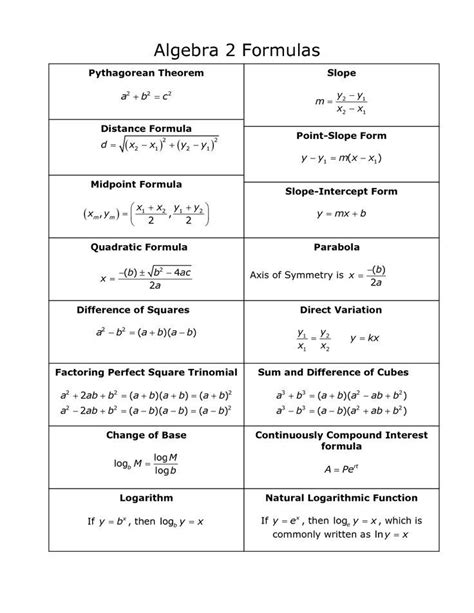
Table of Contents
All the Formulas You Need to Conquer Algebra 2
Algebra 2 builds upon the foundations laid in Algebra 1, introducing more complex concepts and techniques. Mastering the formulas is crucial for success. This comprehensive guide covers all the essential formulas you'll encounter, categorized for easy understanding and reference. We'll explore each formula, provide examples, and highlight their applications within various Algebra 2 problem-solving scenarios.
I. Linear Equations and Inequalities
Linear equations and inequalities form the bedrock of Algebra 2. Understanding these formulas is fundamental to solving more complex problems later on.
1. Slope-Intercept Form:
y = mx + b
- m: Represents the slope of the line (the rate of change of y with respect to x). A positive slope indicates an upward trend, while a negative slope indicates a downward trend. A slope of zero means a horizontal line. An undefined slope signifies a vertical line.
- b: Represents the y-intercept, the point where the line crosses the y-axis (when x = 0).
Example: y = 2x + 3. The slope (m) is 2, and the y-intercept (b) is 3.
2. Point-Slope Form:
y - y₁ = m(x - x₁)
- m: The slope of the line.
- (x₁, y₁): The coordinates of a point on the line.
This form is particularly useful when you know the slope and one point on the line.
Example: If the slope is 2 and the line passes through the point (1, 5), the equation is y - 5 = 2(x - 1).
3. Standard Form:
Ax + By = C
- A, B, and C: Are constants (usually integers). A is typically non-negative.
This form is useful for graphing and finding intercepts easily.
Example: 3x + 2y = 6
4. Solving Systems of Linear Equations:**
There are several methods to solve systems of linear equations (finding the point where two or more lines intersect):
- Substitution: Solve one equation for one variable and substitute it into the other equation.
- Elimination (Addition Method): Multiply equations by constants to eliminate one variable when adding the equations.
- Graphing: Graph both equations and find the point of intersection.
Example (Substitution): x + y = 5 and x - y = 1. Solve the first equation for x (x = 5 - y), substitute into the second equation, and solve for y. Then substitute the value of y back into either equation to solve for x.
II. Quadratic Equations and Functions
Quadratic equations and functions introduce the concept of parabolas, curves described by second-degree polynomials.
1. Standard Form of a Quadratic Equation:
ax² + bx + c = 0
- a, b, and c: Are constants (a ≠ 0).
2. Quadratic Formula:
x = [-b ± √(b² - 4ac)] / 2a
This formula is used to find the solutions (roots or zeros) of a quadratic equation. The discriminant (b² - 4ac) determines the nature of the roots:
- b² - 4ac > 0: Two distinct real roots.
- b² - 4ac = 0: One real root (repeated root).
- b² - 4ac < 0: Two complex conjugate roots.
Example: For 2x² + 3x - 2 = 0, a = 2, b = 3, c = -2. Substitute these values into the quadratic formula to find the roots.
3. Vertex Form of a Quadratic Function:
y = a(x - h)² + k
- (h, k): Represents the coordinates of the vertex (the highest or lowest point) of the parabola.
- a: Determines the parabola's opening direction (upward if a > 0, downward if a < 0) and its vertical stretch or compression.
4. Factoring Quadratic Equations:
Factoring is a method to find the roots of a quadratic equation by expressing it as a product of two linear factors. This method is only applicable to certain quadratic equations.
Example: x² + 5x + 6 = (x + 2)(x + 3) = 0. The roots are x = -2 and x = -3.
III. Polynomials
Polynomials are expressions consisting of variables and coefficients, involving only the operations of addition, subtraction, multiplication, and non-negative integer exponents.
1. Polynomial Long Division:
Used to divide a polynomial by another polynomial of lower degree. This process helps to factor polynomials and find roots.
2. Remainder Theorem:
If a polynomial P(x) is divided by (x - c), the remainder is P(c).
3. Factor Theorem:
(x - c) is a factor of P(x) if and only if P(c) = 0.
4. Fundamental Theorem of Algebra:
A polynomial of degree n has exactly n roots (counting multiplicities), which may be real or complex.
IV. Exponential and Logarithmic Functions
These functions model growth and decay processes.
1. Exponential Function:
y = abˣ
- a: The initial value (when x = 0).
- b: The base (the growth or decay factor). If b > 1, it represents exponential growth; if 0 < b < 1, it represents exponential decay.
2. Logarithmic Function:
y = logₐx
This is the inverse of the exponential function y = aˣ. It means that if y = logₐx, then aʸ = x.
3. Properties of Logarithms:
- logₐ(mn) = logₐm + logₐn
- logₐ(m/n) = logₐm - logₐn
- logₐ(mⁿ) = n logₐm
- logₐa = 1
- logₐ1 = 0
These properties are essential for simplifying and solving logarithmic equations.
V. Rational Functions
Rational functions are functions of the form f(x) = P(x)/Q(x), where P(x) and Q(x) are polynomials, and Q(x) ≠ 0.
1. Finding Vertical Asymptotes:
Vertical asymptotes occur where the denominator Q(x) = 0 and the numerator P(x) ≠ 0.
2. Finding Horizontal Asymptotes:
The behavior of the function as x approaches positive or negative infinity determines the horizontal asymptote. The rules depend on the degrees of the numerator and denominator polynomials.
VI. Sequences and Series
Sequences are ordered lists of numbers, while series are the sums of sequences.
1. Arithmetic Sequence:
A sequence where the difference between consecutive terms is constant (common difference, d).
aₙ = a₁ + (n - 1)d
- aₙ: The nth term.
- a₁: The first term.
- n: The term number.
- d: The common difference.
2. Geometric Sequence:
A sequence where the ratio between consecutive terms is constant (common ratio, r).
aₙ = a₁rⁿ⁻¹
- aₙ: The nth term.
- a₁: The first term.
- n: The term number.
- r: The common ratio.
3. Arithmetic Series:
The sum of an arithmetic sequence.
Sₙ = n/2 [2a₁ + (n - 1)d] or Sₙ = n/2 (a₁ + aₙ)
- Sₙ: The sum of the first n terms.
4. Geometric Series:
The sum of a geometric sequence.
Sₙ = a₁(1 - rⁿ) / (1 - r), r ≠ 1
S = a₁ / (1 - r), |r| < 1 (for infinite geometric series)
VII. Conic Sections
Conic sections are curves formed by the intersection of a plane and a cone.
1. Circle:
(x - h)² + (y - k)² = r²
- (h, k): The center of the circle.
- r: The radius.
2. Parabola:
Various forms exist, depending on the orientation (vertical or horizontal). The vertex form for a vertical parabola is y = a(x - h)² + k, as discussed earlier.
3. Ellipse:
(x - h)²/a² + (y - k)²/b² = 1 (horizontal major axis)
(x - h)²/b² + (y - k)²/a² = 1 (vertical major axis)
- (h, k): The center of the ellipse.
- a: Half the length of the major axis.
- b: Half the length of the minor axis.
4. Hyperbola:
(x - h)²/a² - (y - k)²/b² = 1 (horizontal transverse axis)
(y - k)²/a² - (x - h)²/b² = 1 (vertical transverse axis)
- (h, k): The center of the hyperbola.
- a: Half the length of the transverse axis.
- b: Related to the distance between the center and the foci.
This comprehensive list covers the majority of formulas crucial for success in Algebra 2. Remember that consistent practice and understanding the underlying concepts are key to mastering these formulas and applying them effectively to solve various problems. Don't hesitate to revisit this guide as needed, and remember to work through numerous practice problems to solidify your understanding. Good luck conquering Algebra 2!
Latest Posts
Related Post
Thank you for visiting our website which covers about All Formulas You Need For Algebra 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.