An Angle Measuring More Than 90 Degrees
News Co
May 08, 2025 · 6 min read
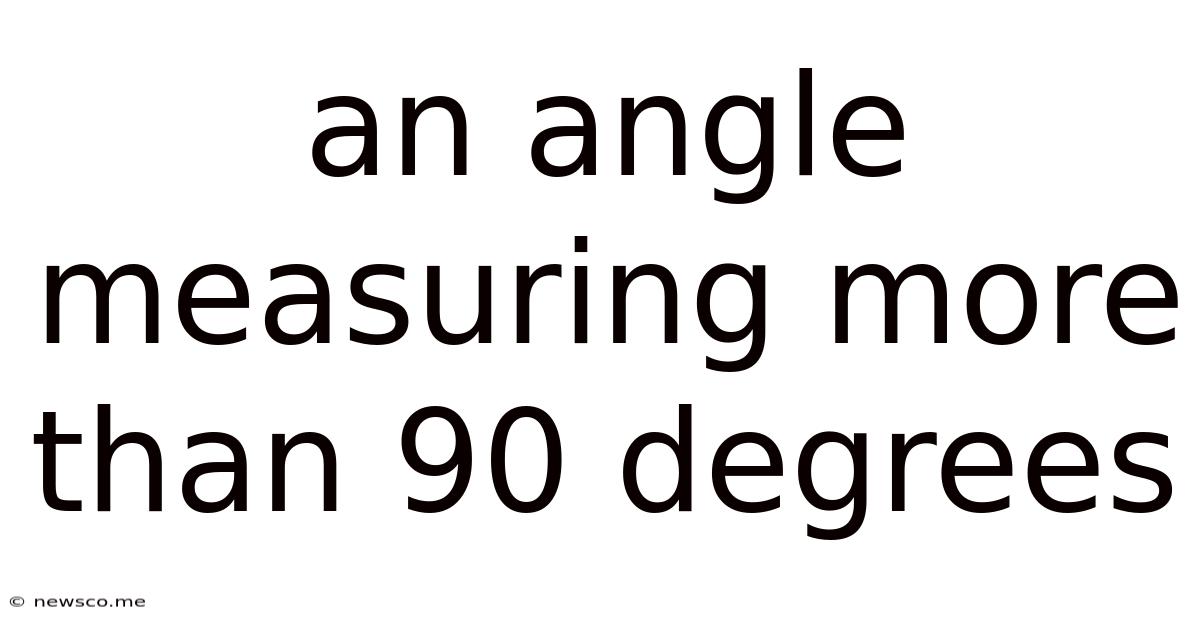
Table of Contents
Angles Greater Than 90 Degrees: A Deep Dive into Obtuse Angles and Their Applications
Angles are fundamental geometric concepts that underpin our understanding of shapes, spatial relationships, and countless real-world applications. While right angles (90 degrees) often take center stage, angles measuring more than 90 degrees, known as obtuse angles, are equally significant and deserve a closer examination. This comprehensive guide delves into the world of obtuse angles, exploring their properties, classifications, applications in various fields, and how to accurately measure and work with them.
Understanding Obtuse Angles: Definition and Properties
An obtuse angle is defined as an angle that measures greater than 90 degrees but less than 180 degrees. It falls within the range of 90° < θ < 180°, where θ represents the measure of the angle. This places it squarely between a right angle and a straight angle. Unlike acute angles (less than 90 degrees), obtuse angles represent a significant deviation from the perpendicularity often associated with right angles.
Key Properties of Obtuse Angles:
- Magnitude: The defining characteristic is its measure, exceeding 90 degrees but falling short of 180 degrees.
- Relationship to Other Angles: It's supplementary to an acute angle (their sum is 180 degrees). This relationship is crucial in solving geometric problems and understanding angle relationships within polygons.
- Visual Representation: An obtuse angle visually appears "wide open," significantly larger than a right angle. Imagine a slightly opened door—that's a visual representation of an obtuse angle.
- Role in Polygons: Obtuse angles play a significant role in defining various polygons, particularly those that are not regular. For instance, many irregular quadrilaterals and other polygons contain at least one obtuse angle.
Measuring Obtuse Angles: Techniques and Tools
Accurately measuring obtuse angles is crucial in various applications. Several methods and tools can be employed:
1. Protractor: The most common method involves using a protractor. Align the base line of the protractor with one ray of the angle, ensuring the center point of the protractor aligns with the vertex of the angle. Then, read the measurement where the other ray intersects the protractor's scale. Remember that protractors measure angles up to 180 degrees, making them perfectly suited for measuring obtuse angles.
2. Digital Angle Gauge: For more precise measurements, especially in industrial settings, a digital angle gauge is preferred. These devices use electronic sensors to provide accurate readings, often with a high degree of precision. They are commonly used in manufacturing, engineering, and construction.
3. Trigonometric Functions: If the lengths of the sides of a triangle containing the obtuse angle are known, trigonometric functions (such as the cosine rule) can be utilized to calculate the angle's measure indirectly. This method is powerful when direct measurement isn't feasible.
4. Software and Applications: Various computer software and mobile apps offer tools for measuring angles in images or diagrams. These applications utilize image analysis techniques to determine the angle's measurement, offering a convenient and often accurate method.
Obtuse Angles in Geometry and Trigonometry
Obtuse angles are far from a mere geometric curiosity; they hold significant importance in various geometric and trigonometric contexts:
1. Triangles: Obtuse triangles, containing one obtuse angle, possess unique properties distinct from acute and right-angled triangles. The trigonometric relationships within obtuse triangles differ slightly from those in acute triangles, demanding careful attention to the signs of trigonometric functions.
2. Polygons: The angles of polygons, including quadrilaterals, pentagons, hexagons, and beyond, can include obtuse angles. The sum of interior angles in any polygon is dependent only on the number of sides (n), given by the formula (n-2) x 180 degrees. The presence of obtuse angles influences the overall shape and properties of the polygon.
3. Coordinate Geometry: In coordinate geometry, obtuse angles can arise when calculating the angle between two lines or vectors. The dot product of vectors, when normalized, provides the cosine of the angle between them, which can be used to determine the angle, including obtuse ones.
Real-World Applications of Obtuse Angles
The relevance of obtuse angles extends far beyond theoretical geometry. They feature prominently in various aspects of the real world:
1. Engineering and Architecture: In structural engineering and architecture, understanding obtuse angles is critical. The angles of support structures, roof designs, and bridge construction often involve obtuse angles to ensure stability and functionality.
2. Construction and Carpentry: From cutting angles for roof trusses to laying out foundations, construction and carpentry frequently require the precise measurement and creation of obtuse angles. Accurate angle measurement and calculation are vital for creating strong and reliable structures.
3. Navigation and Surveying: Determining directions and distances often involves working with angles, including obtuse angles, in navigation and surveying. These calculations are crucial for mapmaking, route planning, and land measurement.
4. Computer Graphics and Animation: In computer-aided design (CAD) and computer animation, understanding and manipulating angles, including obtuse angles, is fundamental to creating realistic three-dimensional models and scenes.
5. Optics: The refraction of light at an oblique angle can result in the formation of obtuse angles. This phenomenon is critical in understanding optical systems like lenses and prisms.
6. Sports and Games: The trajectory of projectiles in sports like baseball, basketball, and golf often involves obtuse angles. Understanding these angles is crucial in analyzing the motion of the projectile and predicting its landing point.
Solving Problems Involving Obtuse Angles
Solving problems involving obtuse angles often involves combining geometric principles, trigonometric functions, and algebraic techniques. Here are some general approaches:
- Identify the type of problem: Determine if the problem involves finding the measure of an obtuse angle, using an obtuse angle in calculations, or analyzing the properties of shapes containing obtuse angles.
- Apply relevant geometric theorems: Utilize relevant theorems such as the sum of angles in a triangle or polygon, angle properties of parallel lines, or properties of specific shapes.
- Use trigonometric functions: If necessary, employ trigonometric functions such as sine, cosine, and tangent to calculate unknown sides or angles in triangles.
- Break down complex shapes: If the problem involves complex shapes, decompose them into simpler shapes such as triangles or rectangles to facilitate calculations.
- Check your work: Always verify the reasonableness of your answer. For example, ensure the calculated angle indeed falls within the range of an obtuse angle (90° < θ < 180°).
Conclusion: The Unsung Importance of Obtuse Angles
Obtuse angles, often overlooked in favor of their more "famous" right-angled counterparts, are indispensable components of various mathematical and real-world applications. Their importance spans numerous disciplines, from the fundamental principles of geometry and trigonometry to the intricate designs of engineering marvels. By understanding the properties, measurements, and applications of obtuse angles, we gain a deeper appreciation of the elegance and practicality of geometry in the world around us. Mastering the concept of obtuse angles unlocks a deeper understanding of the world of shapes, spatial relationships, and problem-solving in countless fields.
Latest Posts
Latest Posts
-
How Many Sides Does Regular Polygon Have
May 08, 2025
-
How Many Glasses Of Water Is 3 Liters
May 08, 2025
-
2 Liters Equals How Many Milliliters
May 08, 2025
-
How Long Is 120 Seconds In Minutes
May 08, 2025
-
Same Side Exterior Angles Are Congruent
May 08, 2025
Related Post
Thank you for visiting our website which covers about An Angle Measuring More Than 90 Degrees . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.