Area Of Composite Figures Answer Key
News Co
Apr 03, 2025 · 6 min read
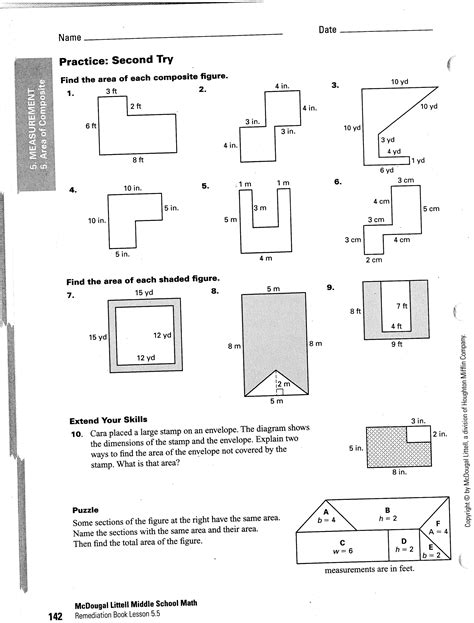
Table of Contents
Area of Composite Figures: A Comprehensive Guide with Answer Key
Calculating the area of composite figures can seem daunting at first, but with a systematic approach and a solid understanding of basic shapes, it becomes a manageable task. This comprehensive guide will break down the process step-by-step, providing you with the tools and techniques to accurately determine the area of any composite figure, along with example problems and an answer key.
Understanding Composite Figures
A composite figure, also known as a complex figure, is a geometric shape that is formed by combining two or more simpler shapes, such as rectangles, triangles, circles, and semicircles. These simpler shapes can be combined in various ways, resulting in a wide array of complex shapes. The key to finding the area of a composite figure lies in decomposing it into its constituent parts.
Why is understanding composite figures important?
The ability to calculate the area of composite figures is crucial in many real-world applications, including:
- Engineering and Architecture: Calculating the surface area of buildings, bridges, and other structures.
- Interior Design: Determining the amount of paint or flooring needed for a room.
- Land Surveying: Measuring the area of irregularly shaped plots of land.
- Manufacturing: Calculating the material needed to create parts with complex shapes.
Strategies for Solving Area of Composite Figures Problems
The process of finding the area of a composite figure typically involves these key steps:
-
Identify the Component Shapes: Carefully examine the composite figure and identify the simpler shapes that make it up (rectangles, squares, triangles, circles, semicircles, etc.). Sketching these individual shapes separately can be helpful.
-
Find Missing Dimensions: You may need to use geometry principles (Pythagorean theorem, properties of similar triangles, etc.) to determine missing lengths or angles necessary to calculate the area of the component shapes.
-
Calculate the Area of Each Component Shape: Use the appropriate area formulas for each shape. Remember these common formulas:
- Rectangle: Area = length × width
- Square: Area = side × side
- Triangle: Area = (1/2) × base × height
- Circle: Area = π × radius²
- Semicircle: Area = (1/2) × π × radius²
-
Add or Subtract Areas: Once you have the area of each component shape, add the areas together if the shapes are combined to form the composite figure. If one shape is cut out of another, subtract the smaller area from the larger area.
Example Problems with Detailed Solutions and Answer Key
Let's work through several example problems to illustrate these steps.
Problem 1:
A figure is composed of a rectangle with a length of 10 cm and a width of 5 cm, and a semicircle with a diameter of 5 cm attached to one side of the rectangle. Find the total area of the figure.
Solution:
-
Component Shapes: The figure is composed of a rectangle and a semicircle.
-
Dimensions: The rectangle has length = 10 cm and width = 5 cm. The semicircle has a diameter of 5 cm, so its radius is 2.5 cm.
-
Area Calculations:
- Area of rectangle = length × width = 10 cm × 5 cm = 50 cm²
- Area of semicircle = (1/2) × π × radius² = (1/2) × π × (2.5 cm)² ≈ 9.82 cm²
-
Total Area: Total area = Area of rectangle + Area of semicircle = 50 cm² + 9.82 cm² ≈ 59.82 cm²
Answer: The total area of the figure is approximately 59.82 cm².
Problem 2:
A figure is made up of a square with sides of 8 cm and a triangle with a base of 8 cm and a height of 6 cm attached to one side of the square. Find the total area.
Solution:
-
Component Shapes: A square and a triangle.
-
Dimensions: The square has sides of 8 cm. The triangle has a base of 8 cm and a height of 6 cm.
-
Area Calculations:
- Area of square = side × side = 8 cm × 8 cm = 64 cm²
- Area of triangle = (1/2) × base × height = (1/2) × 8 cm × 6 cm = 24 cm²
-
Total Area: Total area = Area of square + Area of triangle = 64 cm² + 24 cm² = 88 cm²
Answer: The total area of the figure is 88 cm².
Problem 3:
A larger rectangle with dimensions 12 cm by 10 cm has a smaller rectangle with dimensions 4 cm by 3 cm cut out from its center. What is the remaining area?
Solution:
-
Component Shapes: Two rectangles.
-
Dimensions: Larger rectangle: 12 cm by 10 cm. Smaller rectangle: 4 cm by 3 cm.
-
Area Calculations:
- Area of larger rectangle = 12 cm × 10 cm = 120 cm²
- Area of smaller rectangle = 4 cm × 3 cm = 12 cm²
-
Remaining Area: Remaining area = Area of larger rectangle – Area of smaller rectangle = 120 cm² – 12 cm² = 108 cm²
Answer: The remaining area is 108 cm².
Problem 4: (More Challenging)
A figure consists of a circle with a radius of 7 cm and a square with a side length of 14 cm. The circle is inscribed within the square. Find the area of the region outside the circle but inside the square.
Solution:
-
Component Shapes: A circle and a square.
-
Dimensions: Circle radius = 7 cm. Square side = 14 cm.
-
Area Calculations:
- Area of square = side × side = 14 cm × 14 cm = 196 cm²
- Area of circle = π × radius² = π × (7 cm)² ≈ 153.94 cm²
-
Area of the Region: Area outside circle but inside square = Area of square – Area of circle = 196 cm² – 153.94 cm² ≈ 42.06 cm²
Answer: The area of the region outside the circle but inside the square is approximately 42.06 cm².
Advanced Composite Figures and Problem-Solving Techniques
As you progress, you'll encounter more complex composite figures that may require more sophisticated techniques:
-
Breaking Down Irregular Shapes: Divide irregular shapes into smaller, manageable shapes (triangles, rectangles, etc.) using lines that create right angles.
-
Using Trigonometry: Utilize trigonometric functions (sine, cosine, tangent) to find missing dimensions when angles are given.
-
Coordinate Geometry: Use coordinates to define the vertices of the shapes and then calculate the areas using formulas based on coordinate differences.
-
Subtracting Areas: Remember to subtract areas when a shape is cut out from another.
Practice Problems and Answer Key
Here are some additional practice problems to test your understanding:
Problem 5: Find the area of a figure composed of a rectangle with length 15 cm and width 8 cm and a triangle with base 8 cm and height 5 cm attached to one side of the rectangle.
Problem 6: A semicircle with a diameter of 10 cm is placed on top of a rectangle with length 10 cm and width 6 cm. Calculate the total area.
Problem 7: A square with sides of 12 cm has a smaller square with sides of 4 cm cut out from one corner. What is the remaining area?
Problem 8: A trapezoid with bases of 6 cm and 10 cm and a height of 8 cm is combined with a triangle with a base of 6 cm and height of 4 cm to form a composite figure. Calculate the total area.
Answer Key:
- Problem 5: 146 cm²
- Problem 6: 87.85 cm² (approximately)
- Problem 7: 128 cm²
- Problem 8: 76 cm²
By mastering these techniques and practicing regularly, you'll build confidence in calculating the areas of even the most intricate composite figures. Remember to break down complex shapes into simpler components, use appropriate area formulas, and always double-check your work! Consistent practice is the key to success in mastering this important geometrical skill.
Latest Posts
Related Post
Thank you for visiting our website which covers about Area Of Composite Figures Answer Key . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.