Common Denominator Of 7 And 8
News Co
Apr 08, 2025 · 5 min read
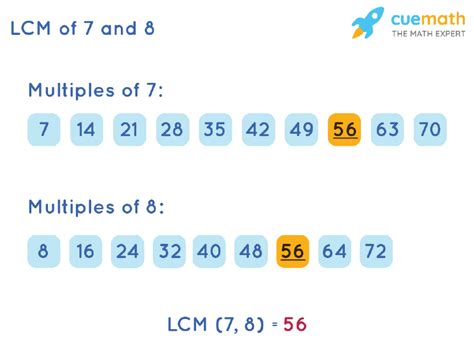
Table of Contents
Finding the Common Denominator of 7 and 8: A Deep Dive into Fractions
Finding the common denominator of 7 and 8 might seem like a simple task, especially for those well-versed in mathematics. However, understanding the underlying principles and exploring different approaches to solving this problem can be incredibly insightful, particularly for those seeking a deeper grasp of fractions and their applications. This comprehensive guide will delve into the intricacies of finding the common denominator, exploring various methods and expanding on the conceptual understanding of this fundamental mathematical concept.
Understanding Common Denominators
Before we tackle the specific problem of finding the common denominator of 7 and 8, let's establish a solid foundation. A common denominator is a shared multiple of the denominators of two or more fractions. It's the crucial element that allows us to add, subtract, compare, and generally perform operations on fractions with different denominators. Without a common denominator, direct addition or subtraction is impossible. For instance, you cannot directly add 1/7 and 1/8; you need a common denominator first.
Methods for Finding the Common Denominator
Several methods exist for determining the common denominator. We'll explore the most common and effective techniques, highlighting their advantages and disadvantages.
1. Listing Multiples: A Simple Approach
The most straightforward approach is to list the multiples of each denominator until a common multiple is found.
- Multiples of 7: 7, 14, 21, 28, 35, 42, 49, 56, 63, 70, 77, 84...
- Multiples of 8: 8, 16, 24, 32, 40, 48, 56, 64, 72, 80, 88, 96...
Notice that 56 appears in both lists. Therefore, 56 is a common denominator of 7 and 8. This method is simple for smaller numbers but can become cumbersome with larger denominators.
2. Prime Factorization: A More Efficient Method
Prime factorization is a more sophisticated, yet efficient method, particularly useful for larger numbers. This involves breaking down each denominator into its prime factors.
- Prime factorization of 7: 7 (7 is a prime number)
- Prime factorization of 8: 2 x 2 x 2 = 2³
To find the least common denominator (LCD), we take the highest power of each prime factor present in either factorization:
- The highest power of 2 is 2³ = 8
- The highest power of 7 is 7¹ = 7
Multiply these together: 8 x 7 = 56. Thus, the LCD of 7 and 8 is 56. This method is far more efficient for larger numbers where listing multiples becomes impractical.
3. The Formula Approach: A Direct Calculation
A formula can be used to directly calculate the least common multiple (LCM) of two numbers, which is equivalent to the least common denominator (LCD) when dealing with fractions. The formula involves the greatest common divisor (GCD) which can be found using Euclid's Algorithm.
-
Finding the GCD of 7 and 8 using Euclid's Algorithm:
- 8 = 7 * 1 + 1
- 7 = 1 * 7 + 0
- The GCD is 1.
-
Using the formula: LCM(a, b) = (a * b) / GCD(a, b)
- LCM(7, 8) = (7 * 8) / 1 = 56
This formula provides a direct calculation, eliminating the need for listing multiples or prime factorization. However, understanding the underlying principles of GCD and LCM is crucial for effective application.
Why 56 is the Least Common Denominator
We've established that 56 is a common denominator. But why is it the least common denominator? The least common denominator (LCD) is the smallest common multiple of the denominators. Using a smaller number simplifies calculations and reduces the risk of working with unnecessarily large fractions. Any other common multiple of 7 and 8 (e.g., 112, 168) would be a valid common denominator, but it would lead to more complex calculations.
Applying the Common Denominator
Now that we know the LCD of 7 and 8 is 56, let's see how to apply this to adding or subtracting fractions.
Let's add 1/7 and 1/8:
-
Convert to equivalent fractions:
- 1/7 = (1 x 8) / (7 x 8) = 8/56
- 1/8 = (1 x 7) / (8 x 7) = 7/56
-
Add the numerators:
- 8/56 + 7/56 = 15/56
Therefore, 1/7 + 1/8 = 15/56.
Similarly, if we were subtracting, we'd follow the same process, subtracting the numerators after converting to a common denominator.
Beyond 7 and 8: Expanding the Concept
The principles and methods discussed here extend beyond the simple case of 7 and 8. These techniques are applicable to any set of numbers, no matter how large or complex. The choice of method (listing multiples, prime factorization, or the formula approach) often depends on the size and complexity of the numbers involved. For smaller numbers, listing multiples may suffice. However, for larger numbers, prime factorization or the formula approach proves significantly more efficient and less prone to errors.
Real-World Applications of Common Denominators
Understanding common denominators is far from a purely academic exercise. It has wide-ranging practical applications across various fields:
-
Construction and Engineering: Accurate measurements and calculations are vital in construction and engineering. Common denominators ensure precise computations when dealing with fractions of units (e.g., inches, meters).
-
Cooking and Baking: Recipes often involve fractional measurements. Accurate calculations involving fractions are essential for achieving consistent results.
-
Finance: Working with fractions is crucial in financial calculations involving percentages, shares, and interest rates.
-
Data Analysis: Understanding common denominators is useful when comparing datasets involving fractional values.
Further Exploration: Advanced Fraction Concepts
For those seeking to deepen their understanding of fractions, further exploration into related concepts is encouraged:
-
Least Common Multiple (LCM): A deeper dive into the mathematical properties and efficient algorithms for calculating LCMs will enhance understanding.
-
Greatest Common Divisor (GCD): Exploring different algorithms for finding GCDs, including the Euclidean algorithm and its variants, will provide a richer understanding.
-
Continued Fractions: Continued fractions offer an alternative representation of rational numbers, providing insights into their properties and approximations.
-
Rational Numbers: A comprehensive understanding of rational numbers – numbers expressible as a ratio of two integers – is crucial for mastering fraction operations.
Conclusion: Mastering the Art of Common Denominators
Finding the common denominator of 7 and 8, while seemingly straightforward, serves as a gateway to understanding broader mathematical concepts related to fractions. This guide has explored multiple approaches to finding the LCD, emphasizing the importance of the least common denominator and illustrating its applications across diverse fields. By mastering the art of finding common denominators, you equip yourself with a fundamental skill applicable across numerous mathematical and real-world scenarios. Whether you're adding fractions, comparing quantities, or tackling complex calculations, a solid understanding of common denominators is indispensable.
Latest Posts
Latest Posts
-
Find The Least Common Multiple Of 4 And 7
Apr 08, 2025
-
Find The Inverse Of The Function Y 2x2 2
Apr 08, 2025
-
What Number Is A Multiple Of 3
Apr 08, 2025
-
Long Division Problems For 6th Graders
Apr 08, 2025
-
What Is A Prime Factorization Of 24
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about Common Denominator Of 7 And 8 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.