Common Factors Of 27 And 45
News Co
Apr 03, 2025 · 6 min read
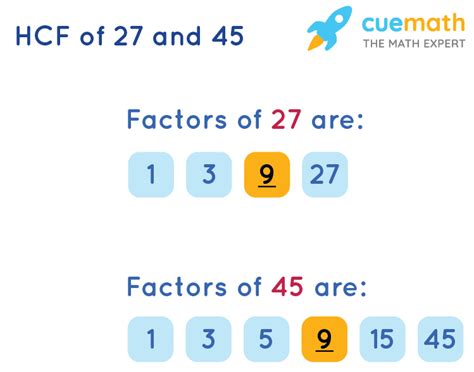
Table of Contents
- Common Factors Of 27 And 45
- Table of Contents
- Unveiling the Secrets of Common Factors: A Deep Dive into 27 and 45
- Understanding Factors and Common Factors
- Finding the Common Factors of 27 and 45: Method 1 - Listing Factors
- Finding the Common Factors of 27 and 45: Method 2 - Prime Factorization
- Greatest Common Factor (GCF)
- Applications of Common Factors and GCF
- Beyond the Basics: Exploring Related Concepts
- Practical Applications and Real-World Examples
- Conclusion: The Importance of Fundamental Concepts
- Latest Posts
- Related Post
Unveiling the Secrets of Common Factors: A Deep Dive into 27 and 45
Finding the common factors of two numbers might seem like a simple arithmetic task, but it's a fundamental concept in mathematics with applications far beyond the classroom. Understanding common factors is crucial for simplifying fractions, solving algebraic equations, and even exploring more advanced mathematical topics. This comprehensive guide will explore the common factors of 27 and 45, providing a detailed explanation of the process, highlighting different methods, and delving into the underlying mathematical principles. We'll also look at how this seemingly basic concept connects to broader mathematical ideas.
Understanding Factors and Common Factors
Before we delve into the specifics of 27 and 45, let's solidify our understanding of key terms.
Factors: Factors of a number are whole numbers that divide into that number without leaving a remainder. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12. Each of these numbers divides evenly into 12.
Common Factors: When we consider two or more numbers, the common factors are the numbers that are factors of all of the given numbers. These are the numbers that divide evenly into each of the numbers without leaving a remainder.
Finding the Common Factors of 27 and 45: Method 1 - Listing Factors
The most straightforward method to find the common factors is to list all the factors of each number and then identify the numbers that appear in both lists.
Factors of 27: 1, 3, 9, 27
Factors of 45: 1, 3, 5, 9, 15, 45
Comparing the two lists, we can see that the common factors of 27 and 45 are 1, 3, and 9. These are the only whole numbers that divide evenly into both 27 and 45.
Finding the Common Factors of 27 and 45: Method 2 - Prime Factorization
Prime factorization is a powerful technique for finding common factors, especially when dealing with larger numbers. It involves expressing each number as a product of its prime factors (numbers divisible only by 1 and themselves).
Prime Factorization of 27:
27 = 3 x 3 x 3 = 3³
Prime Factorization of 45:
45 = 3 x 3 x 5 = 3² x 5
To find the common factors, we identify the prime factors that are common to both numbers and their lowest power. Both 27 and 45 have 3 as a prime factor. The lowest power of 3 present in both factorizations is 3².
Therefore, the common factors are obtained by multiplying the common prime factors raised to their lowest power:
3¹ = 3 3² = 9 3⁰ (which is 1) = 1
So the common factors are 1, 3, and 9. This method confirms the results from the first method.
Greatest Common Factor (GCF)
The greatest common factor (GCF), also known as the highest common factor (HCF), is the largest number that divides evenly into all the given numbers. In the case of 27 and 45, the GCF is 9. This is the largest of the common factors we identified. The GCF is a crucial concept in simplifying fractions and solving various mathematical problems.
Applications of Common Factors and GCF
The concepts of common factors and the greatest common factor have wide-ranging applications in mathematics and beyond. Here are some key areas:
-
Simplifying Fractions: The GCF is used to simplify fractions to their lowest terms. For example, the fraction 27/45 can be simplified by dividing both the numerator and denominator by their GCF, which is 9. This simplifies the fraction to 3/5.
-
Algebra: Common factors are essential in factoring algebraic expressions. Finding the common factor allows us to simplify and solve equations more efficiently.
-
Geometry: Common factors play a role in problems involving geometric shapes and their dimensions. For example, finding the greatest common divisor (GCD) of the dimensions of a rectangle can help in determining the largest square tile that can perfectly cover the rectangle's area.
-
Number Theory: Common factors and GCF are fundamental concepts in number theory, which is the branch of mathematics that studies integers and their properties.
-
Computer Science: The efficient computation of the GCF is important in various algorithms used in computer science, particularly in cryptography and data structures. The Euclidean Algorithm, for example, provides an efficient method for finding the GCF of two integers.
Beyond the Basics: Exploring Related Concepts
Understanding common factors opens doors to more advanced mathematical concepts. Here are some related ideas to consider:
-
Least Common Multiple (LCM): The least common multiple (LCM) is the smallest number that is a multiple of all the given numbers. The relationship between the GCF and LCM is expressed by the formula: GCF(a, b) x LCM(a, b) = a x b, where 'a' and 'b' are the two numbers.
-
Euclidean Algorithm: This is an efficient algorithm for computing the GCF of two integers. It's based on the principle that the GCF of two numbers does not change if the larger number is replaced by its difference with the smaller number. This process is repeated until the two numbers are equal, and that number is the GCF.
-
Modular Arithmetic: Concepts like divisibility and common factors are fundamental to modular arithmetic, which deals with remainders after division.
-
Abstract Algebra: The concept of GCF extends to more abstract algebraic structures, playing a role in ring theory and ideal theory.
Practical Applications and Real-World Examples
The principles of common factors extend beyond theoretical mathematics and find practical application in various real-world scenarios:
-
Sharing equally: Imagine you have 27 apples and 45 oranges. You want to divide them into identical bags, with the same number of apples and oranges in each bag. The greatest number of bags you can create is the GCF of 27 and 45, which is 9. Each bag will contain 3 apples and 5 oranges.
-
Construction and Design: In construction, understanding GCF can help in determining the optimal size of tiles or bricks to cover a given area efficiently without any cuts or wastage.
-
Music Theory: Common factors and multiples play a role in understanding musical intervals and harmonies.
-
Scheduling and Time Management: Determining the common time slots for meetings or events involves finding the common multiples or factors of different time intervals.
Conclusion: The Importance of Fundamental Concepts
While finding the common factors of 27 and 45 might appear to be a simple exercise, it underpins much more profound mathematical principles and has a wide array of applications across various fields. This exploration showcases the power of seemingly basic concepts and how they form the foundation of more advanced mathematical ideas. Understanding common factors, the GCF, and related concepts is crucial for anyone seeking a deeper understanding of mathematics and its applications in the real world. Mastering these fundamentals opens the door to tackling more complex problems and appreciating the interconnectedness of mathematical ideas.
Latest Posts
Related Post
Thank you for visiting our website which covers about Common Factors Of 27 And 45 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.