Derivative Of Sin Cos Tan Sec Csc Cot
News Co
Apr 05, 2025 · 5 min read
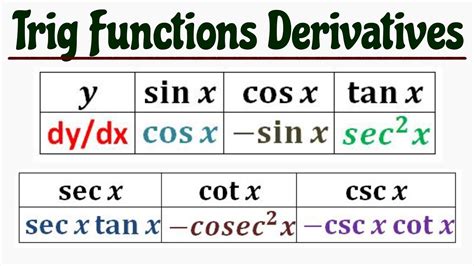
Table of Contents
Derivatives of Trigonometric Functions: A Comprehensive Guide
Understanding the derivatives of trigonometric functions – sin x, cos x, tan x, sec x, csc x, and cot x – is fundamental to calculus. These derivatives form the bedrock of many applications, from physics and engineering to economics and computer graphics. This comprehensive guide will explore each derivative individually, providing clear explanations, derivations (where applicable), and practical examples to solidify your understanding.
Understanding the Derivative
Before diving into the specifics of trigonometric derivatives, let's briefly revisit the concept of a derivative. The derivative of a function, f(x), denoted as f'(x) or df/dx, represents the instantaneous rate of change of the function at a specific point. Geometrically, it represents the slope of the tangent line to the curve of the function at that point.
The derivative is found using the limit definition:
f'(x) = lim (h→0) [(f(x + h) - f(x)) / h]
While we'll use this definition for some derivations, we'll also leverage existing derivative rules (like the quotient rule and chain rule) for efficiency.
1. Derivative of sin x
The derivative of sin x is one of the most fundamental results in calculus. It's derived using the limit definition and trigonometric identities.
Derivation (using limit definition):
d/dx (sin x) = lim (h→0) [(sin(x + h) - sin x) / h]
Using the trigonometric identity sin(A + B) = sin A cos B + cos A sin B:
= lim (h→0) [(sin x cos h + cos x sin h - sin x) / h]
= lim (h→0) [(sin x (cos h - 1) / h) + (cos x (sin h / h))]
Since lim (h→0) (sin h / h) = 1 and lim (h→0) (cos h - 1) / h = 0:
= cos x
Therefore:
d/dx (sin x) = cos x
Example: Find the derivative of f(x) = 3sin x. Using the constant multiple rule, f'(x) = 3cos x.
2. Derivative of cos x
Similar to sin x, the derivative of cos x can be derived using the limit definition and trigonometric identities. However, the result is slightly different.
Derivation (using limit definition): (This derivation follows a similar pattern to the sin x derivation, utilizing the cosine addition formula and limit properties.)
d/dx (cos x) = lim (h→0) [(cos(x + h) - cos x) / h]
Using cos(A + B) = cos A cos B - sin A sin B:
= lim (h→0) [(cos x cos h - sin x sin h - cos x) / h]
= lim (h→0) [(cos x (cos h - 1) / h) - (sin x (sin h / h))]
Using the limits established previously:
= -sin x
Therefore:
d/dx (cos x) = -sin x
Notice the negative sign. This highlights the fundamental difference in the behavior of the sine and cosine functions.
Example: Find the derivative of g(x) = cos(2x). Using the chain rule, g'(x) = -2sin(2x).
3. Derivative of tan x
The tangent function is defined as tan x = sin x / cos x. Therefore, we can find its derivative using the quotient rule:
Quotient Rule: If f(x) = u(x) / v(x), then f'(x) = [v(x)u'(x) - u(x)v'(x)] / [v(x)]²
Applying the quotient rule:
d/dx (tan x) = d/dx (sin x / cos x) = [(cos x)(cos x) - (sin x)(-sin x)] / (cos x)²
= (cos²x + sin²x) / (cos²x)
= 1 / (cos²x)
= sec²x
Therefore:
d/dx (tan x) = sec²x
Example: Find the derivative of h(x) = 5tan(3x). Using the chain rule, h'(x) = 15sec²(3x).
4. Derivative of sec x
The secant function is defined as sec x = 1 / cos x. Again, we utilize the quotient rule (or, alternatively, the power rule combined with the chain rule):
d/dx (sec x) = d/dx (1 / cos x) = [ (cos x)(0) - (1)(-sin x) ] / (cos x)²
= sin x / (cos²x)
= (1/cos x)(sin x / cos x)
= sec x tan x
Therefore:
d/dx (sec x) = sec x tan x
Example: Find the derivative of i(x) = sec(x²). Using the chain rule, i'(x) = 2x sec(x²) tan(x²).
5. Derivative of csc x
The cosecant function is defined as csc x = 1 / sin x. Using the quotient rule (or power rule with chain rule):
d/dx (csc x) = d/dx (1 / sin x) = [(sin x)(0) - (1)(cos x)] / (sin x)²
= -cos x / (sin²x)
= -(1/sin x)(cos x / sin x)
= -csc x cot x
Therefore:
d/dx (csc x) = -csc x cot x
Example: Find the derivative of j(x) = 2csc(4x). Using the chain rule, j'(x) = -8csc(4x)cot(4x).
6. Derivative of cot x
The cotangent function is defined as cot x = cos x / sin x. Using the quotient rule:
d/dx (cot x) = d/dx (cos x / sin x) = [(sin x)(-sin x) - (cos x)(cos x)] / (sin x)²
= (-sin²x - cos²x) / (sin²x)
= -(sin²x + cos²x) / (sin²x)
= -1 / (sin²x)
= -csc²x
Therefore:
d/dx (cot x) = -csc²x
Example: Find the derivative of k(x) = cot(x/2). Using the chain rule, k'(x) = -1/2 csc²(x/2).
Higher-Order Derivatives and Applications
The derivatives we've explored are first-order derivatives. You can find second-order, third-order, and higher-order derivatives by repeatedly differentiating. These higher-order derivatives are crucial in various applications:
-
Physics: Analyzing motion (velocity, acceleration, jerk). For instance, the derivative of position with respect to time gives velocity, and the derivative of velocity gives acceleration. Trigonometric functions often model oscillatory motion (like a pendulum).
-
Engineering: Modeling oscillations in circuits, mechanical systems, and structural analysis.
-
Economics: Modeling cyclical patterns in economic indicators.
-
Computer Graphics: Creating smooth curves and animations.
Memorizing the Derivatives
While understanding the derivations is important for a solid foundation, memorizing the results is crucial for efficiency in problem-solving. Here's a helpful summary:
- d/dx (sin x) = cos x
- d/dx (cos x) = -sin x
- d/dx (tan x) = sec²x
- d/dx (sec x) = sec x tan x
- d/dx (csc x) = -csc x cot x
- d/dx (cot x) = -csc²x
Mastering these derivatives is a significant step towards success in calculus. By understanding their derivations and practicing numerous examples, you'll build a strong foundation for tackling more complex problems involving trigonometric functions. Remember to combine these derivatives with other calculus rules (chain rule, product rule, quotient rule) to solve a wider range of problems.
Latest Posts
Related Post
Thank you for visiting our website which covers about Derivative Of Sin Cos Tan Sec Csc Cot . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.