Distributive Property Of Multiplication Over Addition Example
News Co
Apr 06, 2025 · 4 min read
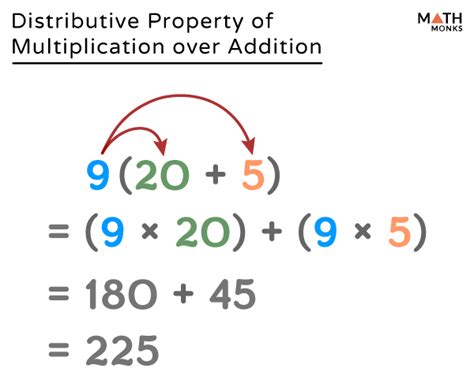
Table of Contents
The Distributive Property of Multiplication Over Addition: A Comprehensive Guide with Examples
The distributive property is a fundamental concept in mathematics, particularly in algebra. Understanding it is crucial for simplifying expressions, solving equations, and progressing to more advanced mathematical concepts. This comprehensive guide will delve into the distributive property of multiplication over addition, providing a thorough explanation, diverse examples, and practical applications.
What is the Distributive Property?
The distributive property states that multiplying a number by a sum is the same as multiplying the number by each addend and then adding the products. In simpler terms, it allows you to "distribute" the multiplication across the terms inside the parentheses. This applies to both addition and subtraction.
Mathematically, it's represented as:
- a(b + c) = ab + ac (Distributive property of multiplication over addition)
- a(b - c) = ab - ac (Distributive property of multiplication over subtraction)
Where 'a', 'b', and 'c' can be any real numbers, variables, or even expressions.
Understanding the Concept Through Visual Representation
Imagine you have 3 bags, each containing 2 apples and 3 oranges. To find the total number of fruits, you can do it in two ways:
-
Directly: Count the total number of fruits in each bag (2 + 3 = 5 fruits) and then multiply by the number of bags (5 fruits/bag * 3 bags = 15 fruits).
-
Distributively: Count the total number of apples (2 apples/bag * 3 bags = 6 apples) and the total number of oranges (3 oranges/bag * 3 bags = 9 oranges). Then add the total apples and oranges (6 + 9 = 15 fruits).
Both methods yield the same result, illustrating the distributive property in action. This visual representation makes the concept more intuitive and easier to grasp.
Examples of the Distributive Property
Let's explore various examples demonstrating the distributive property's application in different scenarios.
Example 1: Basic Numerical Expression
Simplify the expression: 5(2 + 4)
Using the distributive property:
5(2 + 4) = 5(2) + 5(4) = 10 + 20 = 30
Direct calculation:
5(2 + 4) = 5(6) = 30
Both methods yield the same answer, verifying the distributive property.
Example 2: Variables and Numbers
Simplify the expression: 3(x + 5)
Applying the distributive property:
3(x + 5) = 3(x) + 3(5) = 3x + 15
This simplifies the expression without changing its value.
Example 3: Distributive Property with Subtraction
Simplify the expression: 2(7 - 3)
Using the distributive property:
2(7 - 3) = 2(7) - 2(3) = 14 - 6 = 8
Direct calculation:
2(7 - 3) = 2(4) = 8
Again, both methods lead to the same result.
Example 4: Expressions with Multiple Terms
Simplify the expression: 4(2x + 3y - 1)
Applying the distributive property to each term:
4(2x + 3y - 1) = 4(2x) + 4(3y) - 4(1) = 8x + 12y - 4
This demonstrates that the distributive property works with expressions containing multiple terms.
Example 5: Distributive Property with Fractions
Simplify the expression: ½(6x + 8)
Applying the distributive property:
½(6x + 8) = ½(6x) + ½(8) = 3x + 4
This showcases the distributive property's applicability to fractions.
Example 6: Distributive Property with Negative Numbers
Simplify the expression: -3(4x - 5)
Applying the distributive property, remembering to handle negative signs carefully:
-3(4x - 5) = -3(4x) - (-3)(5) = -12x + 15
Note how the product of two negative numbers results in a positive number.
Example 7: Factoring Using the Distributive Property
The distributive property is also invaluable for factoring expressions. For example, consider the expression 6x + 12. We can factor out the common factor of 6:
6x + 12 = 6(x + 2)
This is the reverse application of the distributive property, demonstrating its versatility.
Advanced Applications of the Distributive Property
The distributive property extends beyond basic algebraic simplification. It's a cornerstone in:
-
Solving Equations: The distributive property is often necessary to simplify equations before solving for the unknown variable.
-
Polynomial Multiplication: Multiplying polynomials relies heavily on the distributive property (often referred to as the FOIL method – First, Outer, Inner, Last – when multiplying binomials).
-
Calculus: The distributive property is applied in differentiation and integration, particularly when dealing with functions involving sums or differences.
-
Linear Algebra: The distributive property plays a role in matrix operations and vector calculations.
Common Mistakes to Avoid
While the distributive property is straightforward, some common errors can arise:
-
Forgetting to distribute to all terms: Ensure that the multiplier is distributed to every term within the parentheses.
-
Incorrectly handling signs: Pay close attention to the signs of the terms, especially when dealing with negative numbers.
-
Confusing distribution with combining like terms: Remember that the distributive property is about multiplication, not addition or subtraction. Combining like terms occurs after the distribution is complete.
Practice Problems
To reinforce your understanding, try these practice problems:
- 7(x + 3)
- -2(5y - 4)
- 1/3(9a + 6b - 3)
- (x + 2)(x + 5) (Hint: Use the FOIL method)
- Factor: 15x - 20
Conclusion
The distributive property of multiplication over addition is a fundamental principle in mathematics with far-reaching applications. Mastering this concept is essential for success in algebra and beyond. By practicing consistently and carefully avoiding common errors, you can confidently utilize this crucial tool to simplify expressions, solve equations, and tackle more complex mathematical problems. Remember the visual examples and the step-by-step approaches outlined here to solidify your understanding. Through continuous practice and application, the distributive property will become second nature.
Latest Posts
Latest Posts
-
What Is The Prime Factor Of 300
Apr 08, 2025
-
10 To The Power Of Negative 7
Apr 08, 2025
-
How Do You Write 0 16 As A Fraction
Apr 08, 2025
-
What Is The Square Root Of 1156
Apr 08, 2025
-
How Do You Spell The Word 20
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about Distributive Property Of Multiplication Over Addition Example . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.