Fill In The Ratio Table For 31
News Co
Apr 02, 2025 · 6 min read
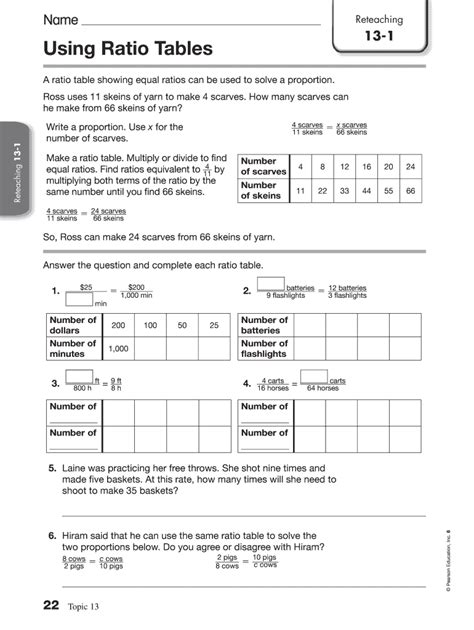
Table of Contents
Filling in Ratio Tables: A Comprehensive Guide for 31 and Beyond
Ratio tables are fundamental tools in mathematics, used to represent and solve problems involving proportional relationships. Understanding how to complete these tables is crucial for success in various mathematical applications, from simple fraction problems to complex algebraic equations. This comprehensive guide will delve into the intricacies of filling ratio tables, focusing specifically on the number 31, while providing a framework applicable to any ratio. We'll explore different methods, highlight common pitfalls, and offer practical examples to solidify your understanding.
Understanding Ratios and Ratio Tables
A ratio is a comparison of two or more quantities. It expresses the relative size of one quantity to another. Ratios can be expressed in various ways: using a colon (e.g., 3:1), as a fraction (e.g., 3/1), or using the word "to" (e.g., 3 to 1). A ratio table is a visual representation of a ratio, organizing the relationship between the quantities involved in a systematic manner. This structure allows for easy scaling and the determination of equivalent ratios.
Key Concepts:
- Equivalent Ratios: Ratios that express the same proportional relationship. For example, 3:1, 6:2, and 9:3 are all equivalent ratios.
- Scaling: The process of multiplying or dividing both parts of a ratio by the same number to obtain an equivalent ratio. This is the core operation in completing ratio tables.
- Simplifying Ratios: Reducing a ratio to its simplest form by dividing both parts by their greatest common divisor. This makes the ratio easier to understand and work with.
Filling in Ratio Tables: Methods and Strategies
Let's assume we have a base ratio of 31:x, where x represents an unknown quantity. Our goal is to determine the value of x and populate the ratio table with equivalent ratios. There are several methods we can employ:
Method 1: Using Equivalent Fractions
This method relies on the principle that equivalent ratios can be expressed as equivalent fractions. If we know one part of the ratio and the relationship between the parts, we can set up a proportion and solve for the unknown.
Example:
Let's say we have a ratio of 31:x, and we know that this ratio is equivalent to 62:12. We can set up a proportion:
31/x = 62/12
To solve for x, we can cross-multiply:
31 * 12 = 62 * x
372 = 62x
x = 372/62 = 6
Therefore, the complete ratio is 31:6. We can extend this to create a table of equivalent ratios.
| Ratio 1 | Ratio 2 | Ratio 3 | Ratio 4 |
|---|---|---|---|
| 31:6 | 62:12 | 93:18 | 124:24 |
Method 2: Using Scaling Factors
This approach focuses on finding a scaling factor that relates one ratio to another. If we know one ratio and need to find an equivalent ratio, we can determine the scaling factor by dividing the corresponding parts of the ratios.
Example:
Suppose we have the ratio 31:6. We want to find an equivalent ratio where the first part is 155. We can determine the scaling factor:
155 / 31 = 5
We then multiply the second part of the original ratio by the scaling factor:
6 * 5 = 30
Therefore, the equivalent ratio is 155:30.
| Ratio 1 | Ratio 2 | Ratio 3 |
|---|---|---|
| 31:6 | 62:12 | 155:30 |
Method 3: Using Unit Rate
The unit rate is the ratio expressed as a rate per one unit. Finding the unit rate simplifies the process of generating equivalent ratios. To find the unit rate, divide both parts of the ratio by the value of one of the parts (usually the first).
Example:
Let's say we have the ratio 31:x and we know that for every 31 units of one quantity, there are 6 units of another quantity. The unit rate is found by dividing both parts by 31:
31/31 : 6/31 ≈ 1 : 0.19
This tells us that for every 1 unit of the first quantity, there are approximately 0.19 units of the second quantity. We can use this unit rate to easily generate other equivalent ratios by multiplying both parts by different factors.
| Ratio 1 | Ratio 2 | Ratio 3 |
|---|---|---|
| 31:6 | 62:12 | 93:18 |
Advanced Techniques and Applications
The methods described above can be applied to solve more complex ratio problems.
Solving Word Problems:
Ratio tables are powerful tools for solving real-world problems involving proportional relationships. The key is to correctly identify the ratio and then use the appropriate method to fill in the table and solve for the unknown quantity.
Example:
A recipe calls for 31 grams of flour for every 6 grams of sugar. If you want to make a larger batch using 93 grams of flour, how much sugar will you need?
- Identify the ratio: 31 grams of flour : 6 grams of sugar
- Set up the table:
| Flour (grams) | Sugar (grams) |
|---|---|
| 31 | 6 |
| 93 | x |
- Solve for x: Since 93 is 3 times 31, we multiply the sugar amount by 3: 6 * 3 = 18 grams of sugar.
Ratios with Three or More Quantities:
The principles of ratio tables extend to ratios involving more than two quantities. The methods remain the same, but you will have more columns in your table to manage the additional quantities.
Handling Decimals and Fractions:
The methods work equally well with decimal and fractional ratios. You might need a calculator to perform the calculations more efficiently.
Common Mistakes and How to Avoid Them
- Incorrect Cross-Multiplication: Carefully ensure you cross-multiply correctly when solving proportions.
- Misinterpreting the Ratio: Clearly define which quantity corresponds to which part of the ratio.
- Ignoring Units: Pay close attention to the units of measurement and ensure consistency throughout the problem.
- Rounding Errors: If you are working with decimals, be mindful of rounding errors and their potential impact on the final answer.
Conclusion: Mastering Ratio Tables
Understanding and mastering ratio tables is a cornerstone of mathematical proficiency. The ability to effectively manipulate and interpret ratios is essential for solving a wide range of problems across various disciplines. This guide has provided a comprehensive overview of the techniques involved, along with practical examples and common pitfalls to avoid. By consistently practicing these methods and focusing on the underlying concepts, you will develop a robust understanding of ratios and confidently apply them to solve any problem you encounter. Remember that practice is key; the more ratio tables you complete, the more comfortable and efficient you'll become. Start with simpler ratios and gradually work your way up to more complex scenarios. The journey to mastering ratio tables might seem challenging at first, but with dedication and persistent effort, you will successfully navigate the world of proportional relationships.
Latest Posts
Related Post
Thank you for visiting our website which covers about Fill In The Ratio Table For 31 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.