Find Exact Value Of Cos Pi 12
News Co
May 08, 2025 · 4 min read
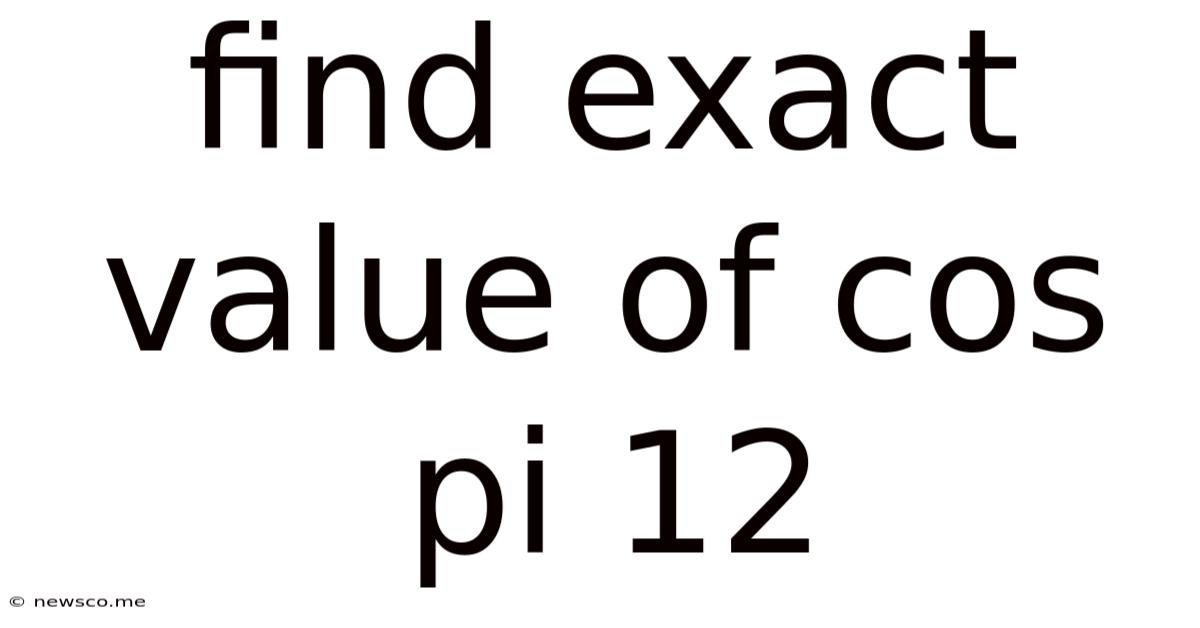
Table of Contents
Finding the Exact Value of cos(π/12): A Comprehensive Guide
Determining the exact value of trigonometric functions for angles that aren't standard (like 30°, 45°, 60°) often requires using trigonometric identities and a bit of clever manipulation. Finding the exact value of cos(π/12) is a classic example of this. This article will guide you through several methods, explaining the underlying principles and providing a thorough understanding of how to solve this problem and similar ones.
Understanding the Angle π/12
Before diving into the calculations, it's essential to understand what π/12 represents. Recall that π radians is equivalent to 180°. Therefore, π/12 radians is equivalent to (180°/12) = 15°. Our goal is to find the exact value of cos(15°).
Method 1: Using the Difference Formula for Cosine
This is perhaps the most common and straightforward method. We can express 15° as the difference between two angles whose cosine values we know: 45° and 30°.
Specifically, 15° = 45° - 30°. We can then utilize the cosine difference formula:
cos(A - B) = cos A cos B + sin A sin B
Let A = 45° and B = 30°. Substituting these values into the formula, we get:
cos(15°) = cos(45° - 30°) = cos(45°)cos(30°) + sin(45°)sin(30°)
Now, we substitute the known values:
- cos(45°) = √2/2
- cos(30°) = √3/2
- sin(45°) = √2/2
- sin(30°) = 1/2
Therefore:
cos(15°) = (√2/2)(√3/2) + (√2/2)(1/2) = (√6 + √2) / 4
Therefore, the exact value of cos(π/12) is (√6 + √2) / 4.
Method 2: Using the Half-Angle Formula for Cosine
Another effective approach involves using the half-angle formula for cosine. Since 15° is half of 30°, we can apply the formula:
cos(θ/2) = ±√[(1 + cos θ) / 2]
In our case, θ = 30°, so θ/2 = 15°. Since 15° lies in the first quadrant, where cosine is positive, we use the positive square root:
cos(15°) = √[(1 + cos 30°) / 2]
Substituting the value of cos(30°) = √3/2:
cos(15°) = √[(1 + √3/2) / 2] = √[(2 + √3) / 4] = √(2 + √3) / 2
This expression might seem different from the result obtained using the difference formula. However, let's simplify it further:
Square the expression: [(√(2 + √3)) / 2]² = (2 + √3) / 4
Now, let's rationalize the denominator of the original result from Method 1:
[(√6 + √2) / 4]² = (6 + 2√12 + 2) / 16 = (8 + 4√3) / 16 = (2 + √3) / 4
This confirms both methods yield the same result, albeit in slightly different forms.
Method 3: Using the Sum-to-Product Formula
While less intuitive for this specific problem, the sum-to-product formulas can also be used. However, this method requires a bit more manipulation and isn't as straightforward as the previous two methods. It's primarily included for completeness and to showcase the versatility of trigonometric identities. This method requires expressing 15° as a sum of angles, which would involve fractions, making the calculation lengthier. Therefore, this approach is less efficient than the previous two methods.
Verifying the Result using a Calculator
While we've derived the exact value, it's always good practice to verify the result using a calculator. Make sure your calculator is set to radians mode, then calculate cos(π/12). The approximate decimal value should match the approximate decimal value of (√6 + √2) / 4. This serves as a valuable check to ensure our calculations are correct.
Applications of cos(π/12)
The exact value of cos(π/12) isn't just an academic exercise. It has applications in various fields, including:
- Geometry: Solving triangles and finding the lengths of sides and angles in various geometric shapes.
- Physics: Analyzing oscillatory motion and wave phenomena, where trigonometric functions are crucial.
- Engineering: Designing structures, analyzing forces, and performing calculations involving angles.
- Computer Graphics: Generating realistic images and animations. Precise trigonometric calculations are essential for creating accurate representations of shapes and movements.
Expanding on Trigonometric Identities
Mastering the exact values of trigonometric functions for various angles relies heavily on understanding and skillfully applying trigonometric identities. These identities aren't just formulas; they are powerful tools that allow for simplification and manipulation of expressions. Familiarize yourself with these key identities:
- Pythagorean Identities: sin²θ + cos²θ = 1; 1 + tan²θ = sec²θ; 1 + cot²θ = csc²θ
- Sum and Difference Formulas: for sin, cos, and tan
- Double-Angle Formulas: for sin, cos, and tan
- Half-Angle Formulas: for sin, cos, and tan
- Product-to-Sum and Sum-to-Product Formulas: useful for transforming expressions.
Practicing with these identities will improve your ability to solve trigonometric problems efficiently and accurately.
Conclusion: A Deeper Understanding of Trigonometric Functions
Finding the exact value of cos(π/12) demonstrates the importance of understanding and applying trigonometric identities. The ability to manipulate these identities is crucial for simplifying complex expressions and arriving at precise solutions. By mastering these techniques, you'll enhance your problem-solving skills in mathematics and related fields. Remember to always check your work and verify your results using a calculator to ensure accuracy. The methods outlined in this article, along with a firm understanding of trigonometric identities, will equip you to tackle similar problems confidently. The journey of mastering trigonometry is a continuous process of learning and applying these fundamental concepts. Consistent practice will further solidify your understanding and enhance your abilities.
Latest Posts
Latest Posts
-
What Is The Product Of 9 And 12
May 08, 2025
-
Find The Sine Of An Angle
May 08, 2025
-
How Do You Write A Quarter Inch
May 08, 2025
-
What Are The Numbers Called In A Division Problem
May 08, 2025
-
How To Find Value Of X When Y Is Given
May 08, 2025
Related Post
Thank you for visiting our website which covers about Find Exact Value Of Cos Pi 12 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.