Formula For Standard Deviation Of Grouped Data
News Co
Apr 05, 2025 · 6 min read
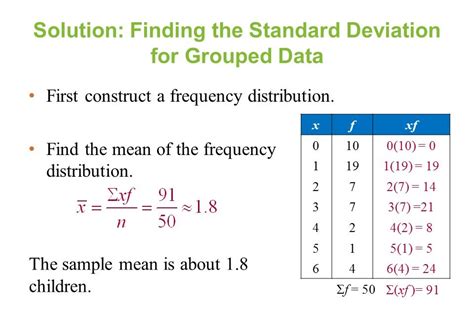
Table of Contents
Formula for Standard Deviation of Grouped Data: A Comprehensive Guide
Understanding standard deviation is crucial for analyzing the spread or dispersion of data. While calculating the standard deviation for ungrouped data is relatively straightforward, dealing with grouped data requires a slightly different approach. This comprehensive guide delves into the formula for the standard deviation of grouped data, explaining the concepts, steps, and providing practical examples. We'll also explore the nuances and limitations of this method.
What is Grouped Data?
Before diving into the formula, let's define grouped data. Grouped data refers to data that has been organized into intervals or classes. Instead of listing each individual data point, we summarize the data by indicating the number of observations falling within specific ranges. This is particularly useful when dealing with large datasets or continuous data. For example, instead of listing the exact age of every participant in a study, we might group them into age ranges (e.g., 20-29, 30-39, 40-49).
Why Use Grouped Data?
There are several compelling reasons for using grouped data:
- Simplified data representation: Large datasets can be overwhelming to analyze directly. Grouping condenses the information, making it easier to manage and understand.
- Improved data visualization: Grouped data is ideal for creating histograms and frequency distributions, providing a clearer visual representation of the data's distribution.
- Enhanced data analysis: Calculating summary statistics like mean and standard deviation becomes more manageable with grouped data, especially for datasets with a wide range of values.
Understanding Standard Deviation
Standard deviation measures the average distance of each data point from the mean. A larger standard deviation signifies greater dispersion, while a smaller standard deviation indicates data points are clustered closely around the mean. It's a vital tool for understanding data variability and making informed decisions.
Formula for Standard Deviation of Grouped Data
The formula for the standard deviation of grouped data is slightly more complex than for ungrouped data. It utilizes the concept of class midpoints and frequencies. Here's the breakdown:
1. Calculate the midpoint of each class interval:
The midpoint (x<sub>i</sub>) is the average of the upper and lower class limits of each interval. For example, if an interval is 10-19, the midpoint is (10+19)/2 = 14.5.
2. Calculate the mean (x̄) of the grouped data:
The formula for the mean of grouped data is:
x̄ = Σ(f<sub>i</sub> * x<sub>i</sub>) / Σf<sub>i</sub>
Where:
- f<sub>i</sub> = frequency of the i<sup>th</sup> class interval
- x<sub>i</sub> = midpoint of the i<sup>th</sup> class interval
- Σf<sub>i</sub> = total number of observations (sum of frequencies)
3. Calculate the variance (σ²) of the grouped data:
The formula for the variance of grouped data is:
σ² = Σ[f<sub>i</sub> * (x<sub>i</sub> - x̄)²] / Σf<sub>i</sub>
Where:
- f<sub>i</sub> = frequency of the i<sup>th</sup> class interval
- x<sub>i</sub> = midpoint of the i<sup>th</sup> class interval
- x̄ = mean of the grouped data
- Σf<sub>i</sub> = total number of observations (sum of frequencies)
4. Calculate the standard deviation (σ) of the grouped data:
The standard deviation is simply the square root of the variance:
σ = √σ²
Step-by-Step Calculation Example
Let's illustrate the calculation with a practical example. Consider the following data representing the weights (in kg) of 50 students:
| Weight (kg) | Frequency (f<sub>i</sub>) |
|---|---|
| 40-45 | 4 |
| 45-50 | 8 |
| 50-55 | 15 |
| 55-60 | 12 |
| 60-65 | 7 |
| 65-70 | 4 |
Step 1: Calculate the midpoints (x<sub>i</sub>):
| Weight (kg) | Frequency (f<sub>i</sub>) | Midpoint (x<sub>i</sub>) |
|---|---|---|
| 40-45 | 4 | 42.5 |
| 45-50 | 8 | 47.5 |
| 50-55 | 15 | 52.5 |
| 55-60 | 12 | 57.5 |
| 60-65 | 7 | 62.5 |
| 65-70 | 4 | 67.5 |
Step 2: Calculate the mean (x̄):
Σ(f<sub>i</sub> * x<sub>i</sub>) = (4 * 42.5) + (8 * 47.5) + (15 * 52.5) + (12 * 57.5) + (7 * 62.5) + (4 * 67.5) = 2625
Σf<sub>i</sub> = 50
x̄ = 2625 / 50 = 52.5 kg
Step 3: Calculate the variance (σ²):
We need to calculate (x<sub>i</sub> - x̄)² for each class:
| Weight (kg) | f<sub>i</sub> | x<sub>i</sub> | (x<sub>i</sub> - x̄) | (x<sub>i</sub> - x̄)² | f<sub>i</sub> * (x<sub>i</sub> - x̄)² |
|---|---|---|---|---|---|
| 40-45 | 4 | 42.5 | -10 | 100 | 400 |
| 45-50 | 8 | 47.5 | -5 | 25 | 200 |
| 50-55 | 15 | 52.5 | 0 | 0 | 0 |
| 55-60 | 12 | 57.5 | 5 | 25 | 300 |
| 60-65 | 7 | 62.5 | 10 | 100 | 700 |
| 65-70 | 4 | 67.5 | 15 | 225 | 900 |
Σ[f<sub>i</sub> * (x<sub>i</sub> - x̄)²] = 2500
σ² = 2500 / 50 = 50
Step 4: Calculate the standard deviation (σ):
σ = √50 ≈ 7.07 kg
Therefore, the standard deviation of the students' weights is approximately 7.07 kg. This indicates a moderate spread in the weight distribution.
Interpreting the Standard Deviation
The standard deviation provides valuable insights into data variability:
- Low standard deviation: Indicates data points are clustered tightly around the mean, suggesting low variability.
- High standard deviation: Indicates data points are widely dispersed from the mean, suggesting high variability.
In our example, a standard deviation of 7.07 kg suggests a moderate level of variability in students' weights.
Limitations of the Formula
It's essential to acknowledge the limitations of using this formula:
- Assumption of uniform distribution within each class: The formula assumes that data points within each interval are uniformly distributed. This might not always be the case in reality, leading to slight inaccuracies.
- Loss of precision: Grouping data inherently involves a loss of precision, as individual data points are not considered separately.
- Sensitivity to class intervals: The choice of class intervals can impact the calculated standard deviation. Using inappropriate intervals might lead to misleading results.
Alternative Methods and Considerations
While the formula provides a reasonable estimate, other methods might be more appropriate depending on the data and the research question:
- Using software: Statistical software packages offer more sophisticated methods for calculating standard deviation, handling potential biases and providing more accurate results.
- Considering data transformations: If the data is significantly skewed, transforming the data (e.g., using logarithmic transformations) before calculating the standard deviation might be beneficial.
- Using different measures of dispersion: While standard deviation is a common measure, other measures like the interquartile range might be more robust to outliers and skewed data.
Conclusion
Calculating the standard deviation of grouped data is a valuable technique for analyzing the spread of data in large or continuous datasets. While the formula provides a useful estimate, it's crucial to be aware of its limitations and consider alternative methods if necessary. By understanding the formula, its interpretations, and limitations, researchers and analysts can make more informed decisions based on their data analysis. Remember that the accuracy of the results depends heavily on the proper selection of class intervals and an understanding of the underlying data distribution. Always consider the context and potential biases when interpreting the standard deviation. Further research and exploration of alternative statistical methods can lead to more robust and reliable conclusions.
Latest Posts
Related Post
Thank you for visiting our website which covers about Formula For Standard Deviation Of Grouped Data . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.