Formula For The Perimeter Of A Hexagon
News Co
Apr 05, 2025 · 5 min read
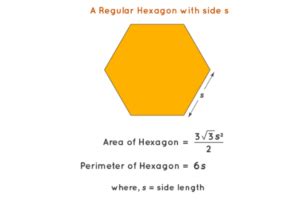
Table of Contents
The Complete Guide to the Perimeter of a Hexagon: Formulas, Calculations, and Applications
The hexagon, a six-sided polygon, holds a fascinating place in geometry and its applications span various fields, from architecture to nature. Understanding its perimeter is fundamental to many calculations and designs. This comprehensive guide will delve into the formulas for calculating the perimeter of a hexagon, explore different types of hexagons, and provide practical examples to solidify your understanding.
What is a Hexagon?
Before we dive into the formulas, let's establish a clear understanding of what a hexagon is. A hexagon is a polygon with six sides and six angles. The sum of the interior angles of any hexagon is always 720 degrees. However, not all hexagons are created equal. They can be classified into different types, each with its own characteristics.
Types of Hexagons:
-
Regular Hexagon: This is the most commonly discussed type. A regular hexagon has all six sides of equal length and all six angles equal to 120 degrees. Its symmetrical nature simplifies perimeter calculations significantly.
-
Irregular Hexagon: An irregular hexagon has sides of varying lengths and angles of varying measures. Calculating the perimeter of an irregular hexagon requires knowing the length of each side individually.
-
Convex Hexagon: In a convex hexagon, all interior angles are less than 180 degrees. This means that all interior angles are "pointing outwards."
-
Concave Hexagon: A concave hexagon has at least one interior angle greater than 180 degrees. This means at least one interior angle "points inwards."
Formulas for Calculating the Perimeter of a Hexagon
The formula used to calculate the perimeter depends on the type of hexagon.
Perimeter of a Regular Hexagon:
The simplest formula applies to regular hexagons. Since all sides are equal, the perimeter is simply the length of one side multiplied by six.
Formula: Perimeter = 6 * s
Where 's' represents the length of one side of the regular hexagon.
Example: If a regular hexagon has a side length of 5 cm, its perimeter is 6 * 5 cm = 30 cm.
Perimeter of an Irregular Hexagon:
Calculating the perimeter of an irregular hexagon is straightforward but requires more information. You must know the length of each individual side.
Formula: Perimeter = a + b + c + d + e + f
Where 'a', 'b', 'c', 'd', 'e', and 'f' represent the lengths of each of the six sides.
Example: If an irregular hexagon has sides measuring 2 cm, 3 cm, 4 cm, 5 cm, 6 cm, and 7 cm, its perimeter is 2 + 3 + 4 + 5 + 6 + 7 cm = 27 cm.
Advanced Concepts and Applications
Beyond the basic formulas, understanding the relationships between a hexagon's perimeter, area, and other properties can lead to more complex calculations and applications.
Relationship between Perimeter and Area of a Regular Hexagon:
The area of a regular hexagon can be calculated using its side length. This relationship is useful in various applications, particularly in engineering and design. The formula for the area (A) of a regular hexagon is:
Formula: A = (3√3/2) * s²
Where 's' is the side length. Notice that the area calculation depends directly on the side length, and therefore indirectly on the perimeter (since the perimeter is 6s).
Hexagons in Nature and Architecture:
Hexagons are frequently found in nature, most notably in honeycombs constructed by bees. The hexagonal shape allows for efficient use of space and materials. This natural occurrence demonstrates the optimization inherent in the hexagon's geometry. In architecture, hexagonal shapes are used in various designs, ranging from floor tiles to building structures, often chosen for their aesthetic appeal and structural integrity.
Calculating the Perimeter Using Coordinates:
If you have the coordinates of the vertices of an irregular hexagon, you can calculate the distance between each pair of consecutive vertices using the distance formula. The sum of these distances will give you the perimeter.
Distance Formula: d = √[(x₂ - x₁)² + (y₂ - y₁)²]
Where (x₁, y₁) and (x₂, y₂) are the coordinates of two points. You would apply this formula six times, once for each side of the hexagon, and then sum the results to find the perimeter.
Practical Problems and Solutions:
Let's work through some practical examples to solidify your understanding.
Problem 1: A regular hexagon-shaped garden has a side length of 2 meters. What is its perimeter?
Solution: Using the formula for the perimeter of a regular hexagon: Perimeter = 6 * s = 6 * 2 meters = 12 meters.
Problem 2: A hexagonal tile has sides measuring 3 cm, 4 cm, 5 cm, 3 cm, 4 cm, and 5 cm. What is its perimeter?
Solution: This is an irregular hexagon. The perimeter is the sum of all its sides: Perimeter = 3 + 4 + 5 + 3 + 4 + 5 cm = 24 cm.
Problem 3: A beehive has a honeycomb cell shaped like a regular hexagon with an area of 10 square centimeters. What is the approximate perimeter of the cell?
Solution: We need to use the area formula to find the side length first: 10 = (3√3/2) * s². Solving for s gives us approximately s ≈ 2 cm. Then, we use the perimeter formula for a regular hexagon: Perimeter = 6 * s = 6 * 2 cm ≈ 12 cm.
Conclusion:
Understanding the perimeter of a hexagon is crucial in various fields, from simple geometry problems to complex architectural designs and natural observations. Whether it's a regular or irregular hexagon, applying the appropriate formula and understanding the underlying principles will help you accurately calculate its perimeter and solve various related problems. Remember to consider the specific type of hexagon and the information provided when choosing the correct formula. Mastering these concepts allows for a deeper appreciation of the hexagon's geometric properties and its significant presence in the world around us. By understanding the concepts presented here, you can confidently tackle any hexagon-related perimeter calculation you may encounter.
Latest Posts
Related Post
Thank you for visiting our website which covers about Formula For The Perimeter Of A Hexagon . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.