Geometry Parallel And Perpendicular Lines Worksheet
News Co
Apr 04, 2025 · 7 min read
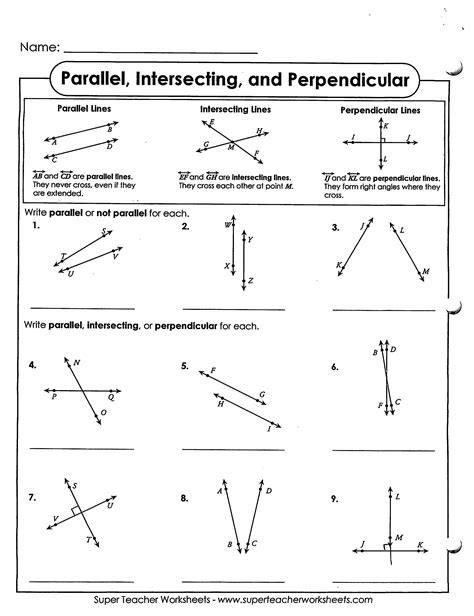
Table of Contents
Geometry: Parallel and Perpendicular Lines Worksheet – A Comprehensive Guide
This comprehensive guide delves into the world of parallel and perpendicular lines, providing a detailed explanation of their properties, relationships, and applications. We'll explore various methods for identifying and working with these lines, using examples and exercises to solidify your understanding. This guide serves as a complete resource for anyone working through a geometry worksheet focused on parallel and perpendicular lines, providing solutions and deeper insights beyond the typical worksheet problems.
Understanding Parallel Lines
Parallel lines are two or more lines that lie in the same plane and never intersect, no matter how far they are extended. Think of train tracks – they are a perfect example of parallel lines.
Key Properties of Parallel Lines:
- No Intersection: The defining characteristic of parallel lines is that they never meet.
- Same Slope (for lines on a coordinate plane): In coordinate geometry, parallel lines have the same slope. This is a crucial concept for identifying parallel lines when given their equations.
- Equal Corresponding Angles: When a transversal line intersects two parallel lines, several pairs of angles are formed. Corresponding angles are angles that occupy the same relative position at an intersection, and they are always equal in measure.
- Equal Alternate Interior Angles: Alternate interior angles are angles that lie on opposite sides of the transversal and inside the parallel lines. These angles are also always equal in measure.
- Supplementary Consecutive Interior Angles: Consecutive interior angles lie on the same side of the transversal and inside the parallel lines. These angles are supplementary, meaning their sum is 180 degrees.
Identifying Parallel Lines:
-
Visual Inspection: For lines drawn on a diagram, visual inspection can often be enough to determine if they are parallel. Look for lines that maintain a consistent distance from each other.
-
Slope Comparison: If you're given the equations of two lines in slope-intercept form (y = mx + b), where 'm' represents the slope, then the lines are parallel if and only if they have the same slope (m1 = m2).
-
Using Transversals and Angle Relationships: If a transversal line intersects two other lines, and the corresponding angles, alternate interior angles, or alternate exterior angles are equal, then the two lines are parallel. This principle is often used in proofs and problem-solving.
Understanding Perpendicular Lines
Perpendicular lines are two lines that intersect at a right angle (90 degrees). The corner of a square or rectangle perfectly illustrates perpendicular lines.
Key Properties of Perpendicular Lines:
- Right Angle Intersection: The defining characteristic is the 90-degree intersection.
- Negative Reciprocal Slopes (for lines on a coordinate plane): In coordinate geometry, if two lines are perpendicular, their slopes are negative reciprocals of each other. That is, if the slope of one line is 'm', then the slope of the perpendicular line is '-1/m'.
- Formation of Right Angles: The intersection always creates four right angles (90 degrees each).
Identifying Perpendicular Lines:
-
Visual Inspection: Similar to parallel lines, visual inspection can often suffice for determining perpendicularity. Look for lines that intersect at a clear right angle.
-
Slope Comparison: If given the equations of two lines, check if their slopes are negative reciprocals. For example, if one line has a slope of 2, a perpendicular line will have a slope of -1/2.
-
Using Geometric Properties: In geometric constructions and proofs, perpendicular lines often play a crucial role in establishing specific properties of shapes and figures.
Solving Problems with Parallel and Perpendicular Lines
Let's illustrate how to solve problems involving parallel and perpendicular lines using examples.
Example 1: Finding the Slope of a Parallel Line
Given a line with equation y = 3x + 2, find the slope of a line parallel to it.
Solution:
Parallel lines have the same slope. The slope of the given line is 3. Therefore, the slope of any line parallel to it is also 3.
Example 2: Finding the Slope of a Perpendicular Line
Given a line with equation y = -2/5x + 7, find the slope of a line perpendicular to it.
Solution:
Perpendicular lines have slopes that are negative reciprocals of each other. The slope of the given line is -2/5. The negative reciprocal is 5/2. Therefore, the slope of a line perpendicular to it is 5/2.
Example 3: Determining Parallelism using Transversals
Two lines are intersected by a transversal. One pair of consecutive interior angles measures 110° and 70°. Are the lines parallel?
Solution:
Consecutive interior angles must be supplementary (add up to 180°) for lines to be parallel. 110° + 70° = 180°. Therefore, the lines are parallel.
Example 4: Determining Perpendicularity using Slopes
Two lines have equations y = 2x + 1 and y = -1/2x - 3. Are they perpendicular?
Solution:
The slopes are 2 and -1/2. These are negative reciprocals of each other (2 * (-1/2) = -1). Therefore, the lines are perpendicular.
Advanced Concepts and Applications
This section explores more advanced concepts related to parallel and perpendicular lines.
Parallel and Perpendicular Lines in Three-Dimensional Space
In three-dimensional space, the concepts of parallelism and perpendicularity extend to planes and lines in space. Lines can be parallel, intersecting, or skew (neither parallel nor intersecting). Planes can be parallel or intersect in a line. A line can be perpendicular to a plane, meaning it's perpendicular to every line in that plane that passes through the point of intersection.
Parallel Postulates and Euclidean Geometry
The concept of parallel lines is foundational in Euclidean geometry. Euclid's fifth postulate (the parallel postulate) states that given a line and a point not on the line, there exists exactly one line through the point that is parallel to the given line. This postulate has significant implications for the overall structure and properties of Euclidean geometry.
Applications in Various Fields
Understanding parallel and perpendicular lines is crucial in various fields including:
- Architecture and Engineering: Used in designing buildings, bridges, and other structures to ensure stability and functionality.
- Computer Graphics: Essential for creating 2D and 3D graphics and animations.
- Cartography: Used in creating maps and projections.
- Physics: Used in analyzing forces and motion.
- Computer Programming: Fundamental concepts in game development and other applications involving spatial reasoning.
Geometry Worksheet Practice Problems:
Here are some practice problems to solidify your understanding:
-
Find the slope of a line parallel to the line y = -4x + 7.
-
Find the slope of a line perpendicular to the line y = 1/3x - 2.
-
Two lines are intersected by a transversal. A pair of alternate interior angles measures 65° and x°. If the lines are parallel, what is the value of x?
-
Two lines have equations y = 5x + 2 and y = -1/5x – 1. Are these lines parallel, perpendicular, or neither?
-
A line passes through points (2, 3) and (4, 7). Another line passes through points (1, 0) and (3, 4). Are these lines parallel or perpendicular? (Hint: Find the slopes first.)
-
In a diagram, two parallel lines are intersected by a transversal. If one of the angles is 130 degrees, find the measures of all the other angles formed by the intersection.
-
Explain the difference between parallel and perpendicular lines, and give real-world examples of each.
-
If two lines have the same y-intercept but different slopes, are they parallel or perpendicular? Explain.
These problems offer a range of difficulties, from straightforward slope calculations to more complex problems involving transversals and angle relationships. Solving these problems will not only improve your understanding of parallel and perpendicular lines but will also enhance your overall problem-solving skills in geometry. Remember to clearly show your work and explain your reasoning for each problem. This thorough approach is vital for mastering these fundamental geometric concepts. By working through these examples and practice problems, you will build a strong foundation in understanding and applying the principles of parallel and perpendicular lines. This mastery will be invaluable as you continue your studies in geometry and related fields.
Latest Posts
Related Post
Thank you for visiting our website which covers about Geometry Parallel And Perpendicular Lines Worksheet . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.