Half Of 2 3/4 In Fraction
News Co
May 08, 2025 · 5 min read
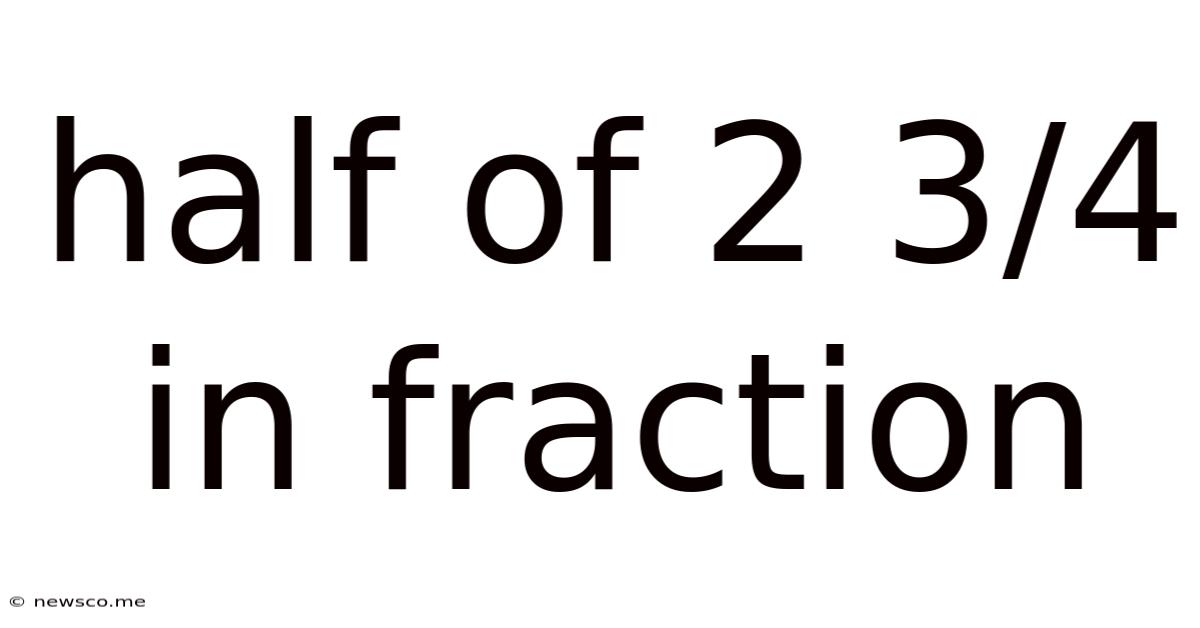
Table of Contents
Half of 2 3/4 in Fraction: A Comprehensive Guide
Finding half of 2 3/4 might seem simple at first glance, but understanding the underlying principles of fraction manipulation is crucial for mastering more complex mathematical concepts. This comprehensive guide will walk you through the process step-by-step, exploring various methods and providing ample practice problems to solidify your understanding. We'll delve into the core concepts, explaining why each step is necessary and offering tips and tricks for efficient calculation. By the end, you'll be confident in calculating half of any mixed number.
Understanding Fractions and Mixed Numbers
Before we dive into calculating half of 2 3/4, let's refresh our understanding of fractions and mixed numbers.
What is a Fraction? A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). The numerator indicates how many parts we have, and the denominator indicates how many equal parts the whole is divided into. For example, in the fraction 3/4, 3 is the numerator and 4 is the denominator. This means we have 3 out of 4 equal parts.
What is a Mixed Number? A mixed number combines a whole number and a proper fraction (a fraction where the numerator is smaller than the denominator). For example, 2 3/4 represents two whole units and three-quarters of another unit.
Method 1: Converting to an Improper Fraction
This is arguably the most straightforward method for finding half of a mixed number. It involves converting the mixed number into an improper fraction (a fraction where the numerator is larger than or equal to the denominator) before performing the division.
Step 1: Convert the mixed number to an improper fraction.
To convert 2 3/4 to an improper fraction, we multiply the whole number (2) by the denominator (4), add the numerator (3), and keep the same denominator (4).
(2 * 4) + 3 = 11
So, 2 3/4 becomes 11/4.
Step 2: Find half of the improper fraction.
To find half of a fraction, we multiply it by 1/2.
(11/4) * (1/2) = 11/8
Step 3: Simplify the fraction (if necessary).
In this case, 11/8 is an improper fraction. We can convert it back to a mixed number by dividing the numerator (11) by the denominator (8).
11 ÷ 8 = 1 with a remainder of 3.
Therefore, 11/8 is equivalent to 1 3/8.
Therefore, half of 2 3/4 is 1 3/8.
Method 2: Finding Half of the Whole Number and the Fraction Separately
This method involves finding half of the whole number part and half of the fractional part separately, then adding the results. This approach can be more intuitive for some learners.
Step 1: Find half of the whole number.
Half of 2 is 1.
Step 2: Find half of the fraction.
Half of 3/4 is (3/4) * (1/2) = 3/8
Step 3: Add the results.
1 + 3/8 = 1 3/8
Therefore, half of 2 3/4 is 1 3/8.
Method 3: Using Decimal Conversion (for Approximation)
While not as precise for fractions, converting to decimals can provide a quick approximation.
Step 1: Convert the mixed number to a decimal.
2 3/4 is equal to 2.75 (because 3/4 = 0.75).
Step 2: Find half of the decimal.
Half of 2.75 is 2.75 / 2 = 1.375
Step 3: Convert the decimal back to a fraction (if needed).
1.375 can be converted back to a fraction by recognizing that 0.375 is 3/8. Therefore, 1.375 is equal to 1 3/8.
Therefore, half of 2 3/4 is approximately 1.375, or 1 3/8. Note that this method is less precise than the other methods due to rounding potential.
Practice Problems
Let's solidify your understanding with some practice problems:
- Find half of 3 1/2.
- What is half of 1 2/5?
- Calculate half of 5 1/3.
- Determine half of 4 3/8.
- Find half of 2 5/6.
Solutions to Practice Problems:
-
Half of 3 1/2: Convert to improper fraction: 7/2. Half of 7/2 is 7/4, which simplifies to 1 3/4.
-
Half of 1 2/5: Convert to improper fraction: 7/5. Half of 7/5 is 7/10.
-
Half of 5 1/3: Convert to improper fraction: 16/3. Half of 16/3 is 8/3, which simplifies to 2 2/3.
-
Half of 4 3/8: Convert to improper fraction: 35/8. Half of 35/8 is 35/16, which simplifies to 2 3/16.
-
Half of 2 5/6: Convert to improper fraction: 17/6. Half of 17/6 is 17/12, which simplifies to 1 5/12.
Conclusion
Finding half of 2 3/4, or any mixed number, involves a fundamental understanding of fraction manipulation. Whether you choose to convert to an improper fraction first, work with the whole and fractional parts separately, or use decimal approximation (for less precise results), the key is to apply the rules of fraction multiplication and simplification consistently. The practice problems provided will help you refine your skills and build confidence in tackling similar problems in the future. Remember to always check your answer for simplification to arrive at the most concise representation of the fraction. Mastering these techniques will form a solid foundation for more advanced mathematical concepts.
Latest Posts
Related Post
Thank you for visiting our website which covers about Half Of 2 3/4 In Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.