How Do You Find The Height Of An Isosceles Triangle
News Co
Apr 04, 2025 · 6 min read
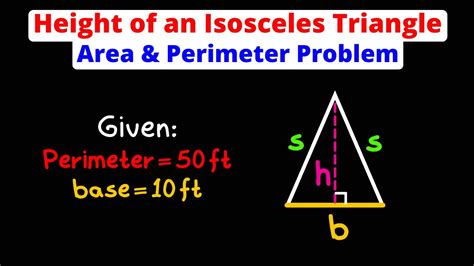
Table of Contents
How to Find the Height of an Isosceles Triangle: A Comprehensive Guide
Finding the height of an isosceles triangle might seem like a simple geometry problem, but understanding the different approaches and when to apply them is crucial. This comprehensive guide will explore multiple methods for calculating the height, catering to various levels of mathematical understanding, from basic geometry to employing trigonometric functions. We'll delve into the nuances of each method, providing clear explanations and practical examples to ensure you master this essential geometric skill.
Understanding Isosceles Triangles and Their Properties
Before we jump into the methods, let's refresh our understanding of isosceles triangles. An isosceles triangle is defined as a triangle with at least two sides of equal length. These equal sides are called legs, and the angle between them is called the apex angle. The side opposite the apex angle is called the base. The height of an isosceles triangle is the perpendicular distance from the apex to the base. This height bisects the base, creating two congruent right-angled triangles. This property is key to many of the methods we'll explore.
Method 1: Using the Pythagorean Theorem (When Base and Leg Lengths are Known)
This is arguably the most straightforward method, applicable when you know the lengths of the base and one of the legs. The Pythagorean theorem states that in a right-angled triangle, the square of the hypotenuse (the longest side) is equal to the sum of the squares of the other two sides (legs).
Steps:
-
Identify the right-angled triangle: The height of the isosceles triangle divides it into two congruent right-angled triangles. The height is one leg, half the base is the other leg, and the leg of the isosceles triangle is the hypotenuse.
-
Apply the Pythagorean theorem: Let 'a' be the length of the leg, 'b' be half the length of the base, and 'h' be the height. Then:
a² = b² + h² -
Solve for h: Rearrange the equation to solve for the height:
h = √(a² - b²)
Example:
Consider an isosceles triangle with a base of 12 cm and legs of 10 cm each.
- Half the base (b) = 12 cm / 2 = 6 cm
- Applying the Pythagorean theorem: 10² = 6² + h²
- Solving for h: h = √(10² - 6²) = √(100 - 36) = √64 = 8 cm
Therefore, the height of the isosceles triangle is 8 cm.
Method 2: Using Trigonometry (When Base and Apex Angle are Known)
Trigonometry provides an elegant solution when the base and the apex angle are given. We'll utilize trigonometric functions such as sine, cosine, and tangent.
Steps:
-
Identify the right-angled triangle: Again, the height divides the isosceles triangle into two congruent right-angled triangles.
-
Apply trigonometric functions: We can use the tangent function, which is defined as the ratio of the opposite side to the adjacent side. In our case:
tan(apex angle/2) = h / b, where 'h' is the height and 'b' is half the base. -
Solve for h: Rearrange the equation to solve for the height:
h = b * tan(apex angle/2)
Example:
Suppose an isosceles triangle has a base of 10 cm and an apex angle of 60 degrees.
- Half the base (b) = 10 cm / 2 = 5 cm
- Applying the tangent function: tan(60°/2) = h / 5
- Solving for h: h = 5 * tan(30°) ≈ 5 * 0.577 ≈ 2.89 cm
Therefore, the height of the isosceles triangle is approximately 2.89 cm. Remember to use the appropriate units (degrees or radians) for your calculator when working with trigonometric functions.
Method 3: Using the Area Formula (When Area and Base are Known)
The area of a triangle is given by the formula: Area = (1/2) * base * height. If the area and base are known, we can easily calculate the height.
Steps:
-
Use the area formula:
Area = (1/2) * base * height -
Solve for h: Rearrange the equation to solve for the height:
h = (2 * Area) / base
Example:
An isosceles triangle has an area of 24 square cm and a base of 8 cm.
- Applying the area formula: 24 = (1/2) * 8 * h
- Solving for h: h = (2 * 24) / 8 = 6 cm
The height of the isosceles triangle is 6 cm.
Method 4: Using Heron's Formula (When All Three Sides are Known)
Heron's formula allows us to calculate the area of a triangle when all three side lengths are known. Once the area is found, we can use Method 3 to determine the height.
Steps:
-
Calculate the semi-perimeter (s):
s = (a + a + b) / 2, where 'a' is the length of the leg and 'b' is the length of the base. -
Apply Heron's formula:
Area = √[s(s - a)(s - a)(s - b)] -
Solve for h using Method 3: Use the calculated area and the base length in the formula
h = (2 * Area) / base
Example:
An isosceles triangle has two legs of 10 cm each and a base of 12 cm.
- Semi-perimeter (s) = (10 + 10 + 12) / 2 = 16 cm
- Heron's formula: Area = √[16(16 - 10)(16 - 10)(16 - 12)] = √[16 * 6 * 6 * 4] = √2304 = 48 square cm
- Solving for h: h = (2 * 48) / 12 = 8 cm
The height of the isosceles triangle is 8 cm.
Choosing the Right Method
The optimal method for finding the height of an isosceles triangle depends on the information provided. Here's a quick summary to guide your choice:
- Base and leg lengths: Use the Pythagorean theorem (Method 1).
- Base and apex angle: Use trigonometry (Method 2).
- Area and base: Use the area formula (Method 3).
- All three side lengths: Use Heron's formula and then the area formula (Method 4).
Advanced Applications and Considerations
The techniques described above are fundamental. However, understanding more advanced concepts can broaden your problem-solving capabilities.
-
Coordinate Geometry: If the vertices of the isosceles triangle are given as coordinates in a Cartesian plane, you can use the distance formula and the slope formula to find the height.
-
Calculus: For more complex scenarios involving curves or changing dimensions, calculus techniques might be necessary.
-
3D Geometry: The concept of height extends to three-dimensional isosceles triangles (tetrahedra) where the height is the perpendicular distance from a vertex to the base plane.
Conclusion
Mastering the calculation of an isosceles triangle's height is a crucial skill in geometry. By understanding the different methods presented in this guide and knowing when to apply each one, you'll be well-equipped to tackle a wide range of geometry problems. Remember to practice regularly, and don't hesitate to explore advanced applications to further enhance your understanding. This comprehensive guide provides a solid foundation for conquering the challenges of isosceles triangle calculations. With practice and a keen eye for the available data, you'll become proficient in determining the height of any isosceles triangle you encounter.
Latest Posts
Related Post
Thank you for visiting our website which covers about How Do You Find The Height Of An Isosceles Triangle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.