How Do You Find The Perimeter Of A Equilateral Triangle
News Co
Apr 08, 2025 · 5 min read
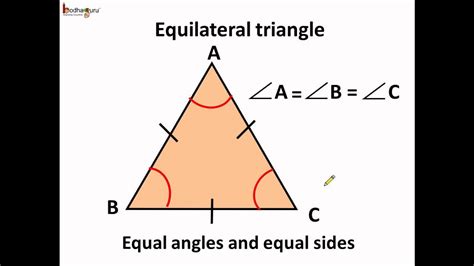
Table of Contents
How Do You Find the Perimeter of an Equilateral Triangle? A Comprehensive Guide
Understanding how to calculate the perimeter of an equilateral triangle is a fundamental concept in geometry. This guide provides a comprehensive walkthrough, exploring various methods and delving into the underlying principles. Whether you're a student brushing up on your geometry skills or simply curious about the topic, this article will equip you with the knowledge and tools to confidently tackle perimeter calculations for equilateral triangles and beyond.
Understanding Equilateral Triangles: The Building Blocks
Before diving into perimeter calculations, let's solidify our understanding of equilateral triangles. An equilateral triangle is a polygon with three equal sides and three equal angles. Each angle in an equilateral triangle always measures 60 degrees. This inherent symmetry is key to simplifying perimeter calculations. Understanding this fundamental property is the first step towards mastering perimeter calculations.
Method 1: The Simple Formula: Side x 3
The most straightforward method for determining the perimeter of an equilateral triangle leverages its defining characteristic: all sides are equal. Therefore, the perimeter is simply three times the length of one side.
Formula: Perimeter = 3 * side
Example: If an equilateral triangle has a side length of 5 cm, its perimeter is 3 * 5 cm = 15 cm.
This method is incredibly efficient and requires minimal calculation. It's the go-to approach when you already know the length of one side. However, real-world scenarios might not always provide this directly. Let's explore situations where we need to find the side length before calculating the perimeter.
Method 2: Using the Area and Height
Sometimes, instead of the side length, you might know the area and height of the equilateral triangle. While seemingly less direct, we can still derive the side length and, consequently, the perimeter.
Understanding the Relationship between Area, Height, and Side
The area of an equilateral triangle can be calculated using the formula:
Area = (√3/4) * side²
The height (altitude) of an equilateral triangle is related to the side length by:
Height = (√3/2) * side
We can manipulate these equations to solve for the side length if we know the area or the height.
Example using Area:
Let's say the area of an equilateral triangle is 25√3 square centimeters. We can set up the equation:
25√3 = (√3/4) * side²
Solving for 'side':
side² = (25√3 * 4) / √3 = 100
side = 10 cm
Therefore, the perimeter is 3 * 10 cm = 30 cm.
Example using Height:
Suppose the height of an equilateral triangle is 10√3 cm. We use the height formula:
10√3 = (√3/2) * side
Solving for 'side':
side = (10√3 * 2) / √3 = 20 cm
Therefore, the perimeter is 3 * 20 cm = 60 cm.
This method highlights the interconnectedness of different geometrical properties within an equilateral triangle. Knowing the area or height opens up a path to calculate the perimeter, demonstrating the versatility of geometric principles.
Method 3: Applying Trigonometry: Using an Angle and One Side
Trigonometry provides another powerful tool for determining the perimeter of an equilateral triangle, especially when dealing with angles and side lengths.
Let's consider a scenario where we know one side and one of the 60° angles. We can use trigonometric functions (sine, cosine, tangent) to find the other sides, which, in an equilateral triangle, are all equal.
Example using Sine:
Imagine we have an equilateral triangle with one side (let's call it 'a') measuring 8 cm. We want to find the perimeter. Since all sides are equal, we only need to find one side. However, we can use sine to verify our result.
We can use the sine rule for any triangle (a/sinA = b/sinB = c/sinC). However, for an equilateral triangle, we know all angles are 60°. Thus, all sides are equal.
Therefore, the perimeter is 3 * 8 cm = 24 cm.
This method showcases the applicability of trigonometry to equilateral triangles, especially beneficial when dealing with problems involving angles and sides in more complex geometric configurations.
Method 4: Using the Circumradius or Inradius
The circumradius (R) is the radius of the circumscribed circle (the circle that passes through all three vertices of the triangle). The inradius (r) is the radius of the inscribed circle (the circle that is tangent to all three sides of the triangle). Both are related to the side length (a) of the equilateral triangle by specific formulas:
- Circumradius: R = a / √3
- Inradius: r = a / (2√3)
Example using Circumradius:
If the circumradius of an equilateral triangle is 5 cm, we can find the side length:
5 = a / √3
a = 5√3 cm
Therefore, the perimeter is 3 * 5√3 cm = 15√3 cm.
Example using Inradius:
If the inradius of an equilateral triangle is 2 cm, we can find the side length:
2 = a / (2√3)
a = 4√3 cm
Therefore, the perimeter is 3 * 4√3 cm = 12√3 cm.
These methods demonstrate the connections between the equilateral triangle's properties and its inscribed and circumscribed circles, offering alternative paths to calculating the perimeter. These formulas are particularly helpful when dealing with problems involving circles and triangles.
Solving Real-World Problems: Applications of Equilateral Triangle Perimeter
The calculation of the perimeter of an equilateral triangle extends beyond theoretical exercises. It finds practical applications in various fields:
- Engineering: Designing structures with equilateral triangular supports, calculating the amount of material needed.
- Architecture: Designing aesthetically pleasing and structurally sound buildings using equilateral triangle patterns.
- Cartography: Determining distances on maps where triangular features are equilateral.
- Construction: Estimating the amount of fencing required for a triangular area.
- Art and Design: Creating symmetrical and balanced patterns.
By understanding the different methods outlined above, engineers, architects, and designers can accurately and efficiently solve real-world problems involving equilateral triangles.
Conclusion: Mastering Equilateral Triangle Perimeter Calculations
Calculating the perimeter of an equilateral triangle, while seemingly simple, offers a window into various geometric concepts and problem-solving techniques. From the straightforward formula of 3 * side to the more advanced applications of area, height, trigonometry, and radii, this article has explored a spectrum of approaches. Mastering these methods empowers you to tackle a wider array of geometric challenges and confidently apply these principles in various real-world contexts. Remember, understanding the underlying properties of the equilateral triangle—its equal sides and angles—is the key to unlocking efficient and accurate perimeter calculations. The choice of method will depend on the information provided in the specific problem. Practice is crucial to developing proficiency and gaining a deep understanding of this fundamental concept.
Latest Posts
Related Post
Thank you for visiting our website which covers about How Do You Find The Perimeter Of A Equilateral Triangle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.