How Many Degrees Is A Rectangle
News Co
Apr 05, 2025 · 5 min read
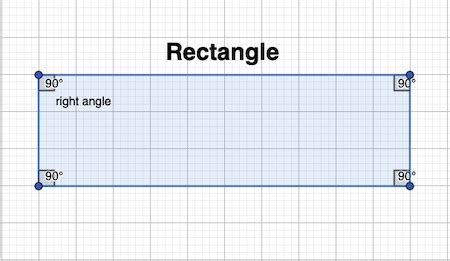
Table of Contents
How Many Degrees is a Rectangle? Understanding Angles and Properties
The question, "How many degrees is a rectangle?" might seem deceptively simple. While a quick answer might be "360 degrees," a deeper understanding requires exploring the fundamental properties of rectangles, their angles, and their relationship to other geometric shapes. This comprehensive guide will delve into the intricacies of rectangular angles, exploring the mathematical concepts and practical applications that underpin this seemingly basic geometric concept.
Defining a Rectangle: More Than Just Four Sides
A rectangle, in its simplest definition, is a quadrilateral—a four-sided polygon—with four right angles. This seemingly straightforward definition lays the groundwork for understanding its angular properties. However, it's crucial to appreciate that this simple definition encapsulates several critical geometric characteristics.
Key Properties of Rectangles:
- Four Sides: A rectangle possesses four straight sides.
- Four Right Angles: Each interior angle measures exactly 90 degrees. This is the defining characteristic of a rectangle, differentiating it from other quadrilaterals like parallelograms or trapezoids.
- Opposite Sides are Equal and Parallel: The lengths of opposite sides are identical, and these sides are parallel to each other.
- Diagonals Bisect Each Other: The diagonals of a rectangle—lines connecting opposite vertices—intersect at their midpoints, dividing each other into equal segments.
The Sum of Interior Angles: 360 Degrees
The total sum of the interior angles of any quadrilateral, including a rectangle, is always 360 degrees. This is a fundamental theorem in geometry. In the case of a rectangle, this 360-degree sum is divided equally among its four right angles, each contributing 90 degrees.
Mathematical Proof:
We can prove this using the concept of triangles. Draw a diagonal line across the rectangle, dividing it into two congruent right-angled triangles. The sum of angles in any triangle is always 180 degrees. Since the rectangle is divided into two triangles, the total sum of angles is 180 degrees * 2 = 360 degrees. This demonstrates that the sum of interior angles in a rectangle is always 360 degrees, regardless of the rectangle's dimensions.
Understanding the 90-Degree Angle: The Cornerstone of Rectangles
The 90-degree angle, also known as a right angle, is the core defining feature of a rectangle. It's crucial to understand what constitutes a right angle and its significance in various applications.
Defining a Right Angle:
A right angle is formed when two lines or rays intersect at a perfectly perpendicular angle. This means that the angle between them is precisely 90 degrees. We often represent right angles using a small square symbol in the corner where the lines meet.
Applications of Right Angles:
Right angles are ubiquitous in our everyday lives and essential in various fields, including:
- Construction: Buildings, bridges, and other structures heavily rely on right angles for stability and structural integrity.
- Engineering: Mechanical designs, blueprints, and computer-aided design (CAD) software all utilize right angles extensively.
- Architecture: The design and construction of houses, offices, and other buildings depend on precise right angles.
- Art and Design: Right angles are frequently used in creating symmetrical and balanced designs.
Rectangles and Other Geometric Shapes: Exploring Relationships
Understanding rectangles also requires understanding their relationship with other geometric shapes. Rectangles belong to a broader family of quadrilaterals, sharing some properties with other shapes while exhibiting distinct characteristics.
Rectangles vs. Squares:
A square is a special type of rectangle. All squares are rectangles, but not all rectangles are squares. The key difference lies in the side lengths: a square has all four sides of equal length, whereas a rectangle only requires opposite sides to be equal. However, both shapes maintain the defining characteristic of four 90-degree angles.
Rectangles vs. Parallelograms:
A parallelogram is a quadrilateral with opposite sides parallel. Rectangles are a subset of parallelograms; all rectangles are parallelograms, but not all parallelograms are rectangles. The crucial distinction is the presence of right angles—rectangles possess them, while parallelograms don't necessarily have them.
Rectangles vs. Trapezoids:
A trapezoid is a quadrilateral with at least one pair of parallel sides. Rectangles are distinct from trapezoids because rectangles have two pairs of parallel sides, while trapezoids only require one. Additionally, rectangles always possess right angles, whereas trapezoids do not.
Real-World Applications of Rectangular Geometry: Beyond the Textbook
The understanding of rectangular angles and properties extends far beyond theoretical geometry; it holds immense practical value in numerous real-world applications.
Architecture and Construction:
The construction of buildings, bridges, and other structures hinges on precise measurements and the use of right angles. Deviation from these angles can lead to structural instability and compromise safety. Architects and engineers rely heavily on accurate calculations and the understanding of rectangular geometry to ensure the structural integrity of their projects.
Computer Graphics and Design:
Computer graphics software and CAD programs extensively utilize rectangular coordinates and the properties of rectangles to create and manipulate images and designs. Understanding rectangular geometry is fundamental to creating accurate and efficient 2D and 3D models.
Cartography and Mapping:
Maps and geographical representations often utilize rectangular grids to organize and display spatial information. Understanding rectangular geometry allows cartographers to accurately represent geographical features and distances.
Manufacturing and Industry:
Many industrial processes and manufacturing techniques rely on the precise creation and manipulation of rectangular shapes. For instance, cutting materials, assembling components, and packaging products often involve rectangular dimensions and the application of right angles.
Conclusion: The Enduring Significance of Rectangular Angles
The seemingly simple question, "How many degrees is a rectangle?" opens a door to a world of geometric principles, mathematical concepts, and real-world applications. While the sum of a rectangle's interior angles is 360 degrees, the significance lies in the four crucial 90-degree angles that define its shape and properties. Understanding these properties is not just a matter of academic interest; it's essential for numerous professions and crucial to our everyday lives, from the buildings we inhabit to the technology we use. The enduring relevance of rectangular geometry highlights the power and practicality of basic geometric concepts. The 90-degree angle, therefore, is much more than just a number; it's a fundamental building block of our physical world and our understanding of shapes and space.
Latest Posts
Related Post
Thank you for visiting our website which covers about How Many Degrees Is A Rectangle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.