How Many Equivalent Fractions For 4 5
News Co
Apr 03, 2025 · 6 min read
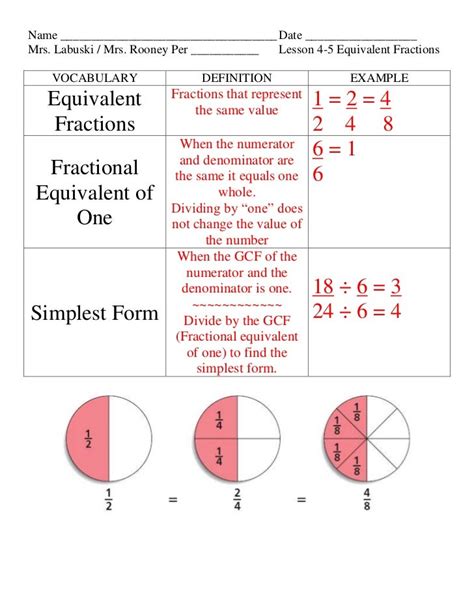
Table of Contents
How Many Equivalent Fractions Are There for 4/5? Exploring the Infinite Nature of Equivalent Fractions
The seemingly simple fraction 4/5 opens a door to a fascinating exploration of mathematical concepts. While the fraction itself represents a single value, the number of equivalent fractions representing that same value is, in fact, infinite. This article delves into the understanding of equivalent fractions, demonstrating why there are infinitely many ways to represent 4/5, and exploring the implications of this concept within mathematics.
Understanding Equivalent Fractions
Equivalent fractions represent the same proportion or value, even though they look different. They are created by multiplying or dividing both the numerator (top number) and the denominator (bottom number) of a fraction by the same non-zero number. This process essentially scales the fraction up or down, maintaining the same ratio.
For example, if we take the fraction 1/2 and multiply both the numerator and the denominator by 2, we get 2/4. Similarly, multiplying by 3 gives 3/6, by 4 gives 4/8, and so on. All of these fractions – 1/2, 2/4, 3/6, 4/8, etc. – are equivalent fractions, each representing one-half.
The Key Principle: The crucial element here is that we must multiply or divide both the numerator and the denominator by the same number. This preserves the fundamental ratio between the two parts of the fraction. If you only multiply or divide one part, you change the value of the fraction entirely.
Demonstrating the Infinity of Equivalent Fractions for 4/5
Let's apply this principle to the fraction 4/5. We can generate equivalent fractions by multiplying both the numerator and denominator by any non-zero integer:
- Multiplying by 2: (4 x 2) / (5 x 2) = 8/10
- Multiplying by 3: (4 x 3) / (5 x 3) = 12/15
- Multiplying by 4: (4 x 4) / (5 x 4) = 16/20
- Multiplying by 5: (4 x 5) / (5 x 5) = 20/25
- Multiplying by 10: (4 x 10) / (5 x 10) = 40/50
- Multiplying by 100: (4 x 100) / (5 x 100) = 400/500
- Multiplying by 'x': (4x) / (5x), where 'x' can be any non-zero integer.
As you can see, we can continue this process indefinitely. There's no limit to the integers we can multiply by. Therefore, the number of equivalent fractions for 4/5 is infinite.
This concept extends beyond just integers. We could also multiply by fractions (e.g., 1/2, 3/4) or even irrational numbers (e.g., π, √2), although the resulting equivalent fractions might be less straightforward to work with. The fundamental principle remains the same: multiplying both the numerator and the denominator by the same value maintains the equivalent value.
Visualizing Equivalent Fractions
Imagine a pizza cut into 5 slices. If you take 4 of those slices, you have 4/5 of the pizza. Now imagine cutting each of those 5 slices into two equal parts. You now have 10 slices, and taking 8 of them (double the amount of slices you originally had) still represents the same amount of pizza: 8/10. This visually demonstrates the concept of equivalent fractions. You can repeat this process – cutting into smaller and smaller pieces – indefinitely, generating infinitely many equivalent fractions.
The Importance of Equivalent Fractions in Mathematics
The concept of equivalent fractions is fundamental to many areas of mathematics, including:
1. Simplifying Fractions
Equivalent fractions allow us to simplify fractions to their lowest terms. This is achieved by dividing both the numerator and the denominator by their greatest common divisor (GCD). For example, the fraction 8/10 can be simplified to 4/5 by dividing both by their GCD, which is 2. Simplifying fractions makes them easier to understand and work with.
2. Adding and Subtracting Fractions
When adding or subtracting fractions, we need to find a common denominator. This often involves finding equivalent fractions with the same denominator. For instance, to add 1/2 and 1/4, we can convert 1/2 to its equivalent fraction 2/4, making the addition straightforward (2/4 + 1/4 = 3/4).
3. Comparing Fractions
Determining which of two fractions is larger or smaller is often easier if we find equivalent fractions with a common denominator. For example, comparing 3/4 and 5/6 might be easier after converting them to equivalent fractions with a common denominator of 12: 9/12 and 10/12. This makes it clear that 5/6 is larger.
4. Ratio and Proportion
Equivalent fractions form the basis of understanding ratio and proportion. They are used extensively in solving problems involving scaling, mixtures, and similar figures in geometry.
5. Algebra and Calculus
The concept of equivalent fractions extends into more advanced mathematics like algebra and calculus. It plays a crucial role in simplifying algebraic expressions, solving equations, and performing calculations involving rational functions.
Beyond the Integers: Exploring Other Multipliers
As mentioned earlier, we are not limited to multiplying by integers. Let's consider multiplying by rational numbers (fractions):
-
Multiplying by 1/2: (4 x 1/2) / (5 x 1/2) = 2/2.5. While this looks different, it simplifies back to 4/5.
-
Multiplying by 3/4: (4 x 3/4) / (5 x 3/4) = 3/3.75. Again, this is equivalent to 4/5.
The key takeaway remains consistent: multiplying both the numerator and denominator by the same non-zero value maintains the equivalence. The resulting fractions may appear more complex due to decimal numbers, but their underlying value remains the same.
Practical Applications and Real-World Examples
The concept of equivalent fractions isn't merely a theoretical exercise; it has numerous real-world applications:
-
Cooking and Baking: Recipes often use fractional measurements. Understanding equivalent fractions allows for adjusting recipes to use different quantities of ingredients while maintaining the same ratios.
-
Construction and Engineering: Accurate measurements and proportions are vital in construction and engineering. Equivalent fractions help ensure that designs and structures are built to the correct specifications.
-
Finance and Economics: Fractions are frequently used in finance to represent portions of investments, debts, or interest rates. Understanding equivalent fractions is essential for accurate financial calculations.
-
Data Analysis: In data analysis, proportions and ratios are often represented as fractions. Working with equivalent fractions can simplify data interpretation and comparisons.
-
Mapping and Cartography: Maps use scales to represent real-world distances. Understanding equivalent fractions allows for accurate calculations of real distances from map measurements.
Conclusion: The Endlessness of Equivalence
The question "How many equivalent fractions are there for 4/5?" has a definitive answer: infinitely many. The ability to multiply both the numerator and denominator by any non-zero number allows for the creation of an endless stream of equivalent fractions, each representing the same proportion. Understanding this concept is not merely an exercise in theoretical mathematics; it is a fundamental building block for various mathematical concepts and real-world applications. The seemingly simple fraction 4/5 therefore provides a gateway to a much broader and infinitely rich mathematical landscape. Mastering this concept solidifies a strong foundation for further mathematical explorations.
Latest Posts
Related Post
Thank you for visiting our website which covers about How Many Equivalent Fractions For 4 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.