How Many Lines Of Symmetry Does Isosceles Triangle Have
News Co
May 09, 2025 · 4 min read
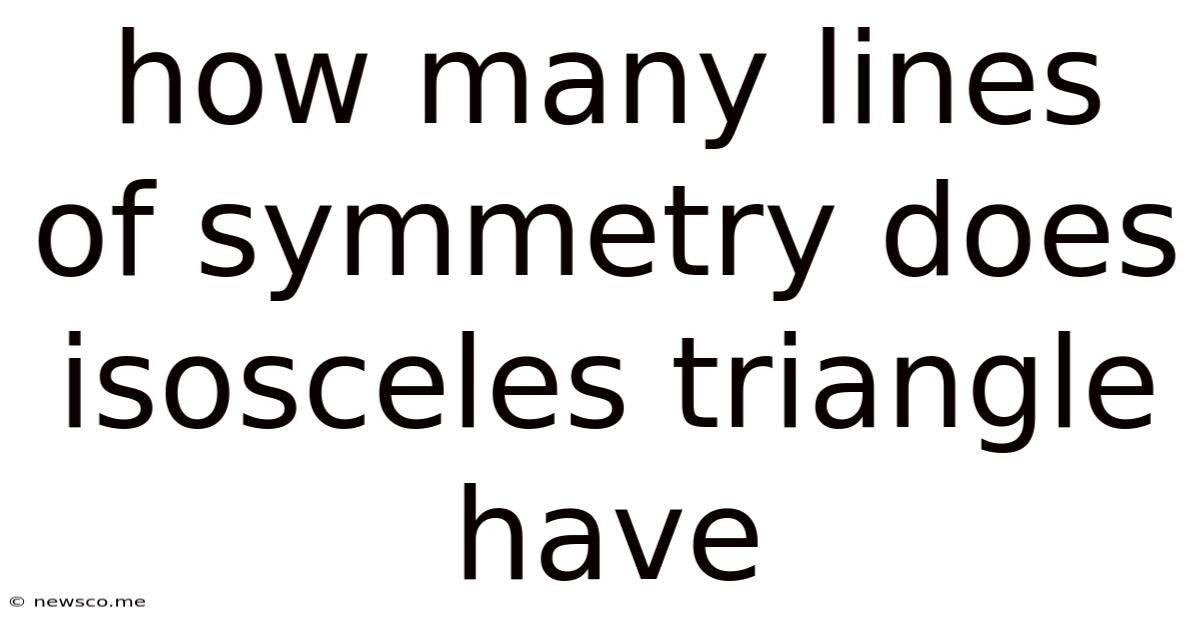
Table of Contents
How Many Lines of Symmetry Does an Isosceles Triangle Have?
Understanding lines of symmetry is fundamental in geometry, offering insights into the inherent properties of shapes. This article delves deep into the fascinating world of isosceles triangles and their lines of symmetry, exploring the concept thoroughly and addressing common misconceptions. We'll investigate the definition of symmetry, explore different types of triangles, and ultimately determine the number of lines of symmetry an isosceles triangle possesses.
Defining Symmetry and Lines of Symmetry
Before we tackle isosceles triangles, let's establish a clear understanding of symmetry and lines of symmetry. Symmetry refers to a balanced and proportionate arrangement of parts in an object. A shape is symmetrical if it can be folded along a line so that the two halves match exactly. This line of folding is known as a line of symmetry, also called an axis of symmetry. Think of it as a mirror – one half reflects perfectly onto the other.
Not all shapes possess lines of symmetry. Some might have several, while others might have none. The number of lines of symmetry depends entirely on the shape's geometry.
Exploring Different Types of Triangles
Triangles are classified based on their side lengths and angles. Understanding these classifications is crucial to determine their lines of symmetry:
1. Equilateral Triangles:
An equilateral triangle has three sides of equal length and three equal angles (each measuring 60 degrees). This perfect balance results in three lines of symmetry. Each line connects a vertex (corner) to the midpoint of the opposite side.
2. Isosceles Triangles:
An isosceles triangle has two sides of equal length, called legs. The third side, of different length, is called the base. The angles opposite the equal sides are also equal. This property leads to a unique characteristic regarding its symmetry.
3. Scalene Triangles:
A scalene triangle has all three sides of different lengths, and consequently, all three angles are also different. Due to this lack of equal sides or angles, a scalene triangle has no lines of symmetry.
Determining the Lines of Symmetry in an Isosceles Triangle
Now, let's focus on the core question: how many lines of symmetry does an isosceles triangle have?
The answer is: one.
An isosceles triangle has only one line of symmetry, which is the perpendicular bisector of the base. This line passes through the vertex opposite the base and bisects the base at a 90-degree angle. When folded along this line, the two halves of the isosceles triangle perfectly overlap.
Why only one?
Unlike an equilateral triangle, the two sides of an isosceles triangle are equal in length, and the angles opposite those sides are equal. However, the third side is unequal. Therefore, there's only one line that can be drawn to divide the triangle into two mirror images. Any other line drawn through the triangle will not result in perfectly symmetrical halves.
Let's visualize this:
Imagine an isosceles triangle with a base of length 'b' and two equal sides of length 'a'. The line of symmetry bisects the base 'b', dividing it into two equal segments of length 'b/2'. This line also passes through the vertex opposite the base, forming a right angle with the base.
Common Misconceptions about Isosceles Triangle Symmetry
A common misconception is that an isosceles triangle has multiple lines of symmetry. This stems from confusing isosceles triangles with equilateral triangles. It's crucial to remember that only equilateral triangles, with their three equal sides and angles, possess three lines of symmetry.
Another misconception is that the altitude from any vertex could be a line of symmetry. While the altitude from the vertex opposite the base is the line of symmetry, the altitudes drawn from the other two vertices do not bisect the triangle into symmetrical halves.
Applications of Symmetry in Isosceles Triangles
The concept of symmetry in isosceles triangles has practical applications in various fields:
- Architecture and Design: Symmetrical designs, including those based on isosceles triangles, create visually appealing and balanced structures. Many architectural marvels incorporate symmetrical elements for aesthetic and structural reasons.
- Engineering: Understanding lines of symmetry is essential in structural engineering to ensure stability and balance in designs.
- Art and Craft: Isosceles triangles frequently appear in art, crafting, and design projects, their symmetry providing a sense of harmony and balance.
Advanced Considerations: Degenerate Cases
While the typical isosceles triangle has one line of symmetry, it's important to consider degenerate cases. A degenerate isosceles triangle, where two sides become collinear, would have infinite lines of symmetry along that line. However, this is a specific and less common scenario.
Conclusion: The Single Line of Symmetry in Isosceles Triangles
In summary, an isosceles triangle possesses only one line of symmetry. This line is the perpendicular bisector of its base. Understanding this fundamental property is essential for various applications in geometry, mathematics, and related fields. By clarifying the differences between isosceles and equilateral triangles, we can appreciate the unique characteristics and the single line of symmetry that defines the beautiful geometry of isosceles triangles. Remember, the key is to visualize the fold – only one line allows for a perfect reflection.
Latest Posts
Latest Posts
-
Find The Point On The Y Axis Which Is Equidistant From
May 09, 2025
-
Is 3 4 Bigger Than 7 8
May 09, 2025
-
Which Of These Is Not A Prime Number
May 09, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 09, 2025
-
Are Alternate Exterior Angles Always Congruent
May 09, 2025
Related Post
Thank you for visiting our website which covers about How Many Lines Of Symmetry Does Isosceles Triangle Have . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.