How Many Symmetrical Lines Does A Rectangle Have
News Co
Apr 06, 2025 · 6 min read
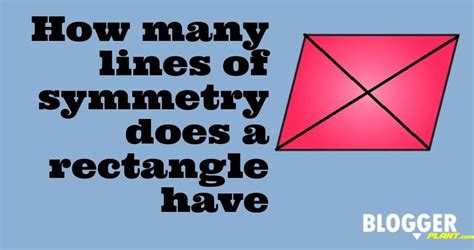
Table of Contents
How Many Lines of Symmetry Does a Rectangle Have? A Comprehensive Exploration
Symmetry, a fundamental concept in geometry and art, refers to a balanced and proportionate arrangement of parts. Understanding lines of symmetry, also known as axes of symmetry, is crucial for grasping the geometrical properties of shapes. This article delves into the fascinating world of symmetry, focusing specifically on the lines of symmetry possessed by a rectangle. We'll explore the definition of symmetry, different types of rectangles, and the implications of symmetry in various fields.
Defining Symmetry and Lines of Symmetry
Before we dive into the specifics of rectangles, let's establish a clear understanding of symmetry. Symmetry implies that a shape can be divided into two or more identical parts that are mirror images of each other. This division is achieved through a line of symmetry, also known as an axis of symmetry. A line of symmetry is a line that divides a shape into two congruent halves, such that if you fold the shape along this line, both halves perfectly overlap.
Imagine folding a piece of paper in half. If the resulting halves match exactly, the fold line represents a line of symmetry. This principle applies to various two-dimensional shapes, including rectangles, squares, circles, and triangles. The number of lines of symmetry a shape possesses depends on its geometrical properties.
Exploring the Rectangle: A Definition
A rectangle is a quadrilateral, meaning a four-sided polygon, with specific properties. These properties define a rectangle and differentiate it from other quadrilaterals such as squares, parallelograms, and rhombuses. The defining characteristics of a rectangle include:
- Four right angles: Each of the four interior angles measures exactly 90 degrees.
- Opposite sides are equal and parallel: The opposite sides of a rectangle have the same length and are parallel to each other.
How Many Lines of Symmetry Does a Rectangle Possess?
Now, let's address the central question: how many lines of symmetry does a rectangle have? The answer is two. A rectangle possesses two lines of symmetry:
-
A vertical line of symmetry: This line runs vertically through the center of the rectangle, dividing it into two identical halves. If you fold the rectangle along this line, the left and right halves will perfectly overlap.
-
A horizontal line of symmetry: This line runs horizontally through the center of the rectangle, dividing it into two identical halves. Folding the rectangle along this line will result in the top and bottom halves overlapping perfectly.
These two lines of symmetry are essential characteristics that distinguish a rectangle from other quadrilaterals. A square, for instance, has four lines of symmetry – two vertical and two horizontal – because of its added property of having all sides equal in length. Other quadrilaterals may have fewer or no lines of symmetry at all.
Understanding the Different Types of Rectangles
It's important to note that while all rectangles share the same fundamental properties of having four right angles and opposite sides that are equal and parallel, there are different types of rectangles based on their side lengths:
-
Square: A special case of a rectangle where all four sides are of equal length. As mentioned earlier, a square possesses four lines of symmetry.
-
Oblong: A rectangle where the length and width are unequal. These maintain the two lines of symmetry found in all rectangles.
Regardless of whether the rectangle is a square or an oblong, the number of lines of symmetry remains consistent: two. The additional symmetry in a square stems from its equal side lengths.
The Significance of Symmetry in Rectangles
The presence of lines of symmetry in rectangles has significant implications in various fields:
-
Architecture and Design: Rectangles, with their inherent symmetry, are frequently used in architecture and design for creating aesthetically pleasing and balanced structures. Buildings, rooms, and furniture often incorporate rectangular shapes because of their visual appeal and structural stability.
-
Art and Graphics: Artists and graphic designers utilize rectangles and their symmetrical properties to create balanced and harmonious compositions. The symmetry contributes to a sense of order and visual stability in artwork.
-
Engineering and Manufacturing: In engineering and manufacturing, symmetrical designs are often preferred for their stability and ease of production. The symmetrical nature of rectangles simplifies manufacturing processes and ensures consistent product quality.
-
Mathematics and Geometry: Rectangles and their lines of symmetry play a crucial role in the study of geometry and mathematics. They serve as a fundamental building block for understanding more complex geometrical concepts and theorems.
Beyond the Basics: Exploring Rotational Symmetry
While we've focused on lines of symmetry, it's also worthwhile to briefly discuss rotational symmetry. Rotational symmetry refers to a shape's ability to be rotated around a central point and still appear unchanged. A rectangle has rotational symmetry of order 2, meaning it looks the same after a 180-degree rotation around its center. This is distinct from its two lines of symmetry.
Practical Applications and Real-World Examples
The concept of lines of symmetry in rectangles is not just a theoretical exercise; it has tangible applications in our everyday lives. Consider these examples:
-
Building a House: The rectangular shape of rooms and the symmetrical placement of windows and doors contribute to a balanced and aesthetically pleasing house design. The symmetry in the design ensures efficient space utilization and visual harmony.
-
Designing a Website: Web designers frequently utilize rectangular elements and their inherent symmetry to create visually appealing and user-friendly websites. The symmetrical arrangement of content enhances the overall user experience.
-
Creating a Logo: Many logos incorporate rectangular shapes and their symmetrical properties to create memorable and impactful designs. The symmetry in the logo contributes to its visual appeal and brand recognition.
-
Playing Games: Many board games and puzzles utilize rectangular grids, leveraging the inherent symmetry of the rectangle for game mechanics and visual clarity. This symmetry simplifies gameplay and improves the overall game design.
Conclusion: Symmetry in Rectangles and Beyond
Understanding the number of lines of symmetry in a rectangle – two – is fundamental to grasping its geometrical properties and its applications across various disciplines. From architecture and design to mathematics and everyday life, the symmetrical nature of rectangles plays a significant role in creating aesthetically pleasing, functional, and efficient designs. While this article primarily focused on rectangles, exploring the lines of symmetry in other shapes further enriches our understanding of geometric concepts and their practical applications in our world. The principles of symmetry extend far beyond simple shapes, influencing art, science, and engineering in profound ways. Appreciating these underlying principles allows us to better understand the world around us and to create more beautiful, functional, and balanced designs.
Latest Posts
Related Post
Thank you for visiting our website which covers about How Many Symmetrical Lines Does A Rectangle Have . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.