How To Change A Ratio To A Percent
News Co
Apr 03, 2025 · 5 min read
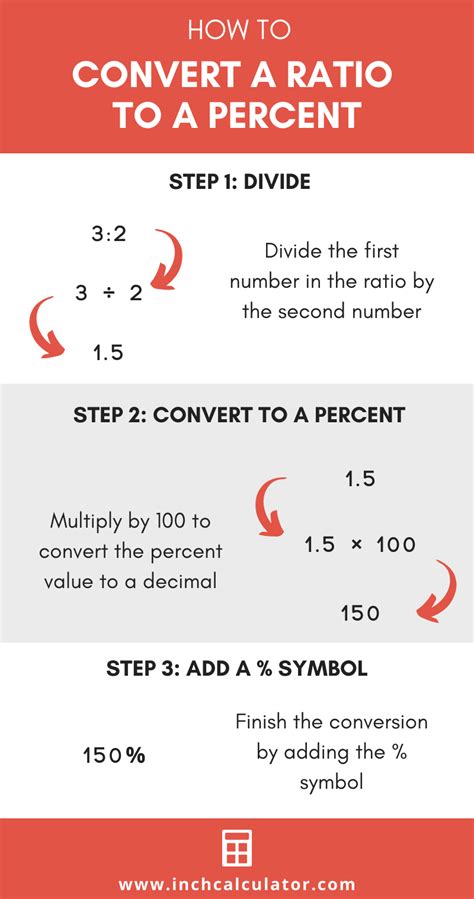
Table of Contents
How to Change a Ratio to a Percent: A Comprehensive Guide
Converting ratios to percentages is a fundamental skill with widespread applications across various fields, from finance and statistics to everyday life. Understanding this conversion is crucial for interpreting data, making comparisons, and solving problems effectively. This comprehensive guide will walk you through the process step-by-step, covering various scenarios and providing practical examples to solidify your understanding.
Understanding Ratios and Percentages
Before diving into the conversion process, let's briefly revisit the concepts of ratios and percentages.
What is a Ratio?
A ratio is a comparison of two or more quantities. It shows the relative sizes of the quantities. Ratios can be expressed in several ways:
- Using the colon symbol (:): For example, the ratio of boys to girls in a class might be 3:2.
- Using the word "to": The same ratio can be written as "3 to 2".
- As a fraction: The ratio 3:2 can also be expressed as 3/2.
What is a Percentage?
A percentage is a way of expressing a number as a fraction of 100. It represents a part of a whole, expressed as a portion of 100. The symbol "%" is used to denote percentages. For example, 25% means 25 out of 100, or 25/100.
Converting a Ratio to a Percentage: The Step-by-Step Process
The process of converting a ratio to a percentage involves these key steps:
-
Express the ratio as a fraction: If the ratio isn't already in fraction form (e.g., 3:2), convert it into a fraction (3/2).
-
Divide the numerator by the denominator: Perform the division to obtain a decimal. In our example, 3/2 = 1.5.
-
Multiply the decimal by 100: This step converts the decimal into a percentage. Multiplying 1.5 by 100 gives us 150.
-
Add the percentage symbol (%): Finally, add the "%" symbol to indicate that the result is a percentage. Therefore, the ratio 3:2 is equivalent to 150%.
Examples: Converting Different Types of Ratios
Let's illustrate the conversion process with various examples, demonstrating different scenarios you might encounter.
Example 1: Simple Ratio
Ratio: 1:4 (representing, for instance, the ratio of red balls to blue balls in a bag).
- Fraction: 1/4
- Decimal: 1 ÷ 4 = 0.25
- Percentage: 0.25 × 100 = 25%
Therefore, the ratio 1:4 is equivalent to 25%.
Example 2: Ratio Greater Than 1
Ratio: 5:2 (representing, for example, the ratio of wins to losses for a sports team).
- Fraction: 5/2
- Decimal: 5 ÷ 2 = 2.5
- Percentage: 2.5 × 100 = 250%
In this case, the ratio 5:2 represents 250%. This indicates that the number of wins is 250% of the number of losses.
Example 3: Ratio with Decimals
Ratio: 2.5:5 (representing, perhaps, the ratio of ingredient A to ingredient B in a recipe).
- Fraction: 2.5/5
- Decimal: 2.5 ÷ 5 = 0.5
- Percentage: 0.5 × 100 = 50%
The ratio 2.5:5 is equivalent to 50%.
Example 4: Ratio involving larger numbers
Ratio: 350:700
- Fraction: 350/700
- Decimal: 350 ÷ 700 = 0.5
- Percentage: 0.5 × 100 = 50%
Example 5: Converting a ratio with three parts to percentages
Sometimes, you might encounter ratios with more than two parts. Let’s consider a ratio of 2:3:5. To convert this to percentages, we first need to find the total parts.
- Total Parts: 2 + 3 + 5 = 10
- Individual Fractions: 2/10, 3/10, 5/10
- Decimals: 0.2, 0.3, 0.5
- Percentages: 20%, 30%, 50%
Practical Applications: Where Ratio to Percentage Conversion is Useful
The conversion of ratios to percentages finds numerous practical applications in diverse fields:
-
Finance: Calculating interest rates, profit margins, and return on investments often involve converting ratios to percentages. For example, understanding the ratio of debt to equity of a company as a percentage provides crucial financial insights.
-
Statistics: Expressing proportions and probabilities as percentages enhances data comprehension and facilitates meaningful comparisons.
-
Science: Many scientific experiments and analyses involve ratios that are ultimately converted to percentages for easy interpretation and presentation.
-
Everyday Life: Calculating discounts, expressing survey results, and interpreting sports statistics frequently rely on converting ratios to percentages. For instance, understanding a 20% discount on a product requires converting the ratio of the discount to the original price into a percentage.
Common Mistakes to Avoid
While the conversion process is straightforward, some common mistakes can lead to inaccuracies:
-
Incorrect order of division: Always divide the numerator by the denominator, not the other way around.
-
Forgetting to multiply by 100: This crucial step is necessary to convert the decimal to a percentage.
-
Misinterpreting percentages greater than 100%: Percentages exceeding 100% simply indicate that the numerator is larger than the denominator. This isn’t inherently incorrect and has valid interpretations within specific contexts.
-
Rounding errors: While rounding might be necessary for simplification, try to minimize rounding errors, especially in critical calculations where precision is vital.
Advanced Techniques: Handling Complex Ratios
While the basic method covers most scenarios, more complex ratios might necessitate additional steps:
-
Ratios with more than two parts: As demonstrated earlier, handle these by calculating the total parts and then finding the individual percentages.
-
Ratios with variables: If the ratio involves variables (algebraic expressions), follow the same steps, treating the variables as numbers. The final percentage might also be an algebraic expression.
-
Ratios with units: If the ratio involves units (e.g., meters, kilograms), ensure that the units are consistent before converting to a percentage. The resulting percentage would be unitless.
Conclusion: Mastering the Conversion
Converting ratios to percentages is a crucial skill applicable across numerous contexts. By understanding the step-by-step process, practicing with varied examples, and avoiding common pitfalls, you can confidently convert ratios to percentages and effectively utilize this knowledge in various fields. This comprehensive guide has provided the foundational knowledge and practical techniques needed to master this essential mathematical skill. Consistent practice will further enhance your understanding and efficiency in performing these conversions. Remember, understanding the underlying principles ensures accurate and insightful results.
Latest Posts
Related Post
Thank you for visiting our website which covers about How To Change A Ratio To A Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.