How To Convert A Ratio To A Percentage
News Co
Apr 02, 2025 · 5 min read
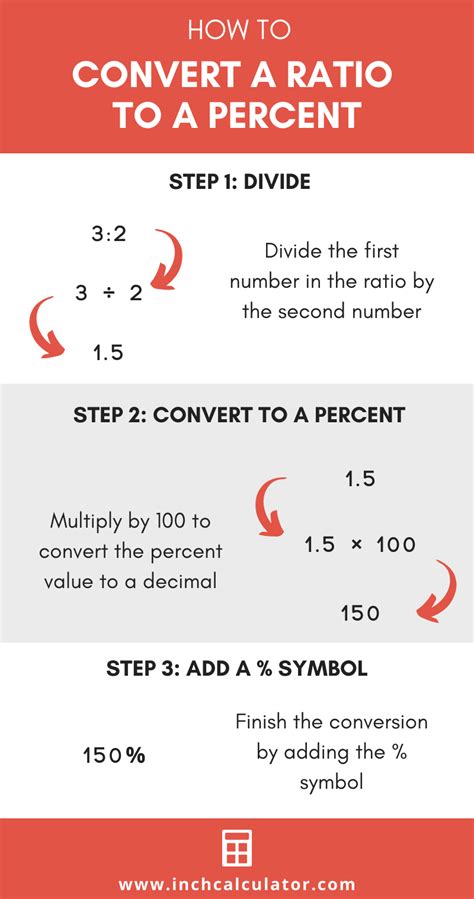
Table of Contents
How to Convert a Ratio to a Percentage: A Comprehensive Guide
Converting ratios to percentages is a fundamental skill with wide-ranging applications, from understanding financial reports to analyzing survey data. While seemingly simple, mastering this conversion ensures accuracy and clarity in various contexts. This comprehensive guide will walk you through the process, offering different approaches and addressing common pitfalls. We'll explore the underlying concepts, provide step-by-step instructions with examples, and delve into scenarios where this conversion is particularly useful.
Understanding Ratios and Percentages
Before diving into the conversion process, it's essential to grasp the core concepts of ratios and percentages.
What is a Ratio?
A ratio is a mathematical comparison of two or more numbers. It shows the relative size of one number to another. Ratios can be expressed in several ways:
- Using the colon (:): For example, 3:5 represents a ratio of 3 to 5.
- Using the word "to": 3 to 5.
- As a fraction: 3/5.
What is a Percentage?
A percentage is a way of expressing a number as a fraction of 100. It represents a proportion out of a whole. The symbol "%" signifies "per hundred". For instance, 75% means 75 out of 100.
Converting Ratios to Percentages: A Step-by-Step Guide
The conversion process involves transforming a ratio (expressed as a fraction) into a decimal, and then multiplying by 100 to obtain the percentage. Let's break it down step-by-step:
Step 1: Express the Ratio as a Fraction
If your ratio isn't already in fractional form, convert it. For example, the ratio 3:5 becomes the fraction 3/5.
Step 2: Divide the Numerator by the Denominator
Divide the top number (numerator) of the fraction by the bottom number (denominator). Using the example above:
3 ÷ 5 = 0.6
Step 3: Multiply the Result by 100
Take the decimal result from Step 2 and multiply it by 100. This converts the decimal to a percentage.
0.6 × 100 = 60
Step 4: Add the Percentage Symbol (%)
Finally, add the percentage symbol (%) to your answer.
Therefore, the ratio 3:5 is equivalent to 60%.
Worked Examples: Different Ratio Types
Let's tackle a variety of ratio types to solidify your understanding:
Example 1: Simple Ratio
Ratio: 2:8
Steps:
- Fraction: 2/8
- Division: 2 ÷ 8 = 0.25
- Multiplication: 0.25 × 100 = 25
- Percentage: 25%
Therefore, the ratio 2:8 is equal to 25%.
Example 2: Ratio with Larger Numbers
Ratio: 150:300
Steps:
- Fraction: 150/300
- Division: 150 ÷ 300 = 0.5
- Multiplication: 0.5 × 100 = 50
- Percentage: 50%
The ratio 150:300 simplifies to 50%.
Example 3: Ratio Greater Than 1
Ratio: 5:2
Steps:
- Fraction: 5/2
- Division: 5 ÷ 2 = 2.5
- Multiplication: 2.5 × 100 = 250
- Percentage: 250%
Note that percentages can be greater than 100%, indicating a proportion larger than the whole.
Example 4: Ratio Involving Decimals
Ratio: 0.75 : 1.5
Steps:
- Fraction: 0.75/1.5
- Division: 0.75 ÷ 1.5 = 0.5
- Multiplication: 0.5 × 100 = 50
- Percentage: 50%
This example demonstrates that the process remains the same even with decimal numbers in the ratio.
Applications of Ratio to Percentage Conversion
The ability to convert ratios to percentages is crucial across diverse fields:
Finance and Investment
Understanding financial statements often involves analyzing ratios like debt-to-equity ratios or profit margins. Converting these ratios to percentages provides a clear and easily interpretable picture of financial health.
Data Analysis and Statistics
In statistical analysis, ratios are frequently encountered in survey results, experimental data, or demographic breakdowns. Converting these ratios into percentages simplifies data interpretation and presentation.
Science and Engineering
Many scientific and engineering calculations involve ratios, such as concentrations in chemistry or efficiency in engineering. Percentage conversion aids in comparing and understanding these ratios effectively.
Everyday Life
From calculating discounts in stores to understanding election results, the ability to convert ratios to percentages is a valuable everyday life skill.
Common Mistakes and How to Avoid Them
While the conversion process is straightforward, several common mistakes can lead to inaccuracies:
- Incorrect Order of Division: Always divide the numerator by the denominator. Reversing the order will yield an incorrect result.
- Forgetting the Multiplication by 100: This crucial step transforms the decimal into a percentage. Omitting it results in a decimal value, not a percentage.
- Misinterpreting Ratios: Ensure you correctly identify the numerator and denominator within the ratio before beginning the calculation.
- Rounding Errors: When working with decimals, be mindful of rounding errors. Rounding too early in the calculation can affect the final percentage significantly. Try to use the full decimal value in your calculations before rounding the final result.
Advanced Techniques and Considerations
Dealing with Complex Ratios
For more complex ratios involving multiple numbers (e.g., 2:3:5), you can handle them individually by comparing each part to the total. For the ratio 2:3:5, the total is 10. Then, each part can be converted to a percentage:
- 2/10 = 0.2 = 20%
- 3/10 = 0.3 = 30%
- 5/10 = 0.5 = 50%
Using a Calculator
Most calculators can easily handle this conversion. Simply input the fraction and use the percentage function to convert it directly.
Conclusion
Converting ratios to percentages is a fundamental mathematical skill applicable across a vast range of disciplines. By understanding the steps, practicing with different examples, and avoiding common pitfalls, you can confidently and accurately convert any ratio to its percentage equivalent. This skill enhances your ability to interpret data, analyze information, and communicate findings effectively across various contexts. Remember the key steps: express as a fraction, divide the numerator by the denominator, multiply by 100, and add the percentage symbol. Mastering this skill empowers you to make better sense of the numerical world around you.
Latest Posts
Related Post
Thank you for visiting our website which covers about How To Convert A Ratio To A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.