How To Divide Mixed Fractions And Whole Numbers
News Co
Apr 02, 2025 · 5 min read
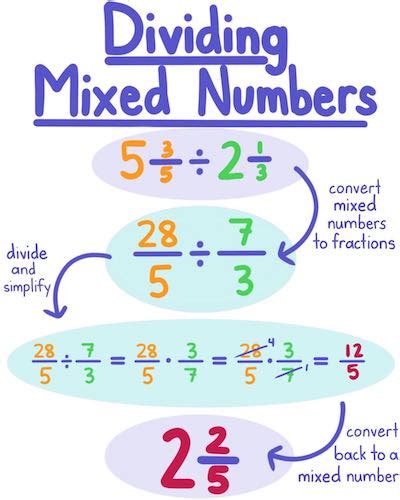
Table of Contents
How to Divide Mixed Fractions and Whole Numbers: A Comprehensive Guide
Dividing mixed fractions and whole numbers can seem daunting, but with a structured approach and a solid understanding of the underlying principles, it becomes a manageable and even enjoyable mathematical task. This comprehensive guide will walk you through the process step-by-step, offering numerous examples and tips to solidify your understanding. We'll explore various methods, catering to different learning styles and preferences. By the end, you'll be confident in tackling any mixed fraction and whole number division problem.
Understanding the Fundamentals
Before diving into the division process, let's refresh our understanding of key concepts:
1. Mixed Fractions: A Recap
A mixed fraction combines a whole number and a proper fraction. For example, 2 ¾ represents two whole units and three-quarters of another unit. Understanding this composition is crucial for division.
2. Converting Mixed Fractions to Improper Fractions
The most efficient way to divide mixed fractions is by converting them into improper fractions. An improper fraction has a numerator (top number) larger than or equal to its denominator (bottom number). To convert:
- Multiply the whole number by the denominator.
- Add the result to the numerator.
- Keep the same denominator.
Example: Convert 2 ¾ to an improper fraction.
- 2 (whole number) * 4 (denominator) = 8
- 8 + 3 (numerator) = 11
- The improper fraction is 11/4.
3. Reciprocals: The Key to Division
Division of fractions involves using the reciprocal of the divisor (the number you're dividing by). The reciprocal is simply the fraction flipped upside down. For example, the reciprocal of 2/3 is 3/2. The reciprocal of a whole number is that number written as a fraction with a denominator of 1 (e.g., the reciprocal of 5 is 1/5).
Method 1: Converting to Improper Fractions and Multiplying
This is generally the preferred method for its efficiency and clarity.
Steps:
- Convert the mixed fraction to an improper fraction: Follow the steps outlined above.
- Convert the whole number to a fraction: Write the whole number as a fraction with a denominator of 1.
- Invert the divisor (whole number fraction) to find its reciprocal.
- Multiply the fractions: Multiply the numerators together and the denominators together.
- Simplify the resulting fraction: Reduce the fraction to its lowest terms if possible, and convert back to a mixed number if needed.
Example: Divide 2 ¾ by 5.
- Convert 2 ¾ to an improper fraction: 11/4
- Convert 5 to a fraction: 5/1
- Find the reciprocal of 5/1: 1/5
- Multiply: (11/4) * (1/5) = 11/20
- The result is 11/20 (this is already in its simplest form).
Example 2 (requiring simplification): Divide 3 1/2 by 2.
- Convert 3 1/2 to an improper fraction: 7/2
- Convert 2 to a fraction: 2/1
- Find the reciprocal of 2/1: 1/2
- Multiply: (7/2) * (1/2) = 7/4
- Simplify 7/4 to a mixed number: 1 ¾
Method 2: Dividing Directly (Less Efficient)
While less efficient, this method provides a deeper understanding of the underlying process. It's suitable for simpler problems.
Steps:
- Divide the whole number part of the mixed fraction by the whole number divisor.
- Divide the fractional part of the mixed fraction by the whole number divisor.
- Combine the results.
Example: Divide 2 ¾ by 5.
- Divide the whole number part: 2 ÷ 5 = 0 with a remainder of 2.
- Convert the remainder to an improper fraction: 2 3/4. The "2" is added to the fractional part, turning 2 3/4 into 11/4.
- Divide the fractional part: 11/4 ÷ 5 = (11/4) * (1/5) = 11/20
This method is more complex and prone to errors, especially with larger numbers or more complex fractions. Therefore, Method 1 (converting to improper fractions) is strongly recommended for most situations.
Handling Complex Scenarios
Let's explore scenarios that introduce additional layers of complexity:
Dividing Mixed Fractions by Mixed Fractions
The same principles apply. Convert both mixed fractions to improper fractions, find the reciprocal of the divisor, and multiply.
Example: Divide 1 2/3 by 2 1/2.
- Convert 1 2/3 to an improper fraction: 5/3
- Convert 2 1/2 to an improper fraction: 5/2
- Find the reciprocal of 5/2: 2/5
- Multiply: (5/3) * (2/5) = 10/15
- Simplify: 10/15 = 2/3
Dealing with Zero
Remember that division by zero is undefined. A calculation involving division by zero will not yield a meaningful result.
Practical Applications and Real-World Examples
Understanding mixed fraction division is essential in various real-world applications:
- Cooking and Baking: Scaling recipes up or down often requires dividing mixed fraction quantities (e.g., dividing a recipe that calls for 2 ½ cups of flour by 2 to halve the recipe).
- Construction and Engineering: Calculating material requirements and dimensions frequently involves mixed fractions.
- Sewing and Quilting: Pattern adjustments and fabric calculations often necessitate dividing mixed fractions.
- Data Analysis: Working with data sets might require dividing measurements or proportions represented as mixed fractions.
Troubleshooting Common Mistakes
- Forgetting to convert to improper fractions: This is the most common error. Always convert mixed fractions to improper fractions before multiplying.
- Incorrectly finding the reciprocal: Double-check that you're inverting the correct fraction (the divisor).
- Simplification errors: Ensure that you simplify the final fraction to its lowest terms.
Conclusion: Mastering Mixed Fraction Division
By consistently applying the steps outlined above and practicing regularly, you'll confidently navigate the world of mixed fraction and whole number division. Remember that converting to improper fractions is the key to efficiency and accuracy. With practice and patience, this seemingly complex task will become second nature. Don't hesitate to revisit the examples and work through additional problems to solidify your understanding. Mastering this skill will empower you to tackle more advanced mathematical concepts and real-world challenges with ease.
Latest Posts
Related Post
Thank you for visiting our website which covers about How To Divide Mixed Fractions And Whole Numbers . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.