How To Find Length And Width With Perimeter
News Co
Apr 06, 2025 · 5 min read
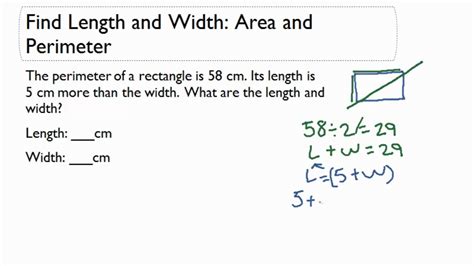
Table of Contents
How to Find Length and Width with Perimeter: A Comprehensive Guide
Determining the length and width of a rectangle when you only know its perimeter might seem like a tricky geometry problem, but with the right approach and understanding, it becomes straightforward. This comprehensive guide breaks down the process, exploring various scenarios and offering practical examples to help you master this essential skill. We'll delve into different methods, addressing potential challenges and providing tips for accurate calculations. Let's unlock the secrets of finding length and width using only the perimeter!
Understanding the Fundamentals: Perimeter and Rectangles
Before diving into the calculations, let's solidify our understanding of the key concepts:
What is Perimeter?
The perimeter of any two-dimensional shape is the total distance around its exterior. Imagine walking around the edges of a rectangle; the total distance covered represents its perimeter. For a rectangle, this is simply the sum of all four sides.
Rectangles: A Special Case
Rectangles are quadrilaterals (four-sided shapes) with four right angles (90-degree angles). A key characteristic of rectangles is that opposite sides are equal in length. This property is crucial for calculating length and width from the perimeter.
Formula and Equation: The Heart of the Calculation
The fundamental formula for the perimeter (P) of a rectangle is:
P = 2(length + width)
or, more concisely:
P = 2l + 2w
where:
- P represents the perimeter
- l represents the length
- w represents the width
This simple equation is the cornerstone of our calculations. To find the length and width, we need to manipulate this equation, often requiring additional information.
Scenario 1: Knowing the Perimeter and the Ratio of Length to Width
This is the most common and often the easiest scenario. Let's say we know the perimeter and the relationship between the length and width.
Example:
A rectangular garden has a perimeter of 40 meters. The length is twice the width (l = 2w). Find the length and width.
Solution:
-
Substitute: We know P = 40 and l = 2w. Substitute these values into the perimeter formula:
40 = 2(2w + w)
-
Simplify: Simplify the equation:
40 = 2(3w) 40 = 6w
-
Solve for w: Divide both sides by 6 to solve for the width:
w = 40/6 = 20/3 meters (approximately 6.67 meters)
-
Solve for l: Since l = 2w, substitute the value of w:
l = 2 * (20/3) = 40/3 meters (approximately 13.33 meters)
Therefore, the width is approximately 6.67 meters, and the length is approximately 13.33 meters.
Scenario 2: Knowing the Perimeter and the Difference Between Length and Width
In this scenario, we are given the perimeter and the difference between the length and width.
Example:
A rectangular swimming pool has a perimeter of 70 feet. The length is 5 feet longer than the width (l = w + 5). Find the length and width.
Solution:
-
Substitute: Substitute P = 70 and l = w + 5 into the perimeter formula:
70 = 2(w + 5 + w)
-
Simplify: Simplify the equation:
70 = 2(2w + 5) 70 = 4w + 10
-
Isolate w: Subtract 10 from both sides:
60 = 4w
-
Solve for w: Divide both sides by 4:
w = 15 feet
-
Solve for l: Substitute the value of w into l = w + 5:
l = 15 + 5 = 20 feet
Therefore, the width is 15 feet, and the length is 20 feet.
Scenario 3: Knowing the Perimeter and the Area
This scenario is more complex and requires solving a quadratic equation. We need to use both the perimeter and area formulas.
Example:
A rectangular field has a perimeter of 50 meters and an area of 150 square meters. Find the length and width.
Solution:
-
Perimeter Equation: We have P = 2l + 2w = 50. Simplify to l + w = 25.
-
Area Equation: We have Area = lw = 150.
-
Solve the System of Equations: We can solve this system using substitution or elimination. Let's use substitution. From the perimeter equation, we can express l as l = 25 - w.
-
Substitute and Solve: Substitute this expression for l into the area equation:
(25 - w)w = 150
-
Expand and Rearrange: Expand and rearrange into a quadratic equation:
25w - w² = 150 w² - 25w + 150 = 0
-
Factor the Quadratic: Factor the quadratic equation:
(w - 10)(w - 15) = 0
-
Solve for w: This gives two possible solutions for w: w = 10 or w = 15.
-
Solve for l:
- If w = 10, then l = 25 - 10 = 15
- If w = 15, then l = 25 - 15 = 10
Therefore, the dimensions of the rectangular field are 10 meters by 15 meters.
Advanced Scenarios and Considerations
While the above scenarios cover the most common situations, several other factors can complicate the process:
-
Units of Measurement: Always ensure consistent units throughout your calculations. Converting between units (e.g., feet to meters) is crucial for accurate results.
-
Real-World Applications: When dealing with real-world problems, consider factors like tolerances and measurement errors. Measurements are rarely perfectly precise.
-
Shapes Similar to Rectangles: While we've focused on rectangles, similar principles can be applied to other shapes with known relationships between their sides.
-
Using Algebraic Techniques: For more complex scenarios involving multiple variables or constraints, algebraic techniques, such as systems of equations, might be necessary. These techniques often involve substitution or elimination to solve for the unknowns.
Troubleshooting Common Mistakes
-
Incorrect Formula: Double-check that you're using the correct perimeter formula for a rectangle.
-
Algebraic Errors: Carefully review your algebraic steps to ensure you haven't made any mistakes in simplification, substitution, or solving equations.
-
Units: Ensure consistent units throughout your calculations. Inconsistent units will lead to incorrect results.
-
Interpreting Solutions: If you obtain multiple solutions, carefully consider which solution makes sense in the context of the problem (e.g., negative lengths are physically impossible).
Conclusion: Mastering Perimeter Calculations
Finding the length and width of a rectangle using its perimeter is a fundamental skill in geometry and problem-solving. By understanding the basic formula and applying the appropriate techniques based on the given information, you can effectively solve a wide range of problems. Remember to always double-check your work, pay attention to units, and consider the context of the problem. With practice and a systematic approach, you'll master this essential skill and confidently tackle any perimeter-related challenges. The key is to break down the problem into manageable steps, using your knowledge of algebra and geometry to arrive at the correct solution. Remember to always visualize the problem; drawing a diagram can be incredibly helpful!
Latest Posts
Related Post
Thank you for visiting our website which covers about How To Find Length And Width With Perimeter . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.