How To Find The Solution Of The Equation
News Co
Apr 07, 2025 · 5 min read
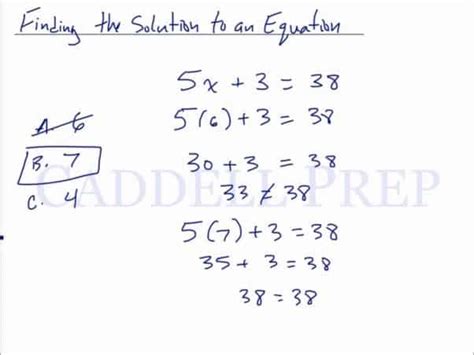
Table of Contents
How to Find the Solution of an Equation: A Comprehensive Guide
Solving equations is a fundamental skill in mathematics, applicable across various fields from basic algebra to advanced calculus. This comprehensive guide explores diverse methods for finding solutions to equations, catering to different equation types and complexity levels. We'll cover strategies for linear, quadratic, cubic, and other polynomial equations, as well as techniques for tackling equations involving trigonometric functions, logarithms, and exponentials. Mastering these techniques will empower you to tackle a wide range of mathematical problems effectively.
Understanding Equations and Solutions
Before diving into specific solution methods, let's establish a clear understanding of what constitutes an equation and its solution. An equation is a mathematical statement asserting the equality of two expressions. These expressions typically contain variables (unknown quantities represented by letters like x, y, z) and constants (known numerical values). A solution (or root) to an equation is a value or set of values for the variables that makes the equation true.
For example, in the equation 2x + 3 = 7, the solution is x = 2, because substituting x = 2 into the equation results in a true statement: 2(2) + 3 = 7.
Solving Linear Equations
Linear equations are equations of the form ax + b = c, where a, b, and c are constants, and x is the variable. Solving these equations involves isolating the variable x using algebraic manipulations.
Steps to Solve Linear Equations:
- Simplify both sides: Combine like terms on each side of the equation.
- Isolate the variable term: Add or subtract constants to move the term containing x to one side of the equation.
- Solve for the variable: Multiply or divide both sides by the coefficient of x to isolate x.
Example: Solve 3x + 5 = 11
- Subtract 5 from both sides: 3x = 6
- Divide both sides by 3: x = 2
Solving Quadratic Equations
Quadratic equations are equations of the form ax² + bx + c = 0, where a, b, and c are constants, and a ≠ 0. Several methods exist for solving quadratic equations:
1. Factoring: If the quadratic expression can be factored, set each factor to zero and solve for x.
Example: Solve x² + 5x + 6 = 0
(x + 2)(x + 3) = 0 x + 2 = 0 or x + 3 = 0 x = -2 or x = -3
2. Quadratic Formula: The quadratic formula provides a general solution for any quadratic equation:
x = [-b ± √(b² - 4ac)] / 2a
Example: Solve 2x² - 5x + 2 = 0
a = 2, b = -5, c = 2 x = [5 ± √((-5)² - 4(2)(2))] / (2 * 2) x = [5 ± √9] / 4 x = (5 ± 3) / 4 x = 2 or x = 1/2
3. Completing the Square: This method involves manipulating the equation to create a perfect square trinomial, which can then be factored easily.
Example: Solve x² + 6x + 5 = 0
- Move the constant term to the right side: x² + 6x = -5
- Take half of the coefficient of x (6/2 = 3), square it (3² = 9), and add it to both sides: x² + 6x + 9 = 4
- Factor the left side as a perfect square: (x + 3)² = 4
- Take the square root of both sides: x + 3 = ±2
- Solve for x: x = -1 or x = -5
Solving Cubic and Higher-Degree Polynomial Equations
Solving cubic and higher-degree polynomial equations can be significantly more challenging. Methods include:
1. Factoring: Similar to quadratic equations, if the polynomial can be factored, you can set each factor to zero and solve for x.
2. Rational Root Theorem: This theorem helps identify potential rational roots (roots that are fractions).
3. Numerical Methods: For equations that are difficult or impossible to solve algebraically, numerical methods like the Newton-Raphson method can provide approximate solutions.
4. Using Software: Mathematical software packages can solve polynomial equations of higher degrees efficiently.
Solving Equations Involving Other Functions
Equations involving trigonometric functions (sin, cos, tan), logarithms, and exponentials require specialized techniques:
1. Trigonometric Equations: These often involve using trigonometric identities to simplify the equation and then solving for the angle.
Example: Solve sin(x) = 1/2
x = π/6 + 2πk or x = 5π/6 + 2πk, where k is an integer.
2. Logarithmic Equations: Utilize logarithm properties to simplify the equation and solve for the variable.
Example: Solve log₂(x) = 3
x = 2³ = 8
3. Exponential Equations: Often solved by taking logarithms of both sides of the equation.
Example: Solve 2ˣ = 8
Take the logarithm base 2 of both sides: x = log₂(8) = 3
Systems of Equations
Solving systems of equations involves finding values for multiple variables that satisfy multiple equations simultaneously. Common methods include:
1. Substitution: Solve one equation for one variable and substitute the expression into the other equation.
2. Elimination: Multiply equations by constants to eliminate one variable and then solve for the remaining variable.
Tips for Successful Equation Solving
- Practice Regularly: The more you practice, the better you'll become at identifying the appropriate solution method.
- Check Your Solutions: Always substitute your solutions back into the original equation to verify their accuracy.
- Understand the Underlying Concepts: A strong grasp of fundamental mathematical concepts will greatly aid your equation-solving abilities.
- Use Visual Aids: Graphs and diagrams can help visualize the problem and solutions.
- Break Down Complex Equations: Divide complex equations into simpler, more manageable parts.
- Explore Different Approaches: If one method isn't working, try a different technique.
Conclusion
Solving equations is a multifaceted skill requiring a diverse toolkit of techniques. By mastering the methods outlined in this guide, you'll be well-equipped to tackle a wide array of equations, from simple linear equations to complex systems involving various functions. Remember that consistent practice and a solid understanding of underlying principles are key to success in equation solving. Continue to challenge yourself with increasingly complex problems, and you'll witness a significant improvement in your mathematical abilities. The journey of mastering equation solving is continuous; embrace the learning process, and your skills will inevitably grow.
Latest Posts
Related Post
Thank you for visiting our website which covers about How To Find The Solution Of The Equation . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.