How To Make A Perfect Square
News Co
Apr 06, 2025 · 6 min read
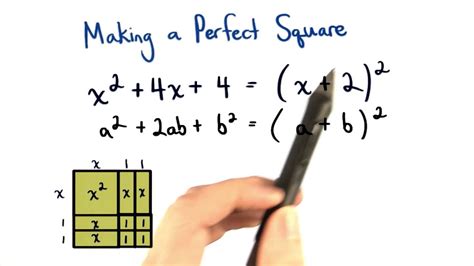
Table of Contents
How to Make a Perfect Square: A Comprehensive Guide
Creating a perfect square might sound deceptively simple, but the process can be surprisingly nuanced depending on your context. This comprehensive guide will explore various interpretations of "making a perfect square," catering to different fields like geometry, algebra, and even cooking! We'll delve into each approach, providing you with practical steps and insightful explanations.
Understanding the Concept of a "Perfect Square"
Before diving into the methods, let's clarify what constitutes a perfect square in different domains.
1. Geometric Perfect Squares:
In geometry, a perfect square is a two-dimensional shape with four equal sides and four right angles (90-degree angles). Its defining characteristics are equality of sides and angles. Constructing a perfect square geometrically requires precision and the right tools.
2. Algebraic Perfect Squares:
In algebra, a perfect square is a number or expression that can be obtained by squaring another number or expression. For example, 9 is a perfect square because it's the square of 3 (3 x 3 = 9). Similarly, x² + 6x + 9 is a perfect square trinomial because it can be factored as (x + 3)². Recognizing and manipulating perfect squares is crucial in simplifying algebraic equations and solving quadratic equations.
3. Perfect Squares in Other Contexts:
The concept of a "perfect square" can extend beyond these core definitions. For example, in cooking, arranging food items in a perfect square might refer to achieving a visually appealing and symmetrical presentation. The "perfection" depends entirely on the context.
Methods for Making a Perfect Square:
Let's now explore different approaches to creating perfect squares, focusing primarily on geometric and algebraic contexts.
I. Creating a Geometric Perfect Square:
Several methods exist for constructing a geometric perfect square. We'll examine two common approaches:
A. Using a Ruler and a Protractor:
This is the most straightforward method, ideal for precise constructions.
-
Determine the side length: Decide on the desired side length of your square. Let's say it's 5 centimeters.
-
Draw the first side: Use a ruler to draw a straight line segment of 5 cm. This will be one side of your square.
-
Construct the right angles: Place the protractor at one end of the line segment. Mark a 90-degree angle. Draw a second line segment of 5 cm from the end of the first segment, ensuring it forms a perfect right angle.
-
Complete the square: Repeat step 3 at the other end of the first line segment, creating another 5 cm line segment that forms a 90-degree angle. Finally, connect the ends of the second and third line segments with a 5 cm line segment. You now have a perfect square!
Important Considerations: The accuracy of this method relies heavily on the precision of your ruler and protractor. Small errors in measurement can lead to noticeable deviations from a perfect square.
B. Using a Compass and Straightedge:
This classic geometric construction method relies only on a compass and a straightedge. It’s a more elegant approach demonstrating fundamental geometric principles.
-
Draw a line segment: Draw a straight line segment of your desired length (let's call it 'a'). This will be one side of the square.
-
Construct a perpendicular: At one end of the line segment, use your compass to draw a circle with a radius larger than half the length of the line segment. Then, using the same radius, draw another circle with its center at the other end of the line segment. The intersection points of the two circles will determine a line perpendicular to the initial segment.
-
Mark the side length: Use your compass to measure the length 'a' along the perpendicular line you've constructed. Mark this point.
-
Complete the square: Draw a line segment of length 'a' from the intersection point (step 2) to the marked point on the perpendicular line (step 3). Similarly, draw a line segment of length 'a' from the other end of the initial line segment to the same point you marked on the perpendicular line. These segments will complete your square.
This method, though more complex, offers a higher degree of accuracy if executed carefully. It avoids the potential inaccuracies associated with measuring angles with a protractor.
II. Creating an Algebraic Perfect Square:
In algebra, creating a perfect square often involves completing the square or recognizing perfect square trinomials.
A. Completing the Square:
This technique is essential for solving quadratic equations and manipulating algebraic expressions. Consider the expression x² + 6x. To make it a perfect square, we follow these steps:
-
Take half of the coefficient of x: The coefficient of x is 6. Half of 6 is 3.
-
Square the result: Squaring 3 gives us 9.
-
Add and subtract the result: Add 9 to the expression and subtract it immediately afterwards to maintain its value. This gives us: x² + 6x + 9 - 9
-
Factor the perfect square trinomial: The expression x² + 6x + 9 is a perfect square trinomial and factors to (x + 3)². Therefore, the complete expression is (x + 3)² - 9.
B. Recognizing Perfect Square Trinomials:
A perfect square trinomial is an expression that can be factored into the square of a binomial. Identifying them is vital for simplification and problem-solving. Here's how to recognize one:
- The first term is a perfect square: e.g., x², 4y², 9a²
- The last term is a perfect square: e.g., 25, 16z², 1
- The middle term is twice the product of the square roots of the first and last terms: For instance, in (x + 5)², which expands to x² + 10x + 25, the middle term (10x) is twice the product of the square roots of x² and 25 (2 * x * 5 = 10x).
Mastering the ability to recognize and factor perfect square trinomials is a cornerstone of algebraic proficiency.
III. Creating a "Perfect Square" in Other Contexts:
As mentioned earlier, the concept of "perfect square" can be interpreted broadly.
In cooking, achieving a perfectly square presentation could involve using square baking dishes, cutting ingredients into uniform squares, or strategically arranging elements on a plate to create a visually pleasing square pattern. The "perfection" here is subjective and depends on individual aesthetic preferences.
In software development, creating a "perfect square" could refer to arranging UI elements or data structures in a grid format with equally spaced elements, maintaining consistency and visual appeal.
Troubleshooting Common Issues:
Even with meticulous planning, creating a perfect square can present challenges. Here are some common problems and their solutions:
-
Inaccurate Measurements (Geometry): Use high-quality tools and double-check your measurements. Consider using digital calipers for precise measurements.
-
Incorrect Angle Measurement (Geometry): Ensure your protractor is properly positioned and read the angles carefully.
-
Difficulty Factoring (Algebra): Practice factoring regularly. Work through numerous examples to build your skill and familiarity with recognizing perfect square trinomials.
-
Visual Imperfection (Cooking/Design): Utilize tools like rulers, templates, or grid systems to aid in achieving a precise square shape.
Conclusion:
Creating a perfect square, regardless of the context, requires attention to detail, precision, and an understanding of the underlying principles. Whether you're meticulously constructing a geometric square, deftly manipulating algebraic expressions, or striving for visual perfection in your culinary creations, the methods outlined above provide a robust foundation for success. Mastering the techniques for creating perfect squares empowers you to achieve accuracy, efficiency, and aesthetic excellence in diverse fields. Remember to practice regularly and refine your skills to become an expert in constructing perfect squares!
Latest Posts
Related Post
Thank you for visiting our website which covers about How To Make A Perfect Square . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.