How To Write In Logarithmic Form
News Co
Apr 07, 2025 · 6 min read
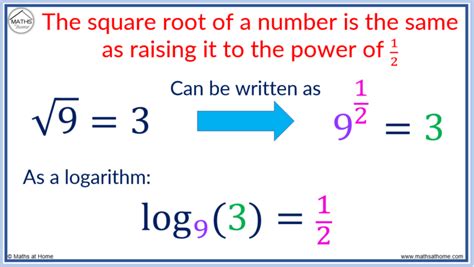
Table of Contents
How to Write in Logarithmic Form: A Comprehensive Guide
Understanding logarithms is crucial for various fields, from mathematics and science to finance and computer science. This comprehensive guide will delve into the intricacies of logarithmic form, explaining its fundamental principles, different bases, practical applications, and how to effectively translate between logarithmic and exponential forms. We'll also explore some common misconceptions and offer tips for mastering this essential mathematical concept.
Understanding Logarithms: The Basics
A logarithm is essentially the inverse operation of exponentiation. In simpler terms, if you have an equation like b<sup>x</sup> = y, where 'b' is the base, 'x' is the exponent, and 'y' is the result, the logarithmic equivalent is log<sub>b</sub>y = x. This reads as "the logarithm of y to the base b is x".
Key Components:
- Base (b): This is the number that is raised to a power. The base must be a positive number, and it cannot be 1. Common bases include 10 (common logarithm), e (natural logarithm), and 2 (binary logarithm).
- Argument (y): This is the number whose logarithm you are calculating. It must be a positive number.
- Exponent (x): This is the power to which the base must be raised to obtain the argument. The exponent can be any real number.
Common Logarithms and Natural Logarithms
Two specific types of logarithms deserve special attention:
Common Logarithms (Base 10)
Common logarithms use a base of 10. They are often written without explicitly stating the base, as log y. For example, log 100 = 2 because 10<sup>2</sup> = 100.
Natural Logarithms (Base e)
Natural logarithms use the mathematical constant e (approximately 2.71828) as their base. They are denoted as ln y. For example, ln e = 1 because e<sup>1</sup> = e. Natural logarithms are frequently encountered in calculus and many scientific applications.
Converting Between Exponential and Logarithmic Forms
The ability to seamlessly switch between exponential and logarithmic forms is paramount. Here's a step-by-step guide:
1. Exponential to Logarithmic:
Let's say you have the exponential equation: b<sup>x</sup> = y
To convert it to logarithmic form, follow these steps:
- Identify the base (b), the exponent (x), and the result (y).
- Write the logarithmic equation:
log<sub>b</sub>y = x
Example: 2<sup>3</sup> = 8 becomes log<sub>2</sub>8 = 3
2. Logarithmic to Exponential:
Now, let's convert a logarithmic equation to its exponential counterpart. Start with: log<sub>b</sub>y = x
- Identify the base (b), the argument (y), and the exponent (x).
- Write the exponential equation:
b<sup>x</sup> = y
Example: log<sub>5</sub>25 = 2 becomes 5<sup>2</sup> = 25
Properties of Logarithms
Understanding the properties of logarithms is critical for simplifying complex expressions and solving logarithmic equations. These properties are derived directly from the properties of exponents:
- Product Rule:
log<sub>b</sub>(xy) = log<sub>b</sub>x + log<sub>b</sub>y - Quotient Rule:
log<sub>b</sub>(x/y) = log<sub>b</sub>x - log<sub>b</sub>y - Power Rule:
log<sub>b</sub>(x<sup>p</sup>) = p * log<sub>b</sub>x - Change of Base Formula:
log<sub>a</sub>x = (log<sub>b</sub>x) / (log<sub>b</sub>a)This is particularly useful when you need to calculate a logarithm with a base that your calculator doesn't directly support. - Logarithm of 1:
log<sub>b</sub>1 = 0(because b<sup>0</sup> = 1) - Logarithm of the Base:
log<sub>b</sub>b = 1(because b<sup>1</sup> = b)
Solving Logarithmic Equations
Solving logarithmic equations involves manipulating the equation using the properties of logarithms to isolate the variable. Here are some strategies:
- Use the properties of logarithms to simplify the equation. Combine or separate logarithmic terms using the product, quotient, and power rules.
- Convert the equation to exponential form if necessary. This often simplifies the equation and makes it easier to solve.
- Isolate the variable. Perform algebraic operations (addition, subtraction, multiplication, division) to isolate the variable on one side of the equation.
- Check your solution. Substitute your solution back into the original equation to ensure it's correct. Remember that the argument of a logarithm must always be positive.
Practical Applications of Logarithms
Logarithms have numerous practical applications across diverse fields:
- Chemistry (pH scale): The pH scale, which measures the acidity or alkalinity of a solution, is a logarithmic scale.
- Physics (decibels): Sound intensity is measured in decibels (dB), a logarithmic unit.
- Earthquake measurement (Richter scale): The Richter scale, used to measure the magnitude of earthquakes, is also a logarithmic scale.
- Finance (compound interest): Logarithms are used to calculate the time it takes for an investment to grow to a certain value.
- Computer science (algorithms): Logarithmic complexity is an important concept in analyzing the efficiency of algorithms.
Common Mistakes to Avoid
Several common mistakes can hinder your understanding and application of logarithms:
- Incorrectly applying the logarithm properties: Carefully review and understand each property before applying it. Common errors include incorrect manipulation of the product, quotient, and power rules.
- Forgetting the restrictions on the argument and base: Remember that the base must be positive and not equal to 1, and the argument must be positive.
- Misinterpreting the notation: Pay close attention to the base of the logarithm. The absence of a base typically implies a base of 10 (common logarithm) or e (natural logarithm).
- Ignoring the order of operations: Follow the standard order of operations (PEMDAS/BODMAS) when evaluating logarithmic expressions.
Mastering Logarithmic Form: Tips and Practice
Mastering logarithms requires consistent practice. Here are some tips to improve your understanding and skills:
- Start with the basics: Ensure you have a solid grasp of exponential functions before tackling logarithms.
- Practice converting between exponential and logarithmic forms: This is fundamental to understanding logarithms.
- Work through examples: Solve a variety of problems to build your confidence and identify areas where you need further practice.
- Use online resources: Numerous websites and online calculators can help you check your work and learn more about logarithms.
- Seek help when needed: Don't hesitate to ask for help from a teacher, tutor, or online community if you're struggling with a particular concept.
Conclusion
Logarithms, while initially appearing complex, become significantly more manageable with a systematic approach. By understanding the fundamental principles, mastering the conversion between exponential and logarithmic forms, applying the properties correctly, and practicing regularly, you can confidently navigate the world of logarithmic functions and apply them across various fields. Remember to always check your work and pay close attention to detail – the rewards of mastering this crucial mathematical concept are substantial.
Latest Posts
Related Post
Thank you for visiting our website which covers about How To Write In Logarithmic Form . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.