Is 12 A Multiple Of 4
News Co
May 08, 2025 · 4 min read
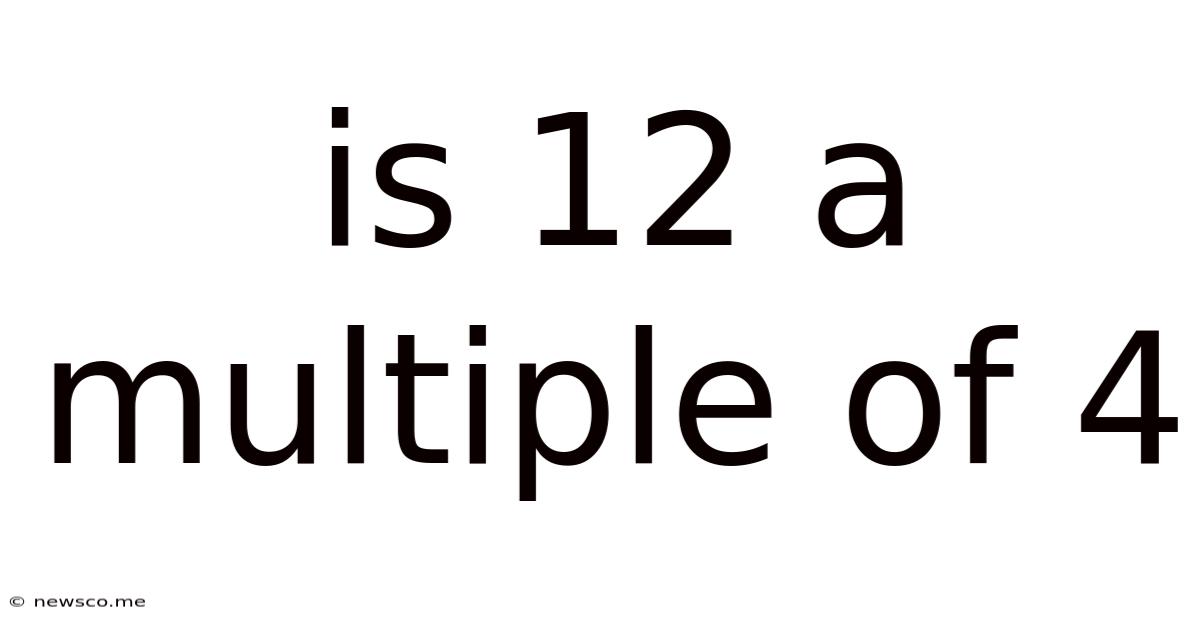
Table of Contents
Is 12 a Multiple of 4? A Deep Dive into Multiplication and Divisibility
The seemingly simple question, "Is 12 a multiple of 4?" opens a door to a fascinating exploration of fundamental mathematical concepts. While the answer itself is straightforward, understanding why it's true provides a solid foundation for grasping more complex mathematical ideas. This article will delve into the intricacies of multiples, divisibility, and related concepts, providing a comprehensive understanding for both beginners and those seeking a refresher.
Understanding Multiples
Before tackling the core question, let's define what a multiple is. A multiple of a number is the product of that number and any integer (whole number). So, multiples of 4 are the results you get when you multiply 4 by any whole number: 0, 1, 2, 3, and so on.
Examples of Multiples of 4:
- 4 x 0 = 0: Zero is a multiple of every number.
- 4 x 1 = 4: Four is the first multiple of 4 (excluding zero).
- 4 x 2 = 8: Eight is the second multiple of 4.
- 4 x 3 = 12: Twelve is the third multiple of 4.
- 4 x 4 = 16: Sixteen is the fourth multiple of 4.
- 4 x 5 = 20: And so on...
This pattern continues infinitely in both positive and negative directions. The set of multiples of 4 is {..., -16, -12, -8, -4, 0, 4, 8, 12, 16, ...}.
Understanding Divisibility
Divisibility is closely linked to the concept of multiples. A number is divisible by another number if the division results in a whole number (no remainder). This is equivalent to saying that the first number is a multiple of the second.
Divisibility Rules
Certain divisibility rules can quickly determine if a number is divisible by another without performing the actual division. For the number 4, the divisibility rule is:
- A number is divisible by 4 if its last two digits are divisible by 4.
Let's test this rule with some examples:
- 12: The last two digits are "12," and 12 is divisible by 4 (12/4 = 3). Therefore, 12 is divisible by 4.
- 28: The last two digits are "28," and 28 is divisible by 4 (28/4 = 7). Therefore, 28 is divisible by 4.
- 35: The last two digits are "35," and 35 is not divisible by 4. Therefore, 35 is not divisible by 4.
Answering the Question: Is 12 a Multiple of 4?
Now, armed with our understanding of multiples and divisibility, we can definitively answer the question: Yes, 12 is a multiple of 4.
This is because:
- 12 is divisible by 4: 12 divided by 4 equals 3 (a whole number).
- 12 can be expressed as a product of 4 and an integer: 12 = 4 x 3. Here, 3 is the integer.
Therefore, 12 fits the definition of being a multiple of 4.
Exploring Further: Prime Factorization and the Fundamental Theorem of Arithmetic
Understanding multiples and divisibility opens the door to more advanced mathematical concepts. One such concept is prime factorization. Every integer greater than 1 can be represented as a unique product of prime numbers (numbers divisible only by 1 and themselves). This is known as the Fundamental Theorem of Arithmetic.
Let's find the prime factorization of 12:
12 = 2 x 6 = 2 x 2 x 3 = 2² x 3
The prime factorization of 4 is:
4 = 2 x 2 = 2²
Notice that the prime factorization of 4 (2²) is a factor of the prime factorization of 12 (2² x 3). This is another way to confirm that 12 is a multiple of 4. If the prime factorization of one number contains all the prime factors of another number, then the first number is a multiple of the second.
Applications in Real Life
Understanding multiples and divisibility isn't just confined to the classroom; it has numerous practical applications in everyday life. Consider these examples:
- Sharing Equally: If you have 12 cookies and want to share them equally among 4 friends, you can easily do so because 12 is a multiple of 4. Each friend gets 3 cookies.
- Measurement Conversions: Many measurement conversions involve multiples. For example, converting inches to feet (12 inches = 1 foot) relies on the fact that 12 is a multiple of 4 (and also 3).
- Time Management: Dividing a 12-hour period into 4 equal parts (3 hours each) uses the concept of divisibility.
- Pattern Recognition: Multiples are frequently seen in patterns and sequences. Recognizing these patterns can be helpful in various problem-solving situations.
Conclusion: Beyond the Basic Answer
The question, "Is 12 a multiple of 4?" is more than just a simple arithmetic problem. It's a gateway to a deeper understanding of fundamental mathematical concepts like multiples, divisibility, prime factorization, and their practical applications. Mastering these concepts provides a strong foundation for more advanced mathematical studies and problem-solving in various fields. By thoroughly understanding these concepts, you can confidently tackle more complex mathematical challenges and appreciate the interconnectedness of seemingly simple mathematical ideas. The answer is a resounding yes, but the journey to understanding why is where the true mathematical learning takes place.
Latest Posts
Related Post
Thank you for visiting our website which covers about Is 12 A Multiple Of 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.