Is 30 A Prime Or Composite Number
News Co
Apr 04, 2025 · 5 min read
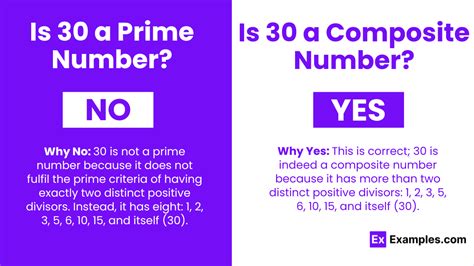
Table of Contents
Is 30 a Prime or Composite Number? A Deep Dive into Number Theory
Determining whether a number is prime or composite is a fundamental concept in number theory. This seemingly simple question – is 30 a prime or composite number? – opens the door to exploring the fascinating world of prime factorization, divisibility rules, and the properties of prime and composite numbers. This article will not only answer the question definitively but also delve into the underlying principles, providing a comprehensive understanding of the topic.
Understanding Prime and Composite Numbers
Before we tackle the specific case of 30, let's define our terms:
-
Prime Number: A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. In simpler terms, it's only divisible by 1 and itself. Examples include 2, 3, 5, 7, 11, and so on.
-
Composite Number: A composite number is a positive integer that has at least one positive divisor other than 1 and itself. Essentially, it can be factored into smaller positive integers. Examples include 4 (2 x 2), 6 (2 x 3), 9 (3 x 3), and many more.
-
Neither Prime nor Composite: The number 1 is neither prime nor composite. This is a special case and is often excluded from discussions about prime and composite numbers.
Determining if 30 is Prime or Composite
Now, let's apply these definitions to the number 30. To determine whether 30 is prime or composite, we need to check if it has any divisors other than 1 and 30.
Let's list the factors of 30:
1, 2, 3, 5, 6, 10, 15, 30
As you can see, 30 has several divisors besides 1 and 30 (e.g., 2, 3, 5, 6, 10, 15). Therefore, 30 is a composite number.
Prime Factorization of 30
A crucial concept related to composite numbers is prime factorization. This involves expressing a composite number as a product of its prime factors. For 30, the prime factorization is:
30 = 2 x 3 x 5
This means that 30 can be broken down into the product of the prime numbers 2, 3, and 5. This prime factorization is unique to 30; no other set of prime numbers will multiply to give 30. This uniqueness is a cornerstone of many theorems in number theory.
Divisibility Rules and Their Application to 30
Divisibility rules provide quick ways to determine if a number is divisible by a particular prime number. Let's examine how these rules apply to 30:
-
Divisibility by 2: A number is divisible by 2 if its last digit is even (0, 2, 4, 6, or 8). Since the last digit of 30 is 0, it's divisible by 2.
-
Divisibility by 3: A number is divisible by 3 if the sum of its digits is divisible by 3. The sum of the digits of 30 (3 + 0 = 3) is divisible by 3, confirming its divisibility by 3.
-
Divisibility by 5: A number is divisible by 5 if its last digit is 0 or 5. The last digit of 30 is 0, making it divisible by 5.
These divisibility rules quickly confirm that 30 is divisible by 2, 3, and 5, further supporting the conclusion that it is a composite number.
The Significance of Prime and Composite Numbers
The distinction between prime and composite numbers is far more significant than it might initially seem. Prime numbers are the building blocks of all integers, forming the foundation of number theory. Their properties are crucial in various areas, including:
-
Cryptography: Prime numbers play a vital role in modern cryptography, particularly in public-key cryptography systems like RSA. The security of these systems relies on the difficulty of factoring large composite numbers into their prime factors.
-
Computer Science: Prime numbers are used in hash table algorithms and other data structures to improve efficiency and performance.
-
Abstract Algebra: Prime numbers are fundamental concepts in abstract algebra, contributing to the study of rings, fields, and other algebraic structures.
-
Number Theory Research: The study of prime numbers remains an active area of mathematical research, with numerous unsolved problems and ongoing investigations. For instance, the Riemann Hypothesis, one of the most important unsolved problems in mathematics, deals with the distribution of prime numbers.
Exploring Related Concepts: Twin Primes and Mersenne Primes
While we've focused on 30, let's briefly touch upon some fascinating related concepts:
-
Twin Primes: These are pairs of prime numbers that differ by 2 (e.g., 3 and 5, 5 and 7, 11 and 13). The infinitude of twin primes is a major unsolved problem in number theory.
-
Mersenne Primes: These are prime numbers of the form 2<sup>p</sup> - 1, where 'p' is also a prime number. Finding Mersenne primes has led to discoveries of the largest known prime numbers.
Practical Applications and Real-World Examples
The concept of prime and composite numbers extends beyond theoretical mathematics. Consider these examples:
-
Resource Allocation: Imagine distributing 30 identical items among groups. Understanding the factors of 30 allows you to efficiently divide these items into groups of 2, 3, 5, 6, 10, or 15.
-
Coding and Algorithms: In computer science, prime numbers are used in various algorithms to ensure data integrity and efficient operations.
-
Game Theory: Certain games and puzzles utilize prime numbers and their properties to create interesting challenges and solutions.
Conclusion: 30 – A Definitive Composite Number
In conclusion, 30 is definitively a composite number. Its divisibility by 2, 3, and 5, along with its prime factorization (2 x 3 x 5), clearly demonstrates this. Understanding the nature of prime and composite numbers, along with related concepts like prime factorization and divisibility rules, is essential for grasping fundamental mathematical principles and their applications across various fields. The seemingly simple question of whether 30 is prime or composite reveals a deeper mathematical richness, highlighting the importance of these numbers in both theoretical and practical contexts. Further exploration of these concepts will undoubtedly unveil a more profound appreciation for the intricate beauty and power of number theory.
Latest Posts
Related Post
Thank you for visiting our website which covers about Is 30 A Prime Or Composite Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.