Is 33 A Multiple Of 11
News Co
Mar 18, 2025 · 5 min read
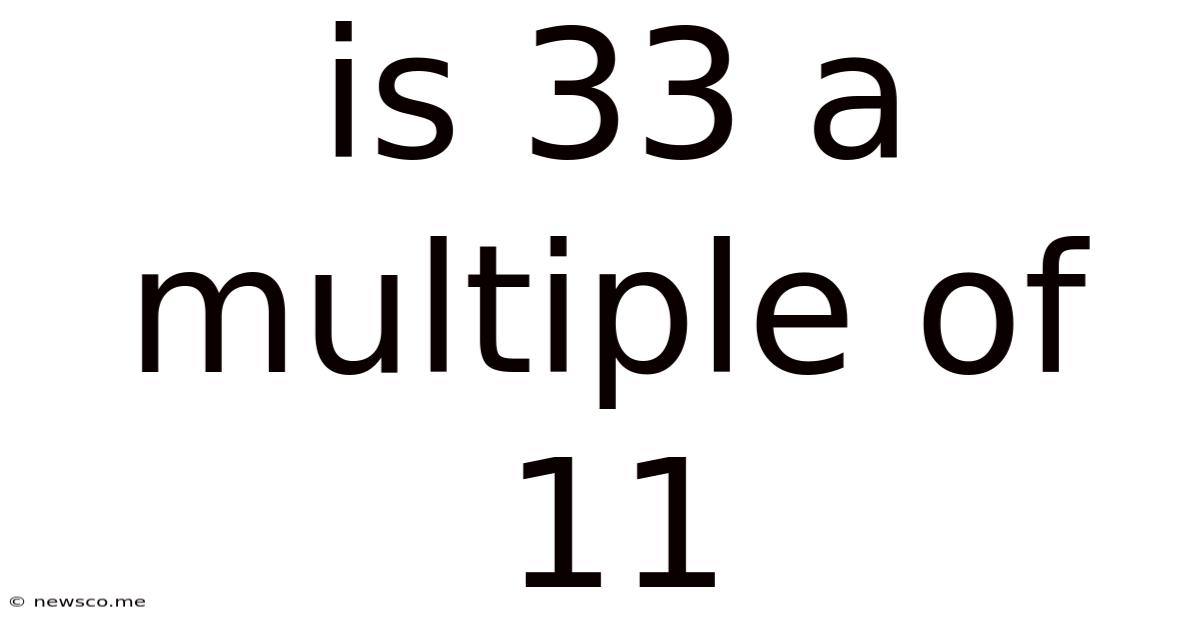
Table of Contents
Is 33 a Multiple of 11? A Deep Dive into Divisibility and Factors
The question, "Is 33 a multiple of 11?" might seem simple at first glance. For those comfortable with basic arithmetic, the answer is a straightforward "yes." However, exploring this seemingly simple question opens a door to a deeper understanding of fundamental mathematical concepts like divisibility, factors, multiples, and prime factorization. This article will not only answer the initial question but also delve into the underlying principles, offering a comprehensive explanation suitable for various levels of mathematical understanding.
Understanding Multiples and Divisibility
Before we directly address whether 33 is a multiple of 11, let's establish a clear understanding of the terms involved.
Multiple: A multiple of a number is the product of that number and any integer (whole number). For example, multiples of 2 include 2, 4, 6, 8, 10, and so on. Each of these numbers is the result of multiplying 2 by another integer (1 x 2, 2 x 2, 3 x 2, etc.).
Divisibility: Divisibility refers to the ability of one number to be divided by another number without leaving a remainder. If a number is divisible by another number, the latter is considered a factor of the former. For instance, 12 is divisible by 3 because 12 divided by 3 equals 4 with no remainder. Therefore, 3 is a factor of 12.
Factor: A factor of a number is an integer that divides the number evenly without leaving a remainder. The factors of 12 are 1, 2, 3, 4, 6, and 12.
Directly Answering the Question: Is 33 a Multiple of 11?
Yes, 33 is a multiple of 11. This is because 33 can be obtained by multiplying 11 by an integer. Specifically:
11 x 3 = 33
Therefore, 33 is the third multiple of 11. Conversely, 11 is a factor of 33, and 33 is divisible by 11.
Exploring Divisibility Rules: A Shortcut to Identifying Multiples
While simple multiplication can always determine whether a number is a multiple of another, divisibility rules offer a quicker way, especially for larger numbers. For the number 11, the divisibility rule is slightly more complex than for some other numbers but still manageable.
The divisibility rule for 11 involves alternating sums and differences of digits. Let's apply it to 33:
- Consider the digits: 3 and 3.
- Alternating sum/difference: 3 - 3 = 0
- Result: Since the result is 0 (or a multiple of 11), 33 is divisible by 11.
This rule works for larger numbers as well. For example, let's check if 121 is divisible by 11:
- Digits: 1, 2, 1
- Alternating sum/difference: 1 - 2 + 1 = 0
- Result: Since the result is 0, 121 is divisible by 11 (11 x 11 = 121).
Prime Factorization and its Relevance
Prime factorization is the process of expressing a number as the product of its prime factors. Prime numbers are numbers greater than 1 that are only divisible by 1 and themselves (e.g., 2, 3, 5, 7, 11, etc.). Prime factorization provides a fundamental understanding of a number's structure.
Let's find the prime factorization of 33:
33 can be factored as 3 x 11. Both 3 and 11 are prime numbers. This factorization clearly shows that 11 is a factor of 33, further confirming that 33 is a multiple of 11.
Practical Applications of Multiples and Divisibility
Understanding multiples and divisibility isn't just an academic exercise; it has numerous practical applications in various fields:
- Everyday Arithmetic: Dividing quantities evenly (e.g., sharing cookies among friends, arranging items in rows).
- Measurement and Conversions: Converting units (e.g., inches to feet, liters to milliliters).
- Programming: Creating loops and arrays, handling data structures efficiently.
- Scheduling and Calendars: Determining the frequency of events (e.g., monthly payments, weekly meetings).
- Engineering and Design: Designing structures and systems with balanced proportions.
Advanced Concepts Related to Multiples and Divisibility
Let's explore some slightly more advanced concepts related to multiples and divisibility:
-
Least Common Multiple (LCM): The LCM of two or more numbers is the smallest number that is a multiple of all the numbers. Finding the LCM is crucial in various applications, such as determining the frequency of cyclical events that coincide.
-
Greatest Common Divisor (GCD): The GCD of two or more numbers is the largest number that divides all the numbers without leaving a remainder. GCD is used in simplifying fractions and solving various mathematical problems.
-
Modular Arithmetic: This branch of mathematics deals with remainders after division. It has significant applications in cryptography and computer science. The concept of divisibility is central to modular arithmetic.
Conclusion: More Than Just a Simple "Yes"
While the answer to "Is 33 a multiple of 11?" is a simple "yes," the journey to reach that answer reveals a wealth of mathematical concepts. Understanding multiples, divisibility, prime factorization, and related concepts like LCM and GCD are fundamental to a strong mathematical foundation. These concepts are not limited to theoretical exercises; they have wide-ranging practical applications in various aspects of life and different fields of study. The seemingly simple question serves as a gateway to a deeper appreciation of the elegance and power of mathematics. Further exploration of these concepts will undoubtedly enrich your mathematical understanding and problem-solving skills. The ability to quickly assess divisibility is also a valuable skill for quick mental calculations and problem-solving in diverse contexts.
Latest Posts
Latest Posts
-
Find The Point On The Y Axis Which Is Equidistant From
May 09, 2025
-
Is 3 4 Bigger Than 7 8
May 09, 2025
-
Which Of These Is Not A Prime Number
May 09, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 09, 2025
-
Are Alternate Exterior Angles Always Congruent
May 09, 2025
Related Post
Thank you for visiting our website which covers about Is 33 A Multiple Of 11 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.