Is Arcsin The Same As Csc
News Co
May 07, 2025 · 4 min read
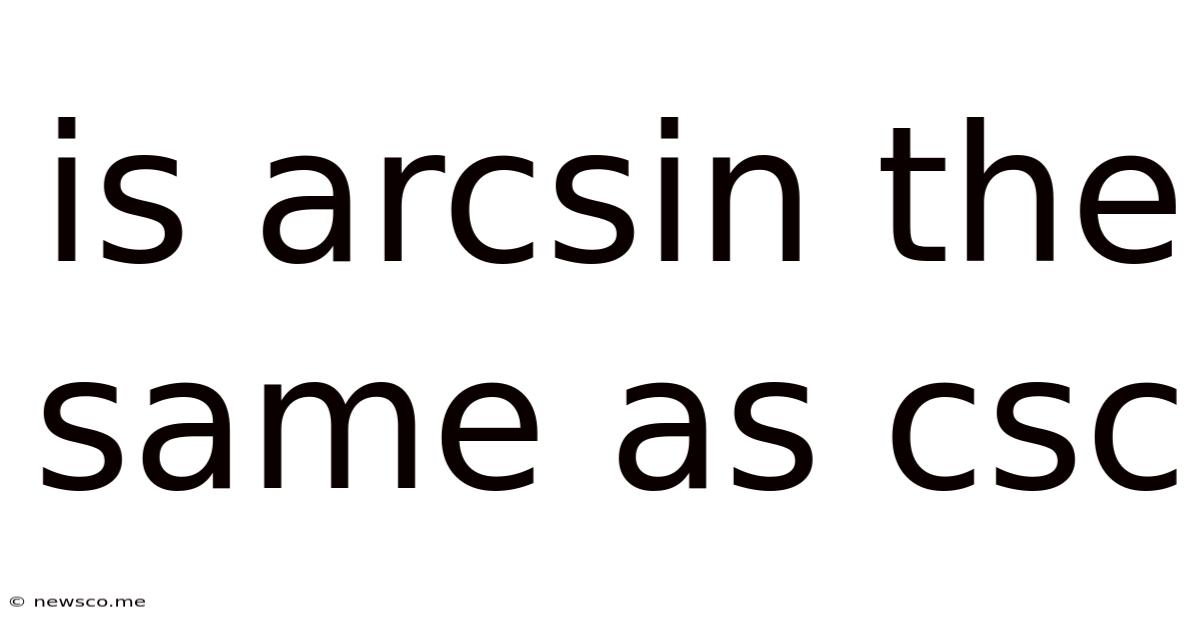
Table of Contents
Is arcsin the same as csc? Understanding Inverse Trigonometric Functions and Reciprocal Trigonometric Functions
The question "Is arcsin the same as csc?" is a common point of confusion for students studying trigonometry. While both arcsin (arcsine) and csc (cosecant) relate to the sine function, they are fundamentally different mathematical concepts. This article will delve into the distinct nature of these functions, clarifying their definitions, properties, and applications, ultimately dispelling any misconception about their equivalence.
Defining arcsin (arcsine) and csc (cosecant)
Let's start by defining each function precisely.
Understanding arcsin (arcsine)
Arcsin, often written as sin⁻¹ (but not to be confused with 1/sin), is the inverse trigonometric function of sine. It answers the question: "What angle has a sine value of x?" In simpler terms, it gives you the angle whose sine is a given value.
- Domain: [-1, 1] (The input, x, must be between -1 and 1, inclusive, because the sine of any angle is always within this range).
- Range: [-π/2, π/2] (The output, the angle, is restricted to the interval from -π/2 radians (-90 degrees) to π/2 radians (90 degrees) to ensure a single, unique output for each input. This is called the principal value).
- Example: arcsin(1/2) = π/6 (Because sin(π/6) = 1/2)
Understanding csc (cosecant)
Csc, or cosecant, is a reciprocal trigonometric function. It's the reciprocal of the sine function. This means:
csc(x) = 1/sin(x)
- Domain: All real numbers except multiples of π (where sin(x) = 0). This is because division by zero is undefined.
- Range: (-∞, -1] ∪ [1, ∞) (The cosecant function's values are always greater than or equal to 1 or less than or equal to -1).
- Example: csc(π/6) = 1/sin(π/6) = 1/(1/2) = 2
Key Differences: A Comparative Analysis
The table below summarizes the crucial distinctions between arcsin and csc:
| Feature | arcsin (arcsine) | csc (cosecant) |
|---|---|---|
| Type of Function | Inverse Trigonometric Function | Reciprocal Trigonometric Function |
| Input | A value between -1 and 1 | An angle (in radians or degrees) |
| Output | An angle (typically in radians) | A ratio (a real number) |
| Relationship to Sine | "Undoes" the sine function (finds the angle) | Is the reciprocal of the sine function (1/sin) |
| Domain | [-1, 1] | All real numbers except multiples of π |
| Range | [-π/2, π/2] | (-∞, -1] ∪ [1, ∞) |
Illustrative Examples to Highlight the Differences
Let's consider a few examples to reinforce the differences between arcsin and csc:
Example 1:
Find the value of arcsin(0.5) and csc(30°).
-
arcsin(0.5): This asks, "What angle has a sine of 0.5?" The answer is π/6 radians (or 30°).
-
csc(30°): This calculates the reciprocal of sin(30°). Since sin(30°) = 0.5, csc(30°) = 1/0.5 = 2.
Notice the different outputs: an angle for arcsin and a ratio for csc.
Example 2:
What is arcsin(csc(π/4))?
First, we find csc(π/4):
csc(π/4) = 1/sin(π/4) = 1/(√2/2) = √2
Now, we find arcsin(√2). However, this is undefined because √2 > 1, which falls outside the domain of arcsin. The domain of arcsin is only [-1,1].
Example 3:
Can we find a value x such that arcsin(x) = csc(x)?
This equation is tricky and doesn't have a simple algebraic solution. You would need to use numerical methods or graphical techniques to find approximate solutions. There will likely be multiple solutions or no solutions depending on how you define the range of the arcsin function.
Applications of arcsin and csc
Both arcsin and csc find significant applications in various fields:
Applications of arcsin
- Physics: Calculating angles of projection, angles of incidence and refraction in optics, determining the phase angle in wave phenomena.
- Engineering: Solving geometrical problems, analyzing oscillations and vibrations, determining the angles in structural designs.
- Navigation: Determining the bearing or direction using trigonometric calculations.
- Computer Graphics: Used in rotations, transformations, and rendering algorithms.
Applications of csc
- Physics: Calculating the amplitude of a wave, analyzing wave patterns.
- Engineering: Determining the maximum or minimum values of certain mechanical systems.
- Astronomy: Calculations related to celestial mechanics and determining distances.
Conclusion: arcsin and csc are distinct functions
In conclusion, while both arcsin and csc are related to the sine function, they serve entirely different purposes and possess unique properties. Arcsin is the inverse function, providing the angle corresponding to a given sine value, whereas csc is the reciprocal function, representing the ratio 1/sin. Understanding their fundamental differences is crucial for accurate calculations and proper application in various mathematical and scientific contexts. Confusing these functions would lead to incorrect results and a fundamental misunderstanding of trigonometric operations. Therefore, it's crucial to clearly differentiate between these two distinct mathematical concepts.
Latest Posts
Latest Posts
-
Which Is Greater 1500 Ml Or 1 5 L
May 09, 2025
-
Find The Value Of X And Y In Each Figure
May 09, 2025
-
20 To The Power Of 20
May 09, 2025
-
Calculate The Current In The Circuit In The Figure
May 09, 2025
-
Does Rhombus Have 4 Equal Sides
May 09, 2025
Related Post
Thank you for visiting our website which covers about Is Arcsin The Same As Csc . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.