Is Square Root Of 100 A Rational Number
News Co
Mar 26, 2025 · 4 min read
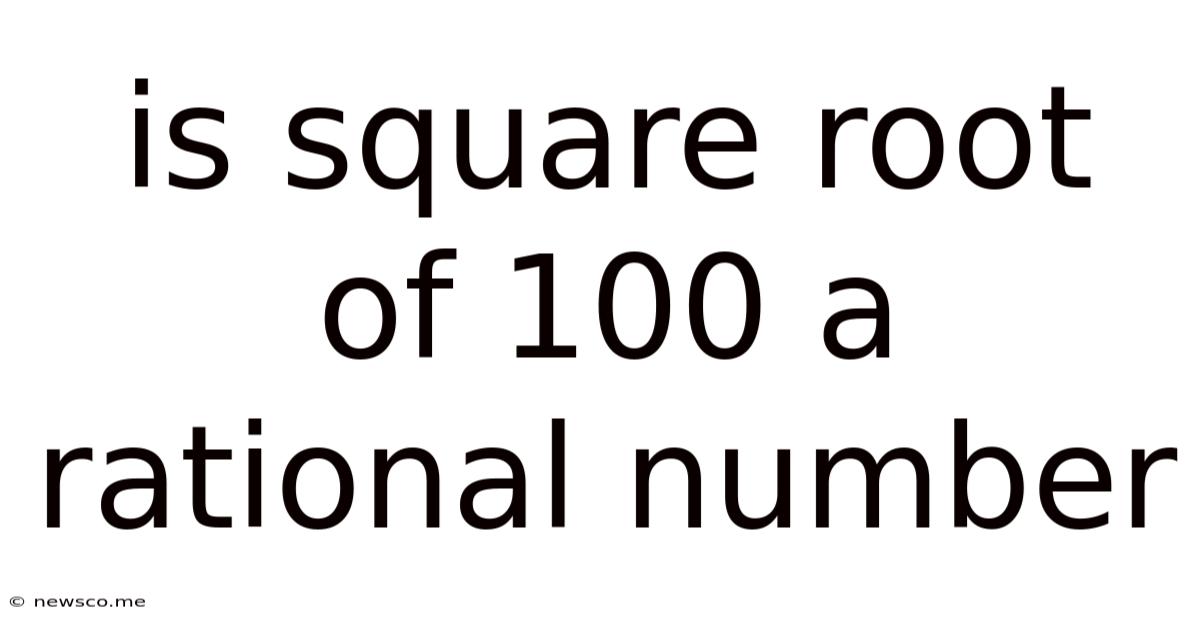
Table of Contents
Is the Square Root of 100 a Rational Number? A Deep Dive into Number Theory
The question, "Is the square root of 100 a rational number?" might seem simple at first glance. However, understanding the answer requires a firm grasp of fundamental number theory concepts. This article will not only answer this specific question definitively but will also explore the broader context of rational and irrational numbers, providing a comprehensive understanding for both beginners and those seeking a deeper dive.
Understanding Rational Numbers
A rational number is any number that can be expressed as a fraction p/q, where 'p' and 'q' are integers, and 'q' is not equal to zero. This seemingly simple definition encompasses a vast range of numbers. Examples of rational numbers include:
-
Integers: All whole numbers, both positive and negative, including zero, are rational. For example, 5 can be expressed as 5/1, -3 as -3/1, and 0 as 0/1.
-
Fractions: Numbers expressed as ratios of integers, like 1/2, 3/4, -2/5, are quintessential examples of rational numbers.
-
Terminating Decimals: Decimal numbers that end after a finite number of digits are rational. For example, 0.75 (which is 3/4) and 2.5 (which is 5/2) are rational.
-
Repeating Decimals: Decimal numbers where a sequence of digits repeats infinitely are also rational. For example, 0.333... (which is 1/3) and 0.142857142857... (which is 1/7) are rational.
The key takeaway is that any number that can be precisely represented as a ratio of two integers falls under the umbrella of rational numbers.
Understanding Irrational Numbers
Irrational numbers, on the other hand, cannot be expressed as a fraction of two integers. Their decimal representations are non-terminating and non-repeating. This means the digits continue infinitely without ever settling into a repeating pattern. Famous examples include:
-
π (Pi): The ratio of a circle's circumference to its diameter. Its decimal representation is approximately 3.14159, but it continues infinitely without repetition.
-
e (Euler's number): The base of the natural logarithm. It's approximately 2.71828, but its decimal representation is also non-terminating and non-repeating.
-
√2 (Square root of 2): This number, which represents the length of the diagonal of a unit square, cannot be expressed as a simple fraction.
The Square Root of 100: A Rational Number?
Now, let's return to the original question: Is the square root of 100 a rational number? The square root of a number 'x' is a value that, when multiplied by itself, equals 'x'. In this case:
√100 = 10
Since 10 can be expressed as the fraction 10/1 (where both 10 and 1 are integers), the square root of 100 is, indeed, a rational number.
Proof by Definition
The proof is straightforward. We fulfill the definition of a rational number:
- p = 10 (an integer)
- q = 1 (an integer, and not zero)
Therefore, √100 = 10/1, satisfying the criteria for a rational number.
Expanding the Concept: Perfect Squares and Rationality
The square root of 100 is a perfect square – meaning it's the square of an integer (10 x 10 = 100). All perfect squares of integers are inherently rational numbers. This is because they can always be expressed as the ratio of the integer itself and 1.
Exploring Further: Non-Perfect Squares and Irrational Numbers
Consider the square root of 2 (√2). This is not a perfect square and is famously irrational. Attempts to express it as a fraction always fail. The proof of its irrationality is often done through contradiction, assuming it's rational and then showing that leads to a logical impossibility.
The Significance of Rational and Irrational Numbers
The distinction between rational and irrational numbers has profound implications across various branches of mathematics and science:
-
Geometry: Understanding rational and irrational numbers is crucial in geometry, particularly when dealing with lengths and areas, as seen with the diagonal of a square (√2).
-
Calculus: The concept of limits and continuity, fundamental to calculus, heavily relies on the properties of rational and irrational numbers.
-
Computer Science: Representing and manipulating irrational numbers in computer systems presents unique challenges because their decimal representations are infinite.
Conclusion: Rationality and its Implications
The square root of 100 is unequivocally a rational number. This understanding reinforces the fundamental concepts of number theory and its application in various fields. By exploring the nature of rational and irrational numbers, we gain a deeper appreciation for the richness and complexity of the mathematical world. The seemingly simple question of whether the square root of 100 is rational provides a gateway to a deeper understanding of number systems and their properties. This understanding forms a crucial foundation for advanced mathematical concepts and their applications in diverse disciplines. The clear distinction between these number types highlights the precision and structure inherent in mathematics, which allows for consistent and reliable calculations and analysis across various fields of study. Further exploration of this topic opens doors to advanced mathematical concepts and their practical applications in diverse fields.
Latest Posts
Latest Posts
-
Find The Point On The Y Axis Which Is Equidistant From
May 09, 2025
-
Is 3 4 Bigger Than 7 8
May 09, 2025
-
Which Of These Is Not A Prime Number
May 09, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 09, 2025
-
Are Alternate Exterior Angles Always Congruent
May 09, 2025
Related Post
Thank you for visiting our website which covers about Is Square Root Of 100 A Rational Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.