Is The Square Root Of 625 An Irrational Number
News Co
Apr 02, 2025 · 5 min read
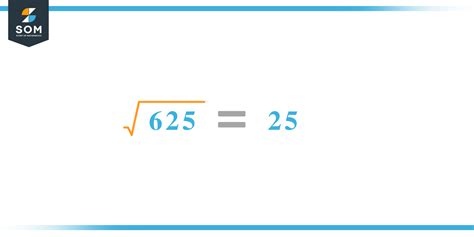
Table of Contents
Is the Square Root of 625 an Irrational Number? A Deep Dive into Rational and Irrational Numbers
The question, "Is the square root of 625 an irrational number?" might seem simple at first glance. However, understanding the answer requires a solid grasp of the fundamental concepts of rational and irrational numbers. This article will not only answer this specific question definitively but also delve into the broader mathematical concepts involved, exploring the properties of rational and irrational numbers and offering practical examples to solidify your understanding.
Understanding Rational Numbers
A rational number is any number that can be expressed as a fraction p/q, where 'p' and 'q' are integers, and 'q' is not equal to zero. This seemingly simple definition encompasses a vast range of numbers. Let's break it down:
- Integers: These are whole numbers, including positive numbers (like 1, 2, 3...), negative numbers (-1, -2, -3...), and zero.
- Fractions: A fraction represents a part of a whole. Rational numbers expressed as fractions can be simplified to their lowest terms. For example, 6/8 simplifies to 3/4.
Examples of Rational Numbers:
- 1/2: A simple fraction representing one-half.
- 3: Can be written as 3/1.
- -2/5: A negative fraction.
- 0: Can be written as 0/1.
- 2.5: Can be written as 5/2. Decimal numbers that terminate (end) or repeat are rational.
Key Characteristics of Rational Numbers:
- Expressible as a fraction: This is the defining characteristic.
- Decimal representation terminates or repeats: When expressed as a decimal, rational numbers either end (like 0.25) or have a repeating pattern (like 0.333...).
Understanding Irrational Numbers
Irrational numbers, on the other hand, cannot be expressed as a fraction of two integers. This seemingly small difference leads to significant mathematical consequences.
Examples of Irrational Numbers:
- π (Pi): The ratio of a circle's circumference to its diameter. Its decimal representation is infinite and non-repeating.
- √2 (Square root of 2): This cannot be expressed as a fraction of two integers. Its decimal representation is infinite and non-repeating (approximately 1.41421356...).
- e (Euler's number): The base of the natural logarithm. Like π, it has an infinite and non-repeating decimal representation.
- √7: The square root of 7 is another classic example of an irrational number.
Key Characteristics of Irrational Numbers:
- Cannot be expressed as a fraction: This is the fundamental distinction.
- Decimal representation is infinite and non-repeating: Their decimal representations go on forever without exhibiting any repeating pattern.
Calculating the Square Root of 625
Now, let's return to the original question: Is the square root of 625 an irrational number? To answer this, we need to find the square root of 625. The square root of a number is a value that, when multiplied by itself, equals the original number. In other words, what number multiplied by itself equals 625?
The answer is 25. Because 25 x 25 = 625.
Is 25 a Rational or Irrational Number?
The number 25 can be expressed as the fraction 25/1. Since it meets the criteria of being expressed as a fraction of two integers, where the denominator is not zero, 25 is a rational number.
Therefore, the square root of 625 is not an irrational number. It is a rational number.
Further Exploring Rational and Irrational Numbers
The distinction between rational and irrational numbers is crucial in various areas of mathematics. Here are some further points to consider:
1. The Real Number System
Rational and irrational numbers together comprise the real number system. This system encompasses all numbers that can be plotted on a number line.
2. Density of Rational and Irrational Numbers
Both rational and irrational numbers are dense. This means that between any two distinct rational numbers, there is always another rational number, and similarly, between any two distinct irrational numbers, there's always another irrational number.
3. Proofs of Irrationality
Proving a number is irrational often involves techniques like proof by contradiction. For instance, the proof that √2 is irrational is a classic example demonstrating the elegance and power of mathematical reasoning. These proofs often leverage the properties of prime numbers and factorization.
4. Decimal Representations and Their Limitations
While decimal representations are useful for understanding numbers, they don't always fully capture the essence of a number's nature. A repeating decimal might seem to suggest a pattern, but it can still represent a rational number. The infinite and non-repeating nature of irrational number decimal representations highlights this limitation.
5. Applications in Real-World Scenarios
Understanding rational and irrational numbers is not just an academic exercise. These concepts are essential in various fields, including:
- Engineering: Precise calculations in engineering often require a deep understanding of rational and irrational numbers. For example, calculating the circumference of a circle involves the irrational number π.
- Physics: Many physical constants are irrational numbers, such as Planck's constant.
- Computer Science: Representing numbers in computers often involves approximations due to the limitations of representing irrational numbers precisely.
- Finance: Calculating compound interest and other financial calculations involve rational numbers.
Conclusion: Rationality and its Significance
In conclusion, the square root of 625 is definitively not an irrational number. It is a rational number, specifically 25. This seemingly simple problem provides a gateway to understanding the profound differences between rational and irrational numbers, their properties, and their significant roles in various fields of study. Understanding these concepts is crucial for developing a strong mathematical foundation and appreciating the elegance and complexity of the number system. The ability to confidently identify and categorize numbers as rational or irrational is a valuable skill for anyone pursuing studies in mathematics, science, or engineering.
Latest Posts
Related Post
Thank you for visiting our website which covers about Is The Square Root Of 625 An Irrational Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.