Least Common Multiple Of 5 And 11
News Co
Apr 05, 2025 · 5 min read
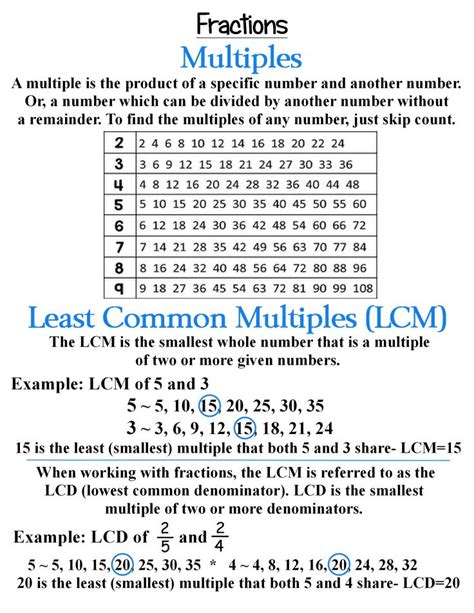
Table of Contents
Unveiling the Least Common Multiple (LCM) of 5 and 11: A Deep Dive
The concept of the Least Common Multiple (LCM) is a fundamental element within number theory and has far-reaching applications in various mathematical fields and practical scenarios. Understanding how to calculate and apply the LCM is crucial for anyone seeking a strong grasp of mathematical principles. This comprehensive article will delve deep into the calculation and significance of the LCM of 5 and 11, exploring different methods and highlighting the broader implications of this seemingly simple concept.
Understanding the Least Common Multiple (LCM)
Before we tackle the specific LCM of 5 and 11, let's establish a solid foundation. The Least Common Multiple, or LCM, of two or more integers is the smallest positive integer that is divisible by all the integers. In simpler terms, it's the smallest number that contains all the given numbers as factors.
For example, let's consider the numbers 2 and 3. The multiples of 2 are 2, 4, 6, 8, 10, 12... and the multiples of 3 are 3, 6, 9, 12, 15... The common multiples are 6, 12, 18... and the least common multiple is 6.
Methods for Calculating the LCM
There are several effective methods for calculating the LCM of two or more numbers. Let's explore the most common ones, applying them to the specific case of 5 and 11:
1. Listing Multiples Method
This is the most straightforward method, particularly useful for smaller numbers. We list the multiples of each number until we find the smallest common multiple.
- Multiples of 5: 5, 10, 15, 20, 25, 30, 35, 40, 45, 50, 55, 60...
- Multiples of 11: 11, 22, 33, 44, 55, 66, 77...
By inspection, we see that the smallest common multiple is 55. Therefore, the LCM(5, 11) = 55.
2. Prime Factorization Method
This method is particularly efficient for larger numbers. It involves finding the prime factorization of each number and then constructing the LCM using the highest powers of each prime factor present.
- Prime factorization of 5: 5 (5 is a prime number)
- Prime factorization of 11: 11 (11 is a prime number)
Since 5 and 11 are both prime numbers and have no common factors other than 1, the LCM is simply the product of the two numbers.
Therefore, LCM(5, 11) = 5 x 11 = 55.
3. Greatest Common Divisor (GCD) Method
This method leverages the relationship between the LCM and the Greatest Common Divisor (GCD) of two numbers. The formula connecting LCM and GCD is:
LCM(a, b) = (|a x b|) / GCD(a, b)
where:
- a and b are the two numbers
- |a x b| represents the absolute value of the product of a and b
- GCD(a, b) is the greatest common divisor of a and b
Let's apply this to 5 and 11:
- The GCD of 5 and 11 is 1 (since they are coprime – they share no common factors other than 1).
- LCM(5, 11) = (5 x 11) / 1 = 55
This method confirms our previous findings.
Significance and Applications of LCM
The concept of the Least Common Multiple extends far beyond simple mathematical exercises. It finds practical application in numerous areas, including:
1. Fraction Arithmetic
LCM plays a crucial role in adding and subtracting fractions with different denominators. To add or subtract fractions, we need to find a common denominator, and the LCM of the denominators is the most efficient choice – the smallest common denominator.
For example, to add 1/5 and 1/11, we find the LCM of 5 and 11 (which is 55), then convert the fractions:
1/5 = 11/55 1/11 = 5/55
Now we can add them: 11/55 + 5/55 = 16/55
2. Scheduling and Timing Problems
LCM is vital in solving problems related to cyclical events. Imagine two machines operating on different cycles. One operates every 5 minutes, and another operates every 11 minutes. To find out when both machines will operate simultaneously, we calculate the LCM(5, 11) = 55. Both machines will operate together every 55 minutes.
3. Modular Arithmetic and Cryptography
The LCM finds application in modular arithmetic, which forms the foundation of many cryptographic systems. The concept of congruences modulo n relies heavily on LCM calculations.
4. Music Theory
In music theory, LCM is used to determine the least common denominator of different rhythmic patterns, helping in the creation of harmonious musical structures.
5. Geometric Problems
Certain geometric problems involving the lengths of sides or the periods of repeating patterns require the use of LCM for their solutions.
Relating LCM to other Mathematical Concepts
The LCM is intrinsically linked to several other key mathematical concepts:
- GCD (Greatest Common Divisor): As demonstrated earlier, the LCM and GCD are inversely related through a simple formula. Understanding one helps in calculating the other.
- Prime Factorization: The prime factorization method provides a systematic approach to calculating the LCM, particularly for larger numbers.
- Divisibility Rules: Understanding divisibility rules can streamline the process of identifying common multiples.
- Modular Arithmetic: LCM is essential in performing calculations within modular arithmetic systems.
Expanding the Concept: LCM of More Than Two Numbers
The methods described above can be extended to find the LCM of more than two numbers. The prime factorization method remains highly efficient in such cases. Simply find the prime factorization of each number and then construct the LCM using the highest powers of each prime factor present across all numbers.
For example, to find the LCM of 5, 11, and 3, we would:
- Find the prime factorization of each number: 5 = 5, 11 = 11, 3 = 3.
- Since there are no common prime factors, the LCM is simply the product of all three numbers: LCM(5, 11, 3) = 5 x 11 x 3 = 165
Conclusion: The Undeniable Importance of LCM
The seemingly simple concept of the Least Common Multiple holds immense significance within mathematics and its diverse applications. Understanding the different methods for calculating the LCM, such as the listing multiples method, prime factorization method, and the GCD method, empowers individuals to tackle various mathematical problems efficiently. From simplifying fraction arithmetic to solving complex scheduling problems and contributing to the foundation of cryptography, the LCM proves its value as a fundamental concept across numerous disciplines. Its interconnectedness with other core mathematical concepts further underscores its importance in building a robust understanding of number theory and its practical applications. Mastering the LCM is a crucial step in developing a stronger mathematical foundation and expanding problem-solving capabilities.
Latest Posts
Related Post
Thank you for visiting our website which covers about Least Common Multiple Of 5 And 11 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.