Number Line Fractions 0 To 1
News Co
Apr 07, 2025 · 5 min read
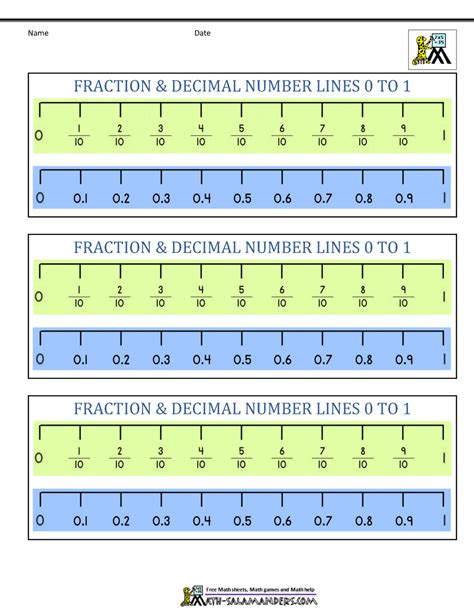
Table of Contents
Number Line Fractions: A Comprehensive Guide from 0 to 1
Understanding fractions is a cornerstone of mathematical literacy. A number line provides a powerful visual tool for grasping the concept of fractions, particularly those between 0 and 1. This comprehensive guide will delve into the intricacies of representing fractions on a number line, exploring various methods, tackling common challenges, and ultimately building a solid foundation for more advanced mathematical concepts.
What is a Number Line?
A number line is a visual representation of numbers, arranged sequentially along a straight line. It provides a clear and intuitive way to compare and order numbers, including fractions. Zero is typically placed in the center, with positive numbers extending to the right and negative numbers extending to the left. For our purposes, we'll focus on the segment of the number line between 0 and 1.
Representing Fractions on the Number Line (0-1)
The number line from 0 to 1 is divided into equal segments, each representing a fraction. The denominator of the fraction dictates the number of segments, while the numerator indicates the number of segments counted from zero.
Simple Fractions: Halves, Thirds, Fourths, and More
Let's start with the simplest fractions:
-
Halves (1/2): The number line is divided into two equal parts. 1/2 is located exactly halfway between 0 and 1.
-
Thirds (1/3, 2/3): The number line is divided into three equal parts. 1/3 is located one-third of the way from 0 to 1, and 2/3 is located two-thirds of the way.
-
Fourths (1/4, 2/4, 3/4): The number line is divided into four equal parts. 1/4, 2/4 (or 1/2), and 3/4 are easily marked.
-
Fifths (1/5, 2/5, 3/5, 4/5): Five equal parts divide the number line, allowing for the precise placement of 1/5, 2/5, 3/5, and 4/5.
This pattern continues for sixths, sevenths, eighths, and any other denominator. The key is to divide the number line into the number of equal parts specified by the denominator.
Visualizing Equivalent Fractions
The number line is incredibly helpful for visualizing equivalent fractions. Equivalent fractions represent the same value, even though they have different numerators and denominators. For example:
- 1/2 = 2/4 = 3/6 = 4/8: If you carefully divide your number line, you'll find that 1/2, 2/4, 3/6, and 4/8 all occupy the exact same point on the line. This visually confirms their equivalence.
This visual representation helps solidify the understanding of equivalent fractions, a crucial concept for simplifying fractions and performing operations like addition and subtraction.
Advanced Fraction Representation: Combining Denominators
Things get more interesting when we need to represent fractions with different denominators on the same number line. This requires finding a common denominator – a number that is a multiple of both denominators.
Example: Representing 1/3 and 1/4 on the same number line.
-
Find the least common multiple (LCM): The LCM of 3 and 4 is 12.
-
Divide the number line into 12 equal parts: This allows us to represent both fractions accurately.
-
Represent 1/3: 1/3 is equivalent to 4/12 (since 1/3 x 4/4 = 4/12). Mark 4/12 on the number line.
-
Represent 1/4: 1/4 is equivalent to 3/12 (since 1/4 x 3/3 = 3/12). Mark 3/12 on the number line.
Now you can visually compare 1/3 and 1/4, clearly demonstrating that 1/3 is greater than 1/4.
Using the Number Line to Compare and Order Fractions
The number line provides an excellent tool for comparing and ordering fractions. Simply plot the fractions on the line; their relative positions indicate their order from least to greatest.
Example: Order the fractions 1/2, 2/5, and 3/4.
-
Find a common denominator (e.g., 20).
-
Convert the fractions to equivalent fractions with the common denominator:
- 1/2 = 10/20
- 2/5 = 8/20
- 3/4 = 15/20
-
Plot these equivalent fractions on a number line divided into 20 equal parts.
-
Observe the order: 8/20 (2/5) < 10/20 (1/2) < 15/20 (3/4).
Addressing Common Challenges and Misconceptions
Several common misconceptions arise when working with fractions on a number line:
-
Unequal divisions: The most critical error is dividing the number line into unequal segments. Each segment must represent the same fraction of the whole.
-
Misinterpreting the numerator and denominator: Students sometimes confuse the roles of the numerator and denominator, leading to incorrect placement of the fraction on the line. Consistent practice reinforces the correct interpretation.
-
Difficulty with larger denominators: Working with fractions having larger denominators can be challenging. Breaking down the task into smaller steps, like finding equivalent fractions with smaller denominators, can help.
Beyond the Basics: Extending the Concepts
The skills developed through working with fractions on a number line form the foundation for more advanced topics:
-
Adding and subtracting fractions: Visualizing fractions on a number line can make adding and subtracting more intuitive. Combine the lengths representing the fractions to find the sum or difference.
-
Decimals and fractions: The number line seamlessly integrates decimals and fractions, allowing for direct comparison and conversion between the two representations.
-
Mixed numbers: Representing mixed numbers (whole numbers and fractions) on a number line extends the concept beyond the 0-1 range.
Practical Applications and Real-World Examples
Understanding fractions is not just an academic exercise; it has numerous practical applications in everyday life:
-
Cooking and baking: Following recipes often requires understanding fractions of cups, teaspoons, and tablespoons.
-
Measurement: Measuring lengths, weights, and volumes invariably involves fractions.
-
Time: Telling time involves fractions of an hour (minutes and seconds).
-
Money: Dealing with cents and dollars is essentially working with fractions of a dollar.
Conclusion: Mastering Fractions with the Number Line
The number line provides a powerful visual aid for understanding and manipulating fractions between 0 and 1. By diligently practicing the techniques outlined in this guide, you will build a strong foundation in fraction comprehension. This solid foundation will significantly aid in tackling more complex mathematical concepts and real-world problem-solving that involve fractions. Remember, the key to success is consistent practice and a clear understanding of the relationship between the numerator, denominator, and the equal divisions on the number line. Through visual representation and careful application, the seemingly abstract world of fractions becomes remarkably accessible and intuitive. The number line serves as a bridge, connecting abstract concepts to tangible, visual understanding.
Latest Posts
Related Post
Thank you for visiting our website which covers about Number Line Fractions 0 To 1 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.