Perimeter And Area Of Triangle Worksheet
News Co
Apr 03, 2025 · 7 min read
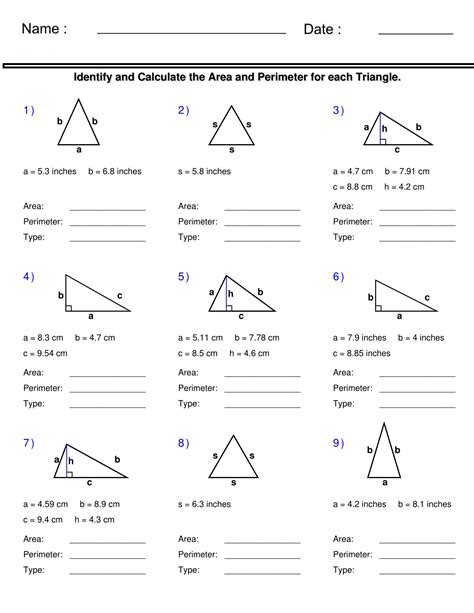
Table of Contents
Perimeter and Area of a Triangle Worksheet: A Comprehensive Guide
This comprehensive guide delves into the calculation of the perimeter and area of triangles, providing you with a complete worksheet, explanations, examples, and practice problems to solidify your understanding. We'll explore various triangle types and the formulas needed for accurate calculations. This resource is designed to be useful for students, educators, and anyone looking to brush up on their geometry skills.
Understanding Triangles: Types and Properties
Before diving into calculations, let's review the different types of triangles and their key properties:
Types of Triangles:
- Equilateral Triangle: All three sides are equal in length, and all three angles are equal (60° each).
- Isosceles Triangle: Two sides are equal in length, and the angles opposite these sides are also equal.
- Scalene Triangle: All three sides have different lengths, and all three angles are different.
- Right-Angled Triangle: One angle is a right angle (90°). The side opposite the right angle is called the hypotenuse, and the other two sides are called legs or cathetus.
- Obtuse Triangle: One angle is greater than 90°.
- Acute Triangle: All three angles are less than 90°.
Key Triangle Properties:
- The sum of the angles in any triangle always equals 180°. This is a fundamental property used in various triangle calculations.
- The longest side of a triangle is always opposite the largest angle. Conversely, the shortest side is opposite the smallest angle.
Calculating the Perimeter of a Triangle
The perimeter of any polygon (a closed figure with straight sides) is simply the sum of the lengths of its sides. For a triangle, this means:
Perimeter = Side a + Side b + Side c
Where 'a', 'b', and 'c' represent the lengths of the three sides of the triangle.
Example:
A triangle has sides of length 5 cm, 7 cm, and 9 cm. Its perimeter is:
Perimeter = 5 cm + 7 cm + 9 cm = 21 cm
Calculating the Area of a Triangle
The area of a triangle is calculated differently depending on the information available. Here are the most common methods:
1. Using Base and Height:
This is the most fundamental method for calculating the area of a triangle.
Area = (1/2) * base * height
Where:
- base: The length of any side of the triangle.
- height: The perpendicular distance from the base to the opposite vertex (the highest point).
Example:
A triangle has a base of 10 cm and a height of 6 cm. Its area is:
Area = (1/2) * 10 cm * 6 cm = 30 cm²
2. Using Heron's Formula:
Heron's formula is particularly useful when you know the lengths of all three sides but not the height.
Area = √[s(s-a)(s-b)(s-c)]
Where:
- a, b, c: The lengths of the three sides of the triangle.
- s: The semi-perimeter of the triangle, calculated as s = (a + b + c) / 2
Example:
A triangle has sides of length 5 cm, 6 cm, and 7 cm.
- Calculate the semi-perimeter (s): s = (5 + 6 + 7) / 2 = 9 cm
- Apply Heron's formula: Area = √[9(9-5)(9-6)(9-7)] = √[9 * 4 * 3 * 2] = √216 ≈ 14.7 cm²
3. Using Trigonometry (for right-angled triangles):
For right-angled triangles, you can use trigonometric functions if you know the lengths of two sides.
- Area = (1/2) * a * b (where 'a' and 'b' are the lengths of the two legs/cathetus)
- Area = (1/2) * a * b * sin(C) (where 'a' and 'b' are the lengths of two sides, and C is the angle between them)
Example:
A right-angled triangle has legs of length 4 cm and 3 cm. Its area is:
Area = (1/2) * 4 cm * 3 cm = 6 cm²
Perimeter and Area of Triangle Worksheet: Practice Problems
Now, let's put your knowledge to the test with some practice problems:
Section 1: Perimeter
- Find the perimeter of an equilateral triangle with side length 8 cm.
- A triangle has sides of length 12 cm, 15 cm, and 20 cm. Calculate its perimeter.
- An isosceles triangle has two equal sides of 10 cm each, and the third side is 16 cm. What is its perimeter?
Section 2: Area (using base and height)
- A triangle has a base of 14 cm and a height of 5 cm. Calculate its area.
- The base of a triangle is 22 cm, and its height is 8 cm. Find the area.
- A right-angled triangle has a base of 9 cm and a height of 12 cm. What is its area?
Section 3: Area (using Heron's formula)
- Calculate the area of a triangle with sides of length 7 cm, 8 cm, and 9 cm.
- A triangle has sides of length 10 cm, 13 cm, and 15 cm. Find its area using Heron's formula.
- Find the area of a triangle with sides of 11cm, 14cm, and 17cm.
Section 4: Area (using trigonometry – for right-angled triangles only)
- A right-angled triangle has a hypotenuse of 13 cm and one leg of 5 cm. Calculate its area. (Hint: Use the Pythagorean theorem to find the other leg first).
- A right-angled triangle has legs of 6cm and 8cm. Calculate the area.
- A right angled triangle has one leg of 12cm and the hypotenuse of 13cm. Find the area of the triangle.
Section 5: Mixed Problems
- A triangle has a perimeter of 36 cm. Two of its sides are 10 cm and 12 cm. What is the length of the third side?
- A triangle has an area of 48 cm² and a base of 16 cm. What is its height?
- An equilateral triangle has an area of 16√3 cm². What is the length of its sides?
Answer Key (Provided at the end of the blog post): (Note: Answers are placed at the very end to encourage independent problem-solving)
Advanced Concepts: Beyond the Basics
This section explores more advanced concepts related to triangles, including:
1. Similar Triangles:
Similar triangles have the same shape but different sizes. Their corresponding angles are equal, and their corresponding sides are proportional. The ratio of corresponding sides is called the scale factor. Areas of similar triangles are proportional to the square of the scale factor.
2. Congruent Triangles:
Congruent triangles have the same size and shape. Their corresponding angles and sides are equal.
3. Area of Triangles in Coordinate Geometry:
If the coordinates of the vertices of a triangle are known, the area can be calculated using the determinant method.
4. Applications of Triangles:
Triangles have numerous applications in various fields, including engineering, architecture, surveying, and computer graphics.
Conclusion
Understanding the perimeter and area of triangles is a fundamental skill in geometry with broad applications. This comprehensive guide and worksheet provide a solid foundation for mastering these calculations. Practice consistently, and you will develop confidence and proficiency in solving a wide range of triangle problems. Remember to always double-check your calculations and choose the appropriate formula based on the given information.
(Answer Key to Practice Problems):
(Note: Due to formatting limitations, a detailed step-by-step solution for each problem is not included here. However, working through the problems using the formulas provided earlier should allow you to obtain the correct answers.)
Section 1: Perimeter
- 24 cm
- 47 cm
- 36 cm
Section 2: Area (using base and height)
- 35 cm²
- 88 cm²
- 54 cm²
Section 3: Area (using Heron's formula)
- ≈26.83 cm²
- ≈60 cm²
- ≈73.5 cm²
Section 4: Area (using trigonometry)
- 30 cm²
- 24 cm²
- 60 cm²
Section 5: Mixed Problems
- 14 cm
- 6 cm
- 4√3 cm
This detailed answer key, combined with the practice problems and comprehensive explanations, makes this a highly effective resource for learning about the perimeter and area of triangles. Remember to utilize different problem-solving approaches to enhance your understanding.
Latest Posts
Related Post
Thank you for visiting our website which covers about Perimeter And Area Of Triangle Worksheet . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.